If each child is given 10 sweets, there are 3 sweets left over. But if each is given 11, then the number of sweets is 4 less. Find the number of sweets. (a) 37 (b) 57 (c) 73 (d) 75
To solve the problem, let's denote the number of sweets as S and the number of children as x.
Step 1: Set up the equations based on the given conditions.
From the first condition:
- If each child is given 10 sweets, there are 3 sweets left over. This can be expressed as:
S=10x+3 (Equation 1)
From the second condition:
- If each child is given 11 sweets, then there are 4 sweets less than the total number of sweets. This can be expressed as:
S=11x−4 (Equation 2)
Step 2: Set the two equations equal to each other.
Since both equations equal S, we can set them equal to each other:
10x+3=11x−4
Step 3: Solve for x.
Rearranging the equation:
10x+3+4=11x
7=11x−10x
7=x
Step 4: Substitute x back into one of the equations to find S.
Using Equation 1:
S=10x+3
Substituting x=7:
S=10(7)+3
S=70+3
S=73
Conclusion
The number of sweets is S=73.
Answer
(c) 73
---
- If each child is given 10 sweets, there are 3 sweets left over. This can be expressed as:
S=10x+3 (Equation 1)
- If each child is given 11 sweets, then there are 4 sweets less than the total number of sweets. This can be expressed as:
S=11x−4 (Equation 2)
10x+3=11x−4
10x+3+4=11x
7=11x−10x
7=x
S=10x+3
Substituting x=7:
S=10(7)+3
S=70+3
S=73
(c) 73
Similar Questions
If each child is given 10 sweets, there are 3 sweets left over. But if each is given 11, then the number of sweets is 4 less. Find the number of sweets. (a) 37 (b) 57 (c) 73 (d) 75
View SolutionThe following frequency table shows the demand for a sweet and the number of customers. Find the mode of demand of sweet.

View Solution
Knowledge Check
A total of 57 sweets were distributed among 10 children such that each girl gets 6 sweets and each boy gets 5 sweets. The number of boys is :
A6B4C3D5
The following frequency table shows the demand for a sweet and the number of customers. Find the mode of the demand of sweet.
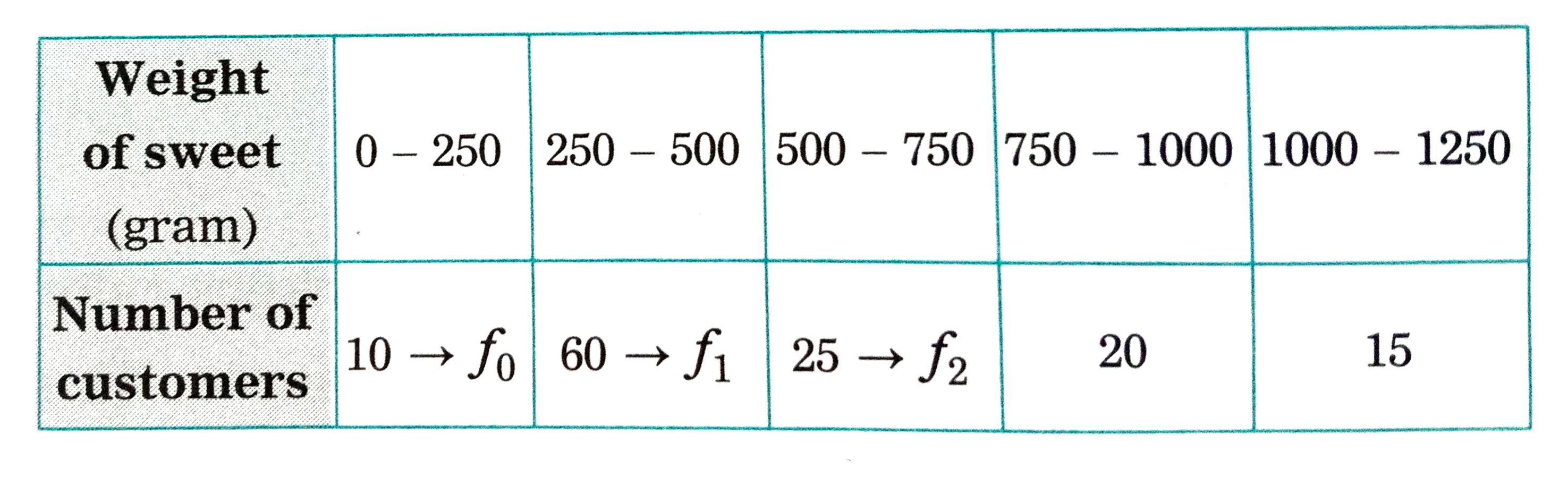
View SolutionThe following frequency table shows the demand for a sweet and the number of customers. Find the mode of the demand of sweet.

View SolutionTwo numbers are in the ratio 7:11. If 7 is added to each of the numbers, the ratio becomes 2:3. Find the numbers. (a) 39 (b) 49 (c) 66 (d) 77
View SolutionIf 2 is added to each of two given numbers, their ratio becomes 1 : 2. However, if 4 is subtracted from each of the given numbers, the ratio becomes 5 : 11. Find the numbers.
View SolutionThe ratio of two numbers is 5:7.3 of each post deducted If the new ratio is 2:3 then find the numbers.
View SolutionIn a group of children, each child exchanges a gift with every other child. If the number of gifts is 132, then the number of children in the group is (a) 10 (b) 11 (c) 12 (d) 13
View SolutionThe ratio between two numbers is 3:4 . If 6 is added in each , the new numbers are in the ration 4:5 find the given numbers .
View Solution
