The equation of a projectile is y = ax - bx2. Its horizontal range is
ab
ba
a + b
b - a
The correct Answer is:A
To find the horizontal range of a projectile given the equation of its trajectory y=ax−bx2, we can follow these steps:
1. Identify the Equation of the Projectile:
The equation of the projectile is given as:
y=ax−bx2
This represents a parabolic trajectory.
2. Understand the Structure of the Equation:
The equation can be rewritten in the standard form of a parabola:
y=ax−bx2
Here, a represents the slope of the trajectory at the origin, and b is related to the curvature of the parabola.
3. Determine the Vertex of the Parabola:
The vertex of the parabola can be found using the formula for the x-coordinate of the vertex:
xv=−B2A=−a2(−b)=a2b
where A=−b and B=a.
4. Calculate the Maximum Height:
Substitute xv back into the original equation to find the maximum height yv:
yv=a(a2b)−b(a2b)2
Simplifying this will give the maximum height, but we are primarily interested in the range.
5. Find the Range:
The range R of the projectile can be determined by finding the x-intercepts of the trajectory (where y=0):
0=ax−bx2
Factoring gives:
x(ax−b)=0
This gives two solutions: x=0 (the launch point) and x=ab (the range).
6. Final Expression for the Range:
Thus, the horizontal range R is:
R=ab
Conclusion:
The horizontal range of the projectile is given by:
ab
The equation of the projectile is given as:
y=ax−bx2
This represents a parabolic trajectory.
The equation can be rewritten in the standard form of a parabola:
y=ax−bx2
Here, a represents the slope of the trajectory at the origin, and b is related to the curvature of the parabola.
The vertex of the parabola can be found using the formula for the x-coordinate of the vertex:
xv=−B2A=−a2(−b)=a2b
where A=−b and B=a.
Substitute xv back into the original equation to find the maximum height yv:
yv=a(a2b)−b(a2b)2
Simplifying this will give the maximum height, but we are primarily interested in the range.
The range R of the projectile can be determined by finding the x-intercepts of the trajectory (where y=0):
0=ax−bx2
Factoring gives:
x(ax−b)=0
This gives two solutions: x=0 (the launch point) and x=ab (the range).
Thus, the horizontal range R is:
R=ab
The horizontal range of the projectile is given by:
ab
Similar Questions
Knowledge Check
The equation of projectile is y=16x−x24 the horizontal range is:-
A16mB8mC64mD12.8mThe equation of a projectile is y=√3x−(gx22) the horizontal range is
A2g√3B2√3gCg2√3D√3g2The equation of motion of a projectile is y=12x−34x2. The horizontal component of velocity is 3ms−1. What is the range of the projectile ?
A18 mB16 mC12 mD21.6 m
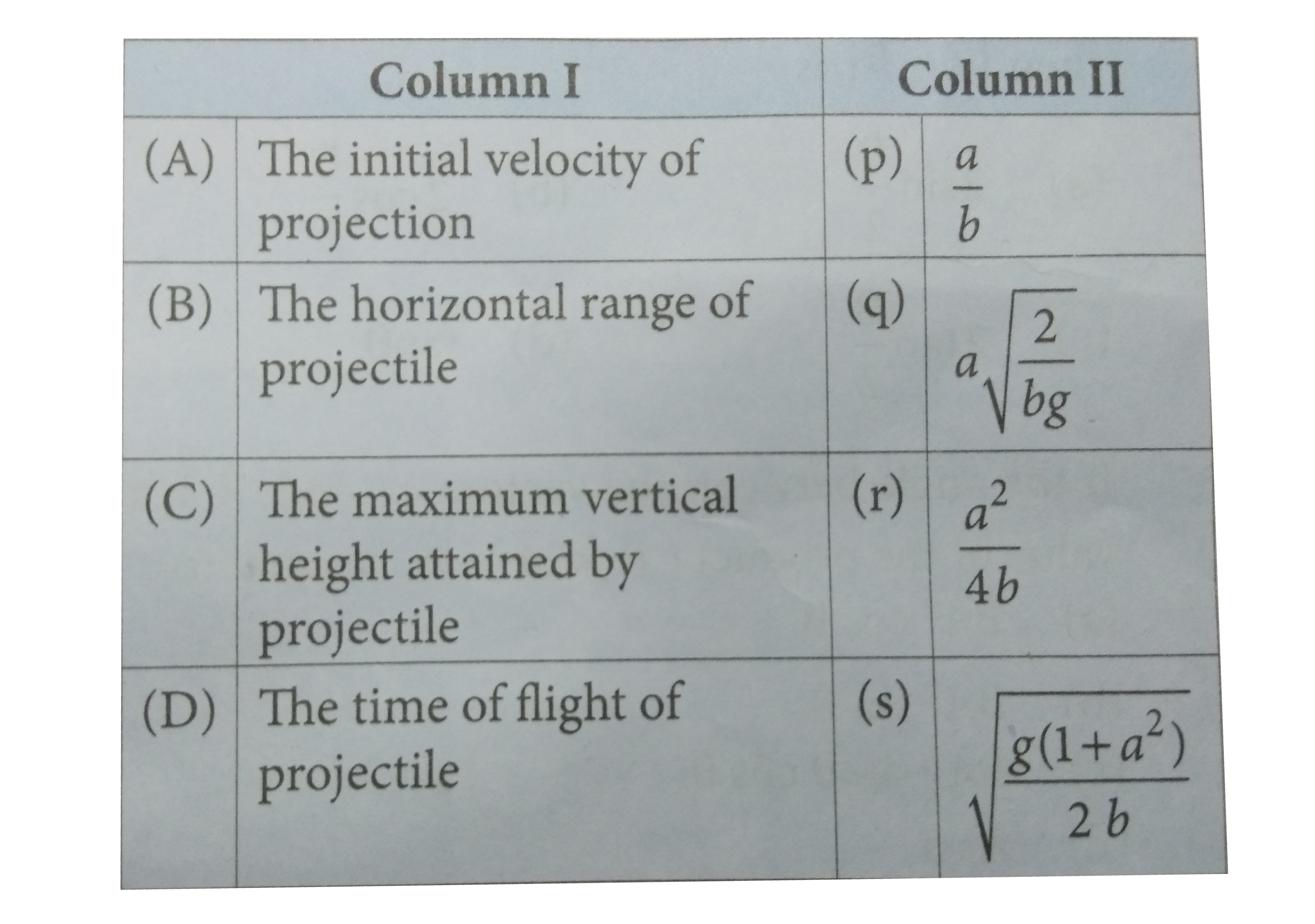
The equation of motion of a projectile is y=ax−bx2, where a and b are constants of motion. Match the quantities in Column I with the relations in Column II.
View SolutionThe time of flight of a projectile is related to its horizontal range by the equation gT2=2R.The angle of projection is?
View Solution[" The equation of trajectory of a projectile is "],[y=ax-bx^(2)" where "x" and "y" axes are along "],[" horizontal and vertical directions "],[" respectively.The range of the projectile is "]
View SolutionThe range of a projectile launched at an angle of 15∘ with horizontal is 1.5km. The range of projectile when launched at an angle of 45∘ to the horizontal is
View SolutionA particle is projected from the ground . Point of projection is taken as origin, horizontal direction as X-axis and verticle upward direction ass Y-axis. Equation of trajectory of the partcle is y=αx−βx2. Horizontal range of the projectile is
View Solution
