In a triangle P Q R ,Sa n dT are points on Q Ra n dP R , respectively,

In a triangle $P Q R, S a n d T$ are points on $Q R a n d P R,$ respectively, such that $Q S = 3 S R a n d P T = 4 T R .$ Let $M$ be the point of intersection of $P S a n d Q T .$ Determine the ratio $Q M : M T$ using the vector method .

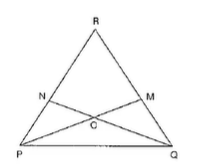
View Solution

In Figure, $∠ Q P R = ∠ P Q R$ and $M$ and $N$ are respectively on sides $Q R$ and $P R$ of $P Q R$ such that $Q M = P N$ . Prove that $O P = O Q,$ where $O$ is the point of intersection of $P M$ and $Q N .$
ocr_image

View Solution

In $P Q R$ , $M$ and $N$ are points on sides $P Q$ and $P R$ respectively such that $P M = 15 c m$ and $N R = 8 c m$ . If $P Q = 25 c m$ and $P R = 20 c m$ state whether $M N Q R$ .

View Solution

In Figure, $∠ Q P R = ∠ P Q R a n d M a n d N$ are respectively on sides $Q R a n d P R$ of $P Q R$ such that $Q M = P N .$ Prove that $O P = O Q,$ where $O$ is the point of intersection of $P M a n d Q N$
ocr_image

View Solution

In Fig. 4.238, $S$ and $T$ are points on the sides $P Q$ and $P R$ respectively of $P Q R$ such that $P T = 2 c m$ , $T R = 4 c m$ and $S T$ is parallel to $Q R$ . Find the ratio of the areas of $P S T$ and $P Q R$ . (FIGURE)

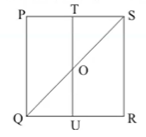
View Solution

In Figure, $P Q R S$ is a square and $T a n d U$ are, respectively, the mid-points of $P S a n d Q R$ . Find the area of $O T S$ if $P Q = 8 c m .$
ocr_image

View Solution

In a triangle, $P, Q a n d R$ are the mid-points of sides $B C, C A a n d A B$ respectively. If $A C = 21 c m, B C = 29 c m a n d A B = 30 c m,$ find the perimeter of the quadrilateral $A R P Q$

View Solution

In $Δ P Q R,$ if $P Q = Q R$ and $L, M$ and $N$ are the mid-points of the sides $P Q, Q R$ and $R P$ respectively. Prove that $L N = M N .$
ocr_image

View Solution

$P Q R S$ is a square such that $P R a n d S Q$ intersect at $O .$ State the measure of $∠ P O Q$

View Solution

$O P Q R$ is a square and $M, N$ are the middle points of the sides $P Q a n d Q R$ , respectively. Then the ratio of the area of the square to that of triangle $O M N$ is 4:1 (b) 2:1 (c) 8:3 (d) 7:3

View Solution

In a triangle $P Q R, S a n d T$ are points on $Q R a n d P R,$ respectively, such that $Q S = 3 S R a n d P T = 4 T R .$ Let $M$ be the point of intersection of $P S a n d Q T .$ Determine the ratio $Q M : M T$ using the vector method .

Similar Questions

Recommended Questions

In a triangle PQR,SandT are points on QRandPR, respectively, such that QS=3SRandPT=4TR. Let M be the point of intersection of PSandQT. Determine the ratio QM:MT using the vector method .

Similar Questions

Recommended Questions

In a triangle $P Q R, S a n d T$ are points on $Q R a n d P R,$ respectively, such that $Q S = 3 S R a n d P T = 4 T R .$ Let $M$ be the point of intersection of $P S a n d Q T .$ Determine the ratio $Q M : M T$ using the vector method .