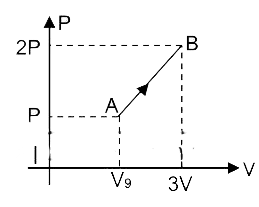
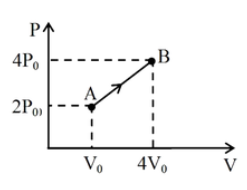
One mole of a monatomic ideal gas undergoes the process A $\to$ B in the given p-V diagram. Specific heat capacity in the process is

A

$\frac{13 R}{3}$

B

$\frac{13 R}{6}$

C

$\frac{7 R}{3}$

D

$\frac{2 R}{3}$

Text Solution

Verified by Experts

The correct Answer is:B

According to the question,

Now, the termperature at point A and B By, pV = nRT (from ideal gas equation )
Temperature at point A,
$T_{A} = \frac{3 p_{0} V_{0}}{R}$
Temperature at point B,
$T_{B} = \frac{30 p_{0} V_{0}}{R}$
So, the termperature difference, $Δ T - T_{B} - T_{A}$
$∴ Δ T = \frac{30 p_{0} V_{0}}{R} - \frac{3 p_{0} V_{0}}{R}$
Or, $Δ T = \frac{27 p_{0} V_{0}}{R}$
Now, Due to the adiabatic processs, change is internal energy,
$∴ Δ U n C_{v} Δ$ T
or , $Δ U = \frac{3}{2} R Δ T$ ....(i)
Work done by the gas undergoes the process
A $\to$ B
$∴$ W = Area under p - V graph
or $W = 18 p_{0} V_{0}$
or $W = \frac{2}{3} R Δ T$ ... (ii)
Now, by first law of thermodynamics,
$Q = Δ U$ + W
By putting the values from Eqs. (i) and (ii) to above Eq. we get
$∴ Q = \frac{3}{2} R Δ T + \frac{2}{3} R Δ T$
or C $Δ T = \frac{13}{6} R Δ T$
$(∵ Q = nC Δ T, where n = 1 mole)$
or $C = \frac{13}{6} R$
So, the specific heat capacity in the process A $\to$ B is C = $\frac{13}{6}$ R .

|

Updated on:21/07/2023

Class 12 PHYSICS QUESTION PAPER 2019

Similar Questions

Knowledge Check

Question 1 - Select One
One mole of a monoatomic ideal gas undergoes process AB in given P – V diagram then average specific heat for this process is:

A $\frac{21 R}{10}$
B $\frac{18 R}{10}$
C $\frac{9 R}{10}$
D $\frac{13 R}{10}$
Question 2 - Select One
One mole of a monoatomic gas undergoes a linear process $A \to B$ in $P - v$ diagram. The volume of the gas when the process turns from an endothermic to an exothermic one is

A $\frac{5}{8} v_{0}$
B $\frac{v_{0}}{2}$
C $\frac{8 v_{0}}{15}$
D $\frac{3 v_{0}}{5}$
Question 3 - Select One
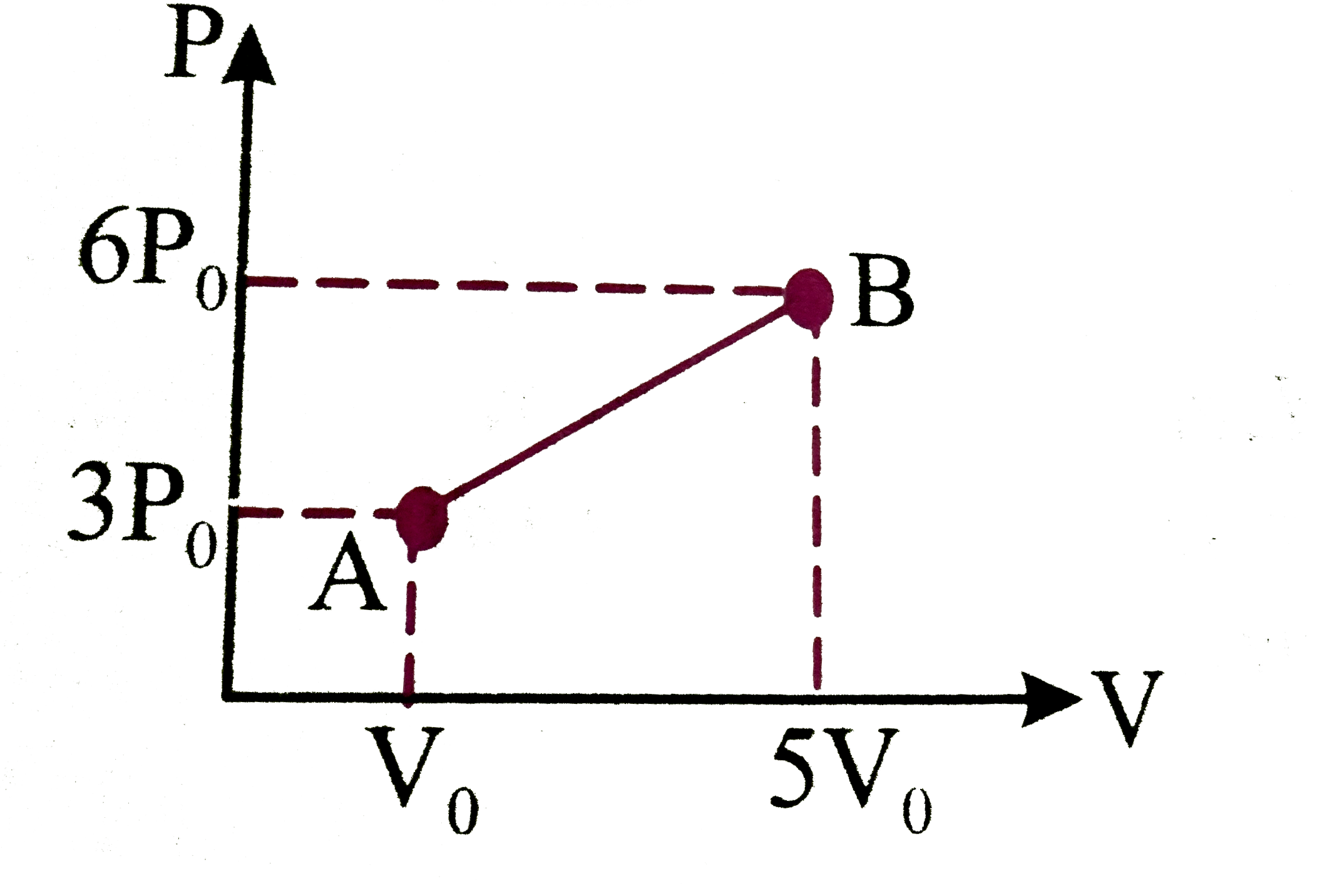
One mole of an ideal monoatomic gas undergoes a process as shown in the figure. Find the molar specific heat of the gas in the process.

R is a gas constant.
A $\frac{3 R}{4}$
B $\frac{R}{2}$
C $\frac{R}{3}$
D $\frac{2 R}{3}$

Recommended Questions

Home
Ask Doubt
Profile