Lloyds mirror experiment
Step by step video solution for Lloyds mirror experiment by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
In Lloyd's mirror experiment (Fig.) a light wave emitted directly be the source S (narrow slit) interferes with the wave reflected form a mirror M. As s result, an interference fringe pattern is

formed on the screen Sc. the source and the mirror are separated by a distance l=100cm.At a certain position of the source the fringe width on the screen was equal to Δx=0.25mm, and after source was moved away from the mirror plane by Δh=0.60mm, the fringe width decreased η=1.5 times. Find the wavelength of light.
View SolutionIn Lloyd's mirror interference experiment the source slit is at a distance of 2 mm from a plane mirror . The interference fringes , observed on a screen at a distance of 1.5 m from the slit , have a separation of 0.221 mm . Calculate the wavelength of light used .
View Solution
Knowledge Check
In a Lloyds's mirror experiment as narraow slit S iransmitting a light of wavelength λ is placed 3 mm above a small plane mirror ( as shown). The light coming directly from the the slit and that coming after the reflection interfere on a screen placed at a distance of 90 cm from the slit. Length of mirror is 2 mm and the middle point of mirror is 2 mm from point P.
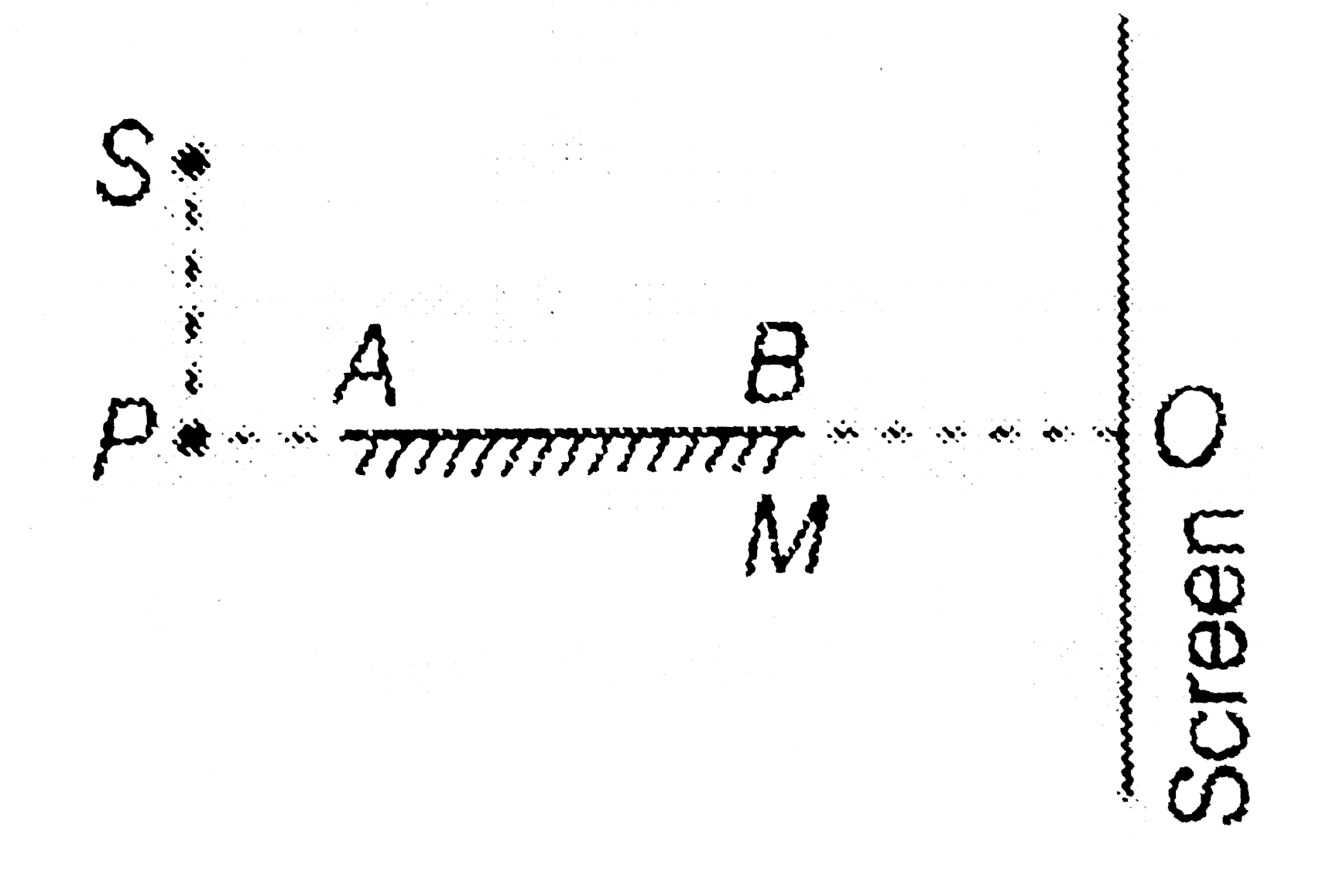
If the mirror is shifted towards left then how does the fringe pattern on screen changes ?Afringe width decreases and the region in which interference is formed shifts downwardsBfringe width decreases and the region in which interference is formed shifts upwardsCfringe width does not change and the region in which interference is formed shifts upwardsDfringe width does not change and the region in which interference is formed shifts downwardsIn a Lloyds's mirror experiment as narraow slit S iransmitting a light of wavelength λ is placed 3 mm above a small plane mirror ( as shown). The light coming directly from the the slit and that coming after the reflection interfere on a screen placed at a distance of 90 cm from the slit. Length of mirror is 2 mm and the middle point of mirror is 2 mm from point P.

If fringe width is 0.1 mm then what is the wavelength of light used ?A3.33×10−7mB6.67×10−7mC1.0×10−7mD4×10sWhen a light wave passes from a rarer medium to a denser medium, there will be a phase change of π radians. This difference brings change in the conditions for constructive and destructive interference. This phenomena also reasons the fromation of interference pattern in thin films like, oily layer, soap film, etc., but has no reason on the shifting of fringes from the central portion outward. The shift is dependent on the refractive index of the material as per the relation, Δy=(μ−1)t
The condition for constructive interference in Lloyd's single mirror experiment is the path difference which is equal toANλB(2N−1)λ2C(N−1)λ2Dλ2(2N−1)
A Lloyd's mirror or length 5 cm is illuminated with mon ochromatic light of wavelength λ(=6000Å) from a narrow 1 mm slit in its plane and 5 cm plane from its near edge. Find the fringe width on a screen 120 cm from the slit and width of interference pattern on the screen.

View SolutionIn Lloyd's experiment , the slit and its image have a separation of 4.32 mm and the obsevations made at a plane 2.0 m away fringes of separation 0.26 mm. Find the wavelength of light used.
View SolutionIn a Lloyd's experiment , the path difference between the direct and reflected beam of light for the 8th maxima fringe was observed to be 0.23 mm . Find the wavelength of light used .
View SolutionIn a concave mirror experiment, an object is placed. at a distance x1 from the focus and the image is formed at a distance x2 , from the focus. The focal length of the mirror would be
View SolutionThe arrangement for a mirror experiment is shown in figure. S is a point source of frequency 6×1014Hz. D and C represent the two ends of a mirror placed horizontally and LOM represents the screen.

Calculate the number of fringes
View Solution
