A uniform narrow rod of mass M and length 2 L is kept vertically, along the y-axis (as shown in Fig. ) on a smooth horizontal plane. The lower end of the rod coincides with the origin (0,0). Due to a slight disturbance at time t = 0 , the rod slides along the positive x-axis and begins to fall. Determine (i) the shift in the centre of gravity during the fall,
(ii) the equation of the locus of a point at a distance r from the lower end of the rod and also mention the shape of the locus.
Initially (0,L) was the coordinates of the centre of gravity of the rod. Let B be the point at a distance r, from point O (0,0) , on the rod. So, (0,r) denotes the point B. At t=0, the rod is disturbed and it falls. During this fall there is no other force acting on it except the downward gravitational force.
(i) We know that the centre of gravity is influenced only by an external force which is gravity in this case. So the centre of gravity will be shifted vertically downwards from (0,L) ot (0,0).
(ii) Let (x,y) be the position of B. at any moment during the fall of the rod, where B. is the point at a distance r, from the lower end O. of the rod.
From the Fig. OG.O.G.=CB.O.B.or,aL=yr
Again, O.OOC=O.GG.B.or,OCG.B.=O.OO.G.or,xr−L=bL
∴x2(r−L)2=b2L2=L2−a2L2=1−a2L2=1−y2r2
or, x2(r−L)2+y2r2=1
This is the equation of the locus of a point (x,y) and it is elliptical with its centre at the origin.

Topper's Solved these Questions
- STATICSBOOK - CHHAYA PUBLICATIONCHAPTER - STATICSEXERCISE - HIGHER ORDER THINKING SKILL (HOTS) QUESTIONS
18 Videos
- STATICSBOOK - CHHAYA PUBLICATIONCHAPTER - STATICSEXERCISE - EXERCISE (Multiple Choice Questions )
14 Videos
- SIMPLE HARMONIC MOTIONBOOK - CHHAYA PUBLICATIONCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - CBSE SCANNER
16 Videos
- SUPERPOSITION OF WAVESBOOK - CHHAYA PUBLICATIONCHAPTER - SUPERPOSITION OF WAVESEXERCISE - CBSE Scanner
14 Videos
Similar Questions
A uniform thin rod of mass m and length L is standing vertically along the y-axis on a smooth horizontal surface, with its lower end at the origin (0,0). A slight disturbane at t = 0 causes the lower end t slip on the smooth surface along the positive x-axis, and the rod starts falling.
(i) What is the path followed by the centr of mass of the rod during its fall?
(ii) Find the equaiton to the trajectory of a point on the rod shape of the path of this point?
View SolutionA uniform thin rod of mass m and length L is standing vertically along the y-axis on a smooth horizontal surface, with its lower end at the origin (0,0). A slight disturbane at t = 0 causes the lower end t slip on the smooth surface along the positive x-axis, and the rod starts falling.
(i) What is the path followed by the centr of mass of the rod during its fall?
(ii) Find the equaiton to the trajectory of a point on the rod shape of the path of this point?
View Solution
Knowledge Check
Three thin uniform rods each of mass M and length L and placed along the three axis of a Cartesian coordinate system with one end of each rod at the origin. The M.I. of the system about z-axis is
AML23B2ML23CML26DML2
A rod of length l is released from the vertical position on a smooth horizontal plane. What is the nature of motion of the free end of the rod ?
View SolutionA uniform rod of mass M and length L is standing vertically along the y-axis on a smooth horizontal surface as shown. A small disturbance causes the lower end to slip on the smooth horizontal surface and rod starts falling. The path followed by centre of mass of the rod during the fall is
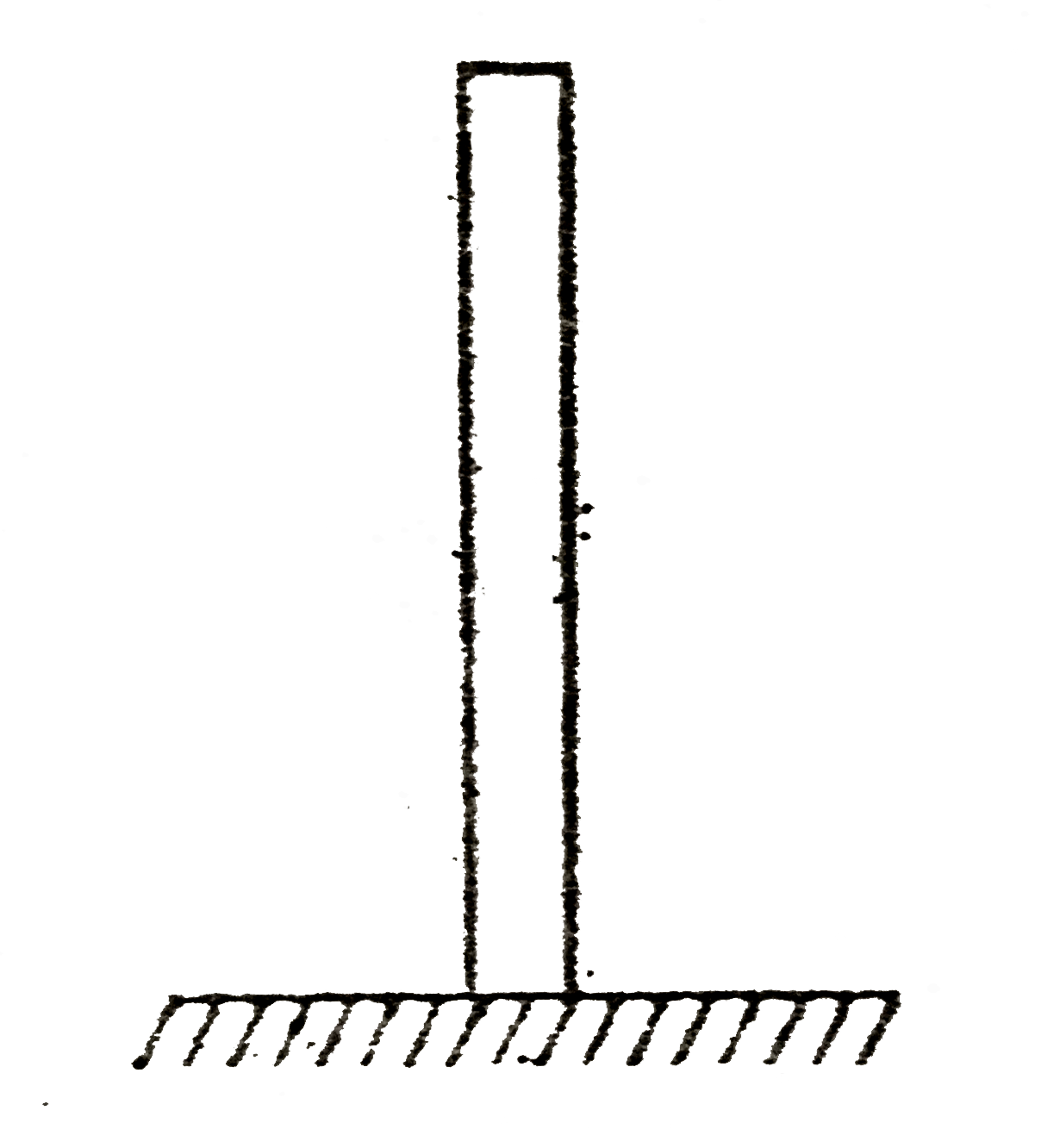
View SolutionA uniform rod of mass M and length L is standing vertically along the y-axis on a smooth horizontal surface as shown. A small disturbance causes the lower end to slip on the smooth horizontal surface and rod starts falling. The path followed by centre of mass of the rod during the fall is

View SolutionA uniform rod of length l and mass m is free to rotate in a vertical plane about A as shown in Fig. The rod initially in horizontal position is released. The initial angular acceleration of the rod is

View SolutionA uniform rod of mass m and length L is free to rotate in the vertical plane about a horizontal axis passing through its end. The rod initially in horizontal position is released. The initial angular acceleration of the rod is:

View SolutionA uniform rod of length l is kept vertically on a rough horizontal surface at x = 0 . It is rotated slightly and released . When the rod finally falls on the horizontal surface , the lower end will remain at
View SolutionA uniform rod of length l is kept vertically on a rough horizontal surface at x = 0 . It is rotated slightly and released . When the rod finally falls on the horizontal surface , the lower end will remain at

View Solution
