Three identical square plates rotate about the axes shown in the. In such a way that their kinetic energies are equal. Each of the rotation axes passes through the centre of the square. Then the ratio of angular speeds ω1:ω2:ω3 is

1:1:1
√2:√2:1
1:√2:1
1:2:√2
The correct Answer is:B
Kinetic energy , K = 12Iω2
According to the question, K = constant
∴ ω∝1√I
I1=I2=112l2m
[ l = length of the side of square and m = mass of the square plate]
and I3=16l2m
∴ ω1:ω2ω3=1√I1:1√I2:1√I3
= √12:√12:√6=√2:√2:1
Topper's Solved these Questions
- ROTATION OF RIGID BODIES Book:CHHAYA PUBLICATIONChapter:ROTATION OF RIGID BODIES Exercise:EXAMINATION ARCHIVE with solutions (JEE Main )
Explore 10 Videos
- ROTATION OF RIGID BODIES Book:CHHAYA PUBLICATIONChapter:ROTATION OF RIGID BODIES Exercise:EXAMINATION ARCHIVE with solutions (AIPMT )
Explore 3 Videos
- ROTATION OF RIGID BODIES Book:CHHAYA PUBLICATIONChapter:ROTATION OF RIGID BODIES Exercise:EXAMINATION ARCHIVE with solutions (WBCHSE )
Explore 12 Videos
- QUESTION PAPERS OF WBCHSE -2018Book:CHHAYA PUBLICATIONChapter:QUESTION PAPERS OF WBCHSE -2018Exercise:SECTION -II
Explore 20 Videos
- SIMPLE HARMONIC MOTIONBook:CHHAYA PUBLICATIONChapter:SIMPLE HARMONIC MOTIONExercise:CBSE SCANNER
Explore 16 Videos
Similar Questions
A solid square plate is spun around different axes with the same angular speed. In which of the following choice of axis of rotation will the kinetic energy of the plate be the largest?
View SolutionA solid sphere of radius r and mass m rotates about an axis passing through its centre with angular velocity ω. Its K.E. Is
View Solution
Knowledge Check
Three identical square plates rotate about the axes shown in the figure in such a way that their kinetic energies are equal. Each of the rotation axes passes through the centre of the square. Then the ratio of angular speeds ω1:ω2:ω3 is
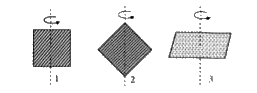 A1:1:1B√2:√2:1C1:√2:1D1:2:√2
A1:1:1B√2:√2:1C1:√2:1D1:2:√2A solid cylinder of mass M and radius R rotates about its axis with angular speed ω. Its rotational kinetic energy is
A12MR2ω2BMR2ω2C14MR2ω2D18MR2ω2A solid cylinder of mass M and radius R rotates about its axis with angular speed ω. Its rotational kinetic energy is
A12MR2ω2BMR2ω2C14MR2ω2D18MR2ω2
A solid sphere of radius r and mass m rotates about an axis passing through its centre with angular velocity ω. Its K.E. Is
View SolutionTwo discs of moments of inertia I1andI2 about their respective axes (normal to the disc and passing through the centre), and rotating with angular speed ω1andω2 are brought into contact face to face with their axes of rotation coincident . What is the angular speed of the two-disc system ?
View SolutionTwo discs of moments of inertia I1andI2 about their respective axes (normal to the disc and passing through the centre), and rotating with angular speed ω1andω2 are brought into contact face to face with their axes of rotation coincident (i) What is the angular speed of the two-disc system ? (ii) Show that the kinetic energy of the combined system is less than the sum of the initial kinetic energies of the two discs. How do you account for this loss in energy ? Take ω1≠ω2.
View SolutionTwo discs of moments of inertia I1andI2 about their respective axes (normal to the disc and passing through the centre), and rotating with angular speed ω1andω2 are brought into contact face to face with their axes of rotation coincident . What is the angular speed of the two-disc system ?
View SolutionTwo discs of moment of inertia I1 and I2 about their respectively axes (normal) to the disc and passing through the centre) , and rotating with angular speed ω1 and ω2 are brought into contact face to face with their axes of rotation coincident .
What is the loss in kinetic energy of the system in the process ?
View Solution
