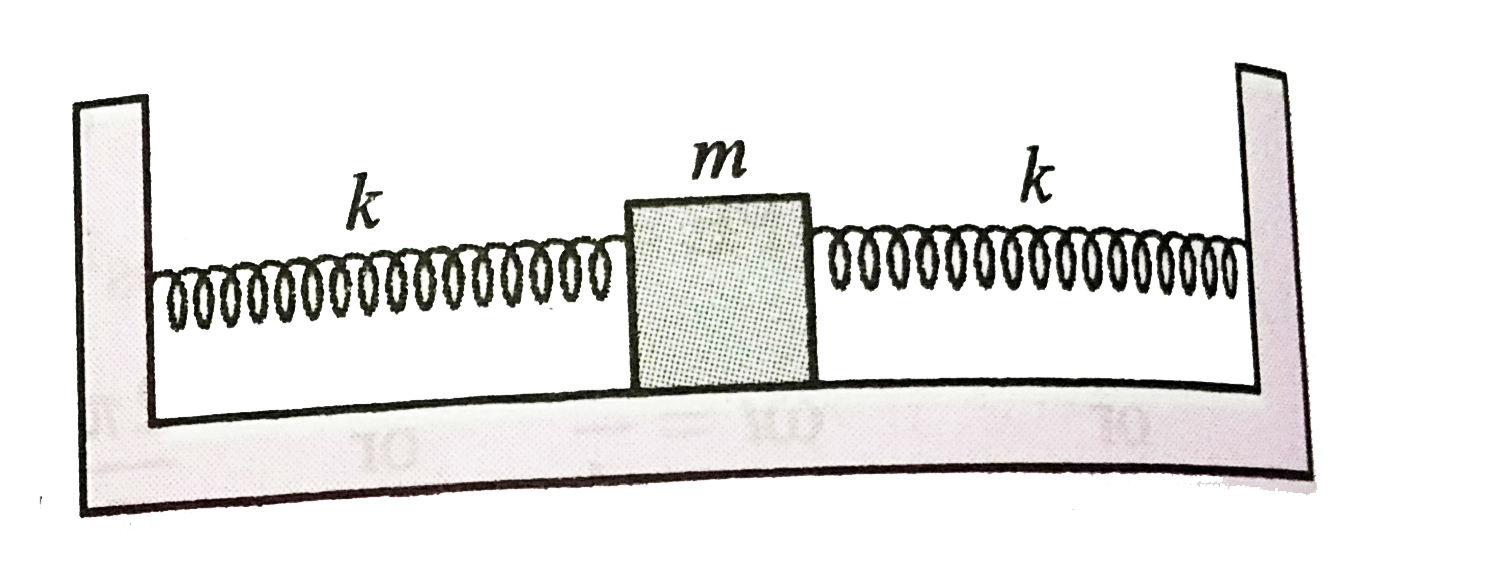
Two identical springs of spring constant k are attached to a black of mass m and to fixed supports in the Fig. 1.45. Show that the mass executes simple harmonic motion when displaced from its rest position on either side.

Text Solution

Verified by Experts

Let the block of mass be displaced by a small distance x to the right side of the equilibrium position as shown in Fig. 1.46. Under this situation the spring on the left side of the mass gets elongated by a length equal to x and that on right side gets compressed by the same length.

The force acting on the mass are
$F_{1} = - k x$
(exerted by the spring on the left side, trying to pull the mass towards the mean position)
$F_{2} = - k x$
(exerted by the spring on the right side, tryying to push the mass towards the mean position)
Hence, the net force acting on the blcok of mass is F = -2kx which is proportional to the displacement x and is directed towards the mean position. Therefore, the motion executed by the block of mass is simple harmonic.

Updated on:21/07/2023

A body of mass 20 g connected to a spring of spring constant k, executes simple harmonic motion with a frequency of $(5 / π)$ Hz. The value of spring constant is
A $4 N m^{- 1}$
B $3 N m^{- 1}$
C $2 N m^{- 1}$
D $5 N m^{- 1}$
A body of mass 20 g connected to a spring of spring constant k, executes simple harmonic motion with a frequency of $(5 / π)$ Hz. The value of spring constant is
A $4 N m^{- 1}$
B $3 N m^{- 1}$
C $2 N m^{- 1}$
D $5 N m^{- 1}$