Question

The equation ${\sin }^{-1}x-{\cos }^{-1}x={\cos }^{-1}\left(\frac{\sqrt{3}}{2}\right)$ has

Options

A. no solution
B. one solution
C. two solutions
D. more than two solutions

Explanation

We have,
`3^(x-1)+5^(x-1)=34`
`implies 3^(x-1)+5^(x-1)=3^(2)+5^(2) impliesx-1=2 implies x=3`
So, the given equation has just one solution.
ALITER The total number of solutions is same as the number of points of intersection of the curves
`y=3^(x-1)+5^(x-1)` and y=34

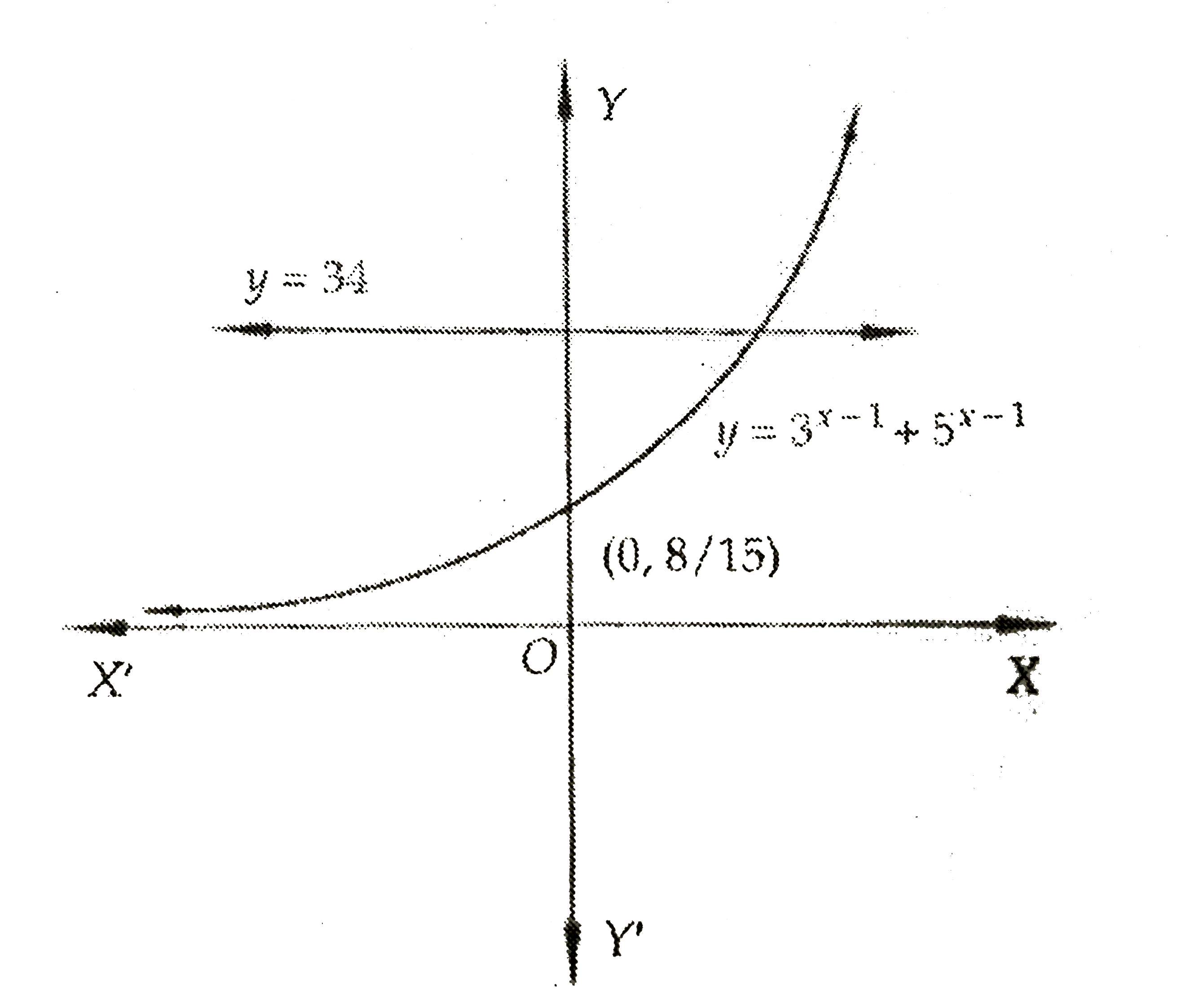
It is evident from the graph that these two curves intersect at exactly one point. Hence, the given equation has exactly one solution.