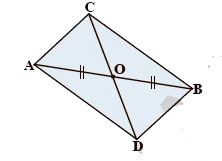
In Fig. 9.24, ABC and ABD are two triangles on the same base AB. If line- segment CD is bisected by AB at O, show that ar(△ABC)=ar(△ABD)
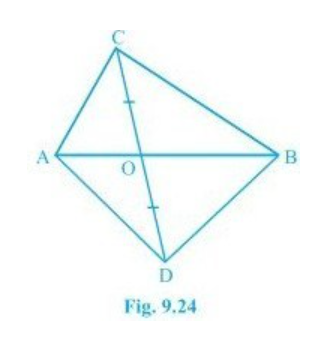
As CD is bisected by AB at O, it means,
AO is the median of triangle ACD and BO is the median of triangle BCD.
As median divides a triangle into two triangles with the same area.
ar(AOC)=ar(AOD) and ar(BOC)=ar(BOD)
So,ar(AOC)+ar(BOC)=ar(AOD)+ar(BOD)
ar(ABC)=ar(ABD)
Topper's Solved these Questions
- BOOK - NCERTCHAPTER - AREAS OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Solved Examples4videos
AREAS OF PARALLELOGRAMS AND TRIANGLES
- BOOK - NCERTCHAPTER - AREAS OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Exercise 9.26videos
AREAS OF PARALLELOGRAMS AND TRIANGLES
- BOOK - NCERTCHAPTER - CIRCLESEXERCISE - EXERCISE 10.22videos
CIRCLES
Similar Questions
In Fig. 9.22, ABCD is a quadrilateral and BE ∣∣ ACand also BE meets DC produced at E. Show that area of ΔADEis equal to the area of the quadrilateral ABCD.
02:11View SolutionABCD is a parallelogram, AE⊥DCand CF⊥AD. If AB = 16 cm, AE = 8 cm and CF = 10 cm, find AD.
02:46View SolutionRecall that two circles are congruent if they have the same radii. Prove that equalchords of congruent circles subtend equal angles at their centres.
04:23View SolutionDetermine the point of symmetry of a regular hexagon.
05:41View SolutionIn the figure above (not to scale), ¯¯¯¯¯¯AB⊥¯¯¯¯¯¯CDandAD is the bisector of ∠BAE.AB=3cm and AC=5cm. Find CD.
02:10View SolutionIn the following figure, AB and BC are equidistant from the centre 'O' of the Show that
(a) ABC is an isosceles triangle.
(b) OB bisects angle ABC.
ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/PS_MATH_X_C13_E02_006_Q01.png" width="80%"gt02:17View SolutionMatch the following Column A to Column B
 02:10View Solution
02:10View SolutionThe inequation represented by the graph given below is :
05:26View SolutionThe inequation that best describes the graph given below is _________
04:09View SolutionThe inequation that best describes the following graph is _________
02:46View Solutionआकृति में, ABC और ABD एक ही आधार AB पर बने दो त्रिभुज है यदि रेखाखड़ CD रेखाखड़ AB से बिंदु O पर समद्विभाजित होता है , तो दर्शाइए कि ar(ABC)=ar(ABD) है
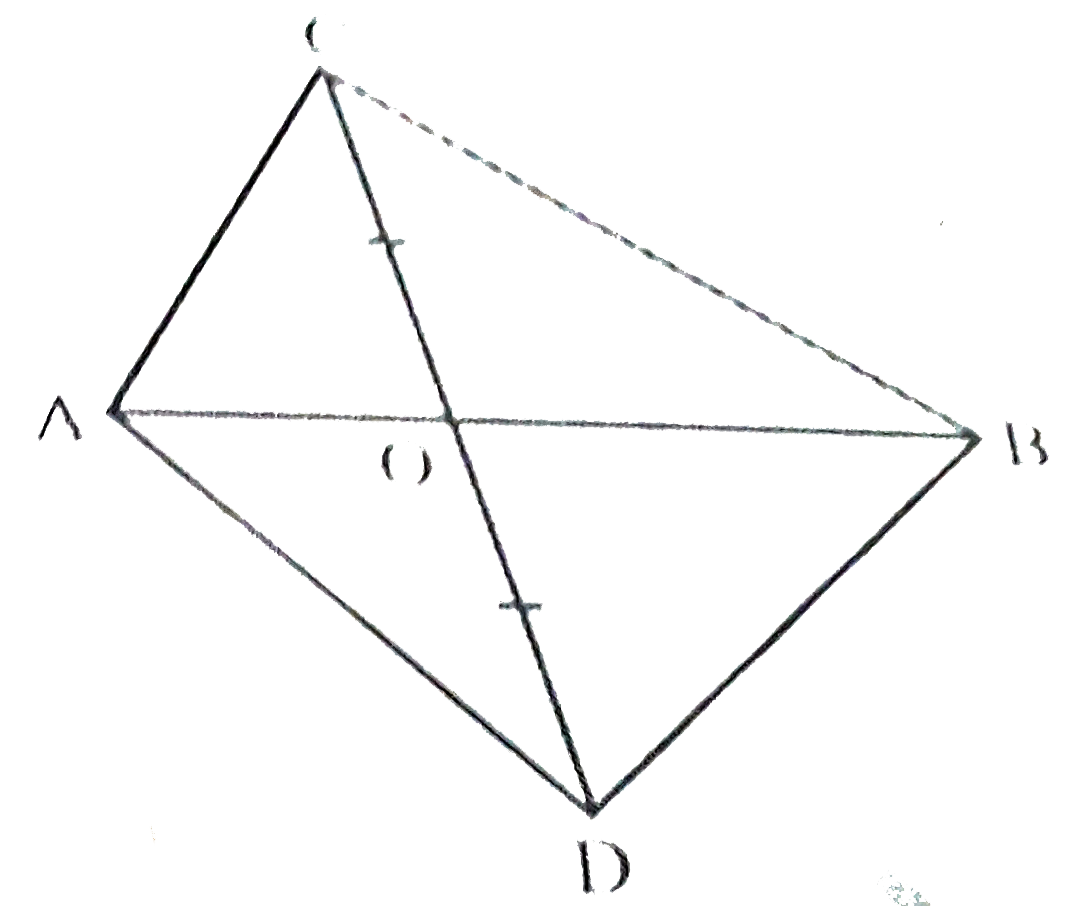 03:57View Solution
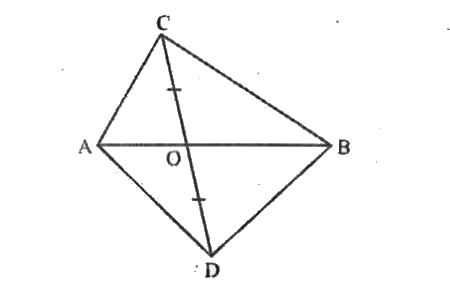
03:57View Solutionचित्र में AB आधार पर दो त्रिभुजों ABC तथा ABD है है यदि रेखाखण्ड CD को रेखा AB बिंदु O पर प्रतिच्छेद करती है, तो प्रदर्शित कीजिए कि ar(ΔABC)=ar(ΔABD)
 04:08View Solution
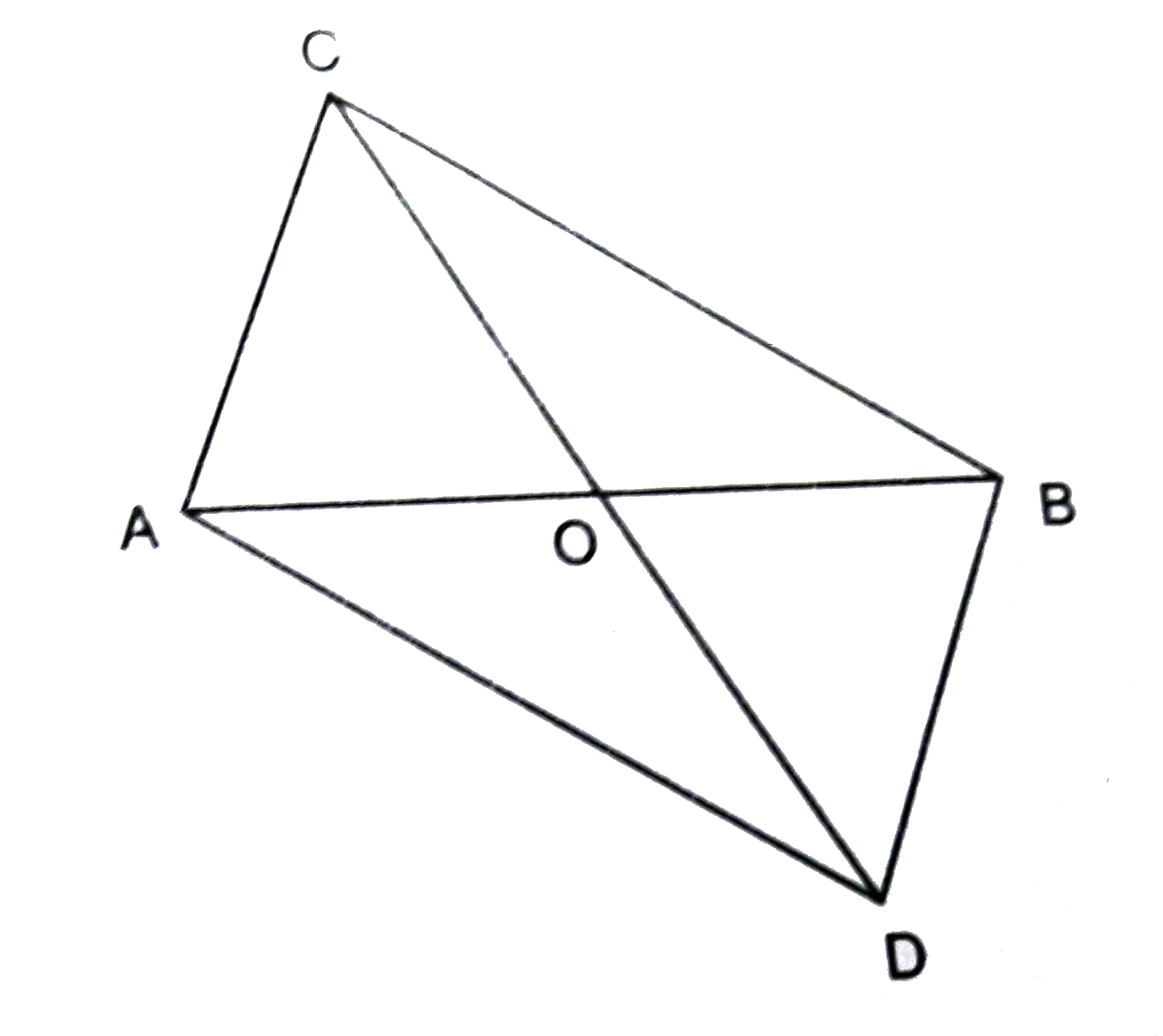
04:08View SolutionIn, ABC and ABD are two triangles on the same base AB. If line- segment CD is bisected by AB at O, show that ar (ABC) = ar (ABD).
 04:32View Solution
04:32View SolutionIn the given figure, ABC and ABD are two triangles on the same base AB . If line - segment CD is bisected by AB at O, show that ar (ABC) = ar (ABD)
05:12View SolutionIn the figure, ΔABC and ΔABD are two triangles on the same base AB. If line segment CD is bisected by ¯¯¯¯¯¯AB at O, show that ar (ΔABC)=ar(ΔABD).
 02:35View Solution
02:35View Solution

