Consider a 70% efficient hydrogen - oxygen fuel cell working under standard conditions at 1 bar and 298 K. Its cell reaction is
H2(g)+12O2(g)→H2O(l).
The work derived from the cell on the consumption of 1.0×10−3 mol of H2(g) is used to compress 1.00 mol of a monoatomic ideal gas in a thermally insulated container. What is the change in the temperature (in K) of the ideal gas?
The standard reduction potentials for the two half - cells are gien below.
O2(g)+4H+(aq)+4e−→2H2O(l),E∘=1.23V,
2H+(aq)+2e−→H2(g),E∘=0.00V.
Use F=96500 C mol−1,=8.314 J mol−1K−1.
The correct Answer is:13.32
To solve the problem step by step, we will follow these steps:
Step 1: Calculate the standard cell potential (E°) of the fuel cell reaction.
The cell reaction is:
H2(g)+12O2(g)→H2O(l)
Using the given standard reduction potentials:
- For the reduction of oxygen:
O2(g)+4H+(aq)+4e−→2H2O(l),E∘=1.23V
- For the oxidation of hydrogen:
2H+(aq)+2e−→H2(g),E∘=0.00V
The overall cell potential (E°) is given by:
E∘=E∘cathode−E∘anode=1.23V−0.00V=1.23V
Step 2: Calculate the standard Gibbs free energy change (ΔG°) for the reaction.
Using the formula:
ΔG∘=−nFE∘
Where:
- n=2 (number of moles of electrons transferred)
- F=96500C/mol
- E∘=1.23V
Calculating ΔG°:
ΔG∘=−2×96500C/mol×1.23V
ΔG∘=−237390J/mol
Step 3: Calculate the work done (W) by the fuel cell on the consumption of 1.0×10−3 mol of H2(g).
Given the efficiency of the fuel cell is 70%, the work done can be calculated as:
W=Efficiency×ΔG∘×moles of H2
W=0.70×(−237390J/mol)×(1.0×10−3mol)
W=0.70×237.390J
W≈166.173J
Step 4: Use the work done to find the change in temperature (ΔT) of the monoatomic ideal gas.
Using the adiabatic process formula:
W=nCvΔT
For a monoatomic ideal gas, Cv=32R and n=1mol:
W=1×32RΔT
Substituting R=8.314J/(mol K):
W=32×8.314ΔT
Now substituting the value of W:
166.173=32×8.314ΔT
Step 5: Solve for ΔT.
Rearranging gives:
ΔT=166.17332×8.314
ΔT=166.17312.471
ΔT≈13.32K
Final Answer:
The change in temperature of the ideal gas is approximately 13.32K.
---
The cell reaction is:
H2(g)+12O2(g)→H2O(l)
- For the reduction of oxygen:
O2(g)+4H+(aq)+4e−→2H2O(l),E∘=1.23V
- For the oxidation of hydrogen:
2H+(aq)+2e−→H2(g),E∘=0.00V
E∘=E∘cathode−E∘anode=1.23V−0.00V=1.23V
Using the formula:
ΔG∘=−nFE∘
Where:
- n=2 (number of moles of electrons transferred)
- F=96500C/mol
- E∘=1.23V
ΔG∘=−2×96500C/mol×1.23V
ΔG∘=−237390J/mol
Given the efficiency of the fuel cell is 70%, the work done can be calculated as:
W=Efficiency×ΔG∘×moles of H2
W=0.70×(−237390J/mol)×(1.0×10−3mol)
W=0.70×237.390J
W≈166.173J
Using the adiabatic process formula:
W=nCvΔT
For a monoatomic ideal gas, Cv=32R and n=1mol:
W=1×32RΔT
Substituting R=8.314J/(mol K):
W=32×8.314ΔT
Now substituting the value of W:
166.173=32×8.314ΔT
Rearranging gives:
ΔT=166.17332×8.314
ΔT=166.17312.471
ΔT≈13.32K
The change in temperature of the ideal gas is approximately 13.32K.
Topper's Solved these Questions
- JEE (ADVANCED ) 2020BOOK - JEE ADVANCED PREVIOUS YEARCHAPTER - JEE (ADVANCED ) 2020EXERCISE - SECTION -II
5 Videos
- MOCK TEST 2022BOOK - JEE ADVANCED PREVIOUS YEARCHAPTER - MOCK TEST 2022EXERCISE - QUESTIONS
14 Videos
- JEE ADVANCEDBOOK - JEE ADVANCED PREVIOUS YEARCHAPTER - JEE ADVANCEDEXERCISE - CHEMISTRY SECTION - IV : Matric Match Type
2 Videos
Similar Questions
In thermodynamics, the P−V work done is given by
w=−∫dVPext.
For a system undergoing a particular process, the work done is ,
w=−∫dV(RTV−b−aV2)
The equation is applicable to a
View SolutionWhich green coloured compound of chromium is formed in borax bead test ?
View SolutionMatch the transformation in column I with appropriate options in column II
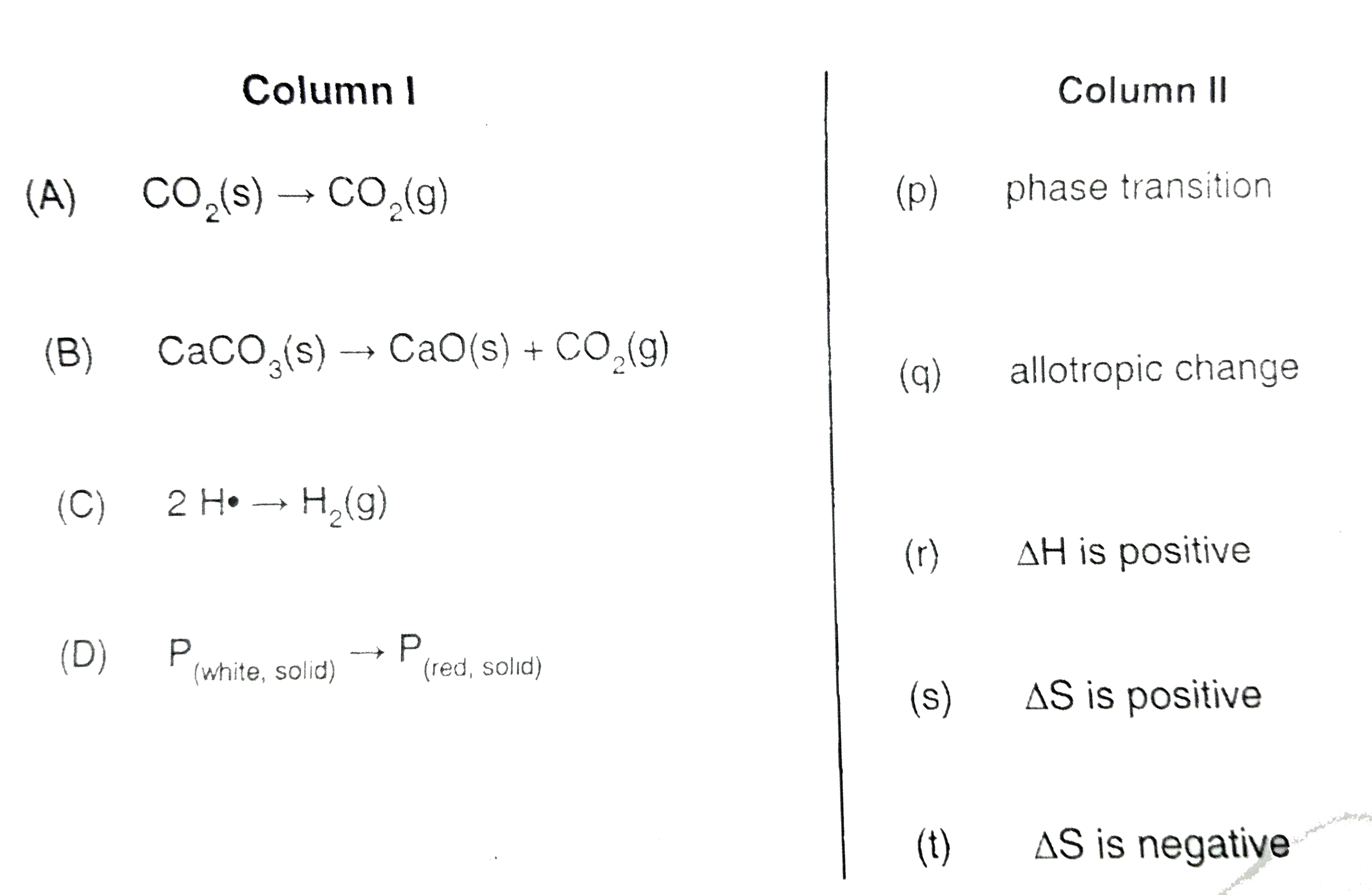
View SolutionFor hydrogen oxygen fuel cell with reaction 2H_(2)(g)+O_(2)(g) rarr 2 H_(2)O(l) DeltaG_(f)^(c-)(H_(2)O)=-237.2kJ mol^(-1) . Hence, EMF of the fuel cell is
View SolutionIn a fuel cell methanol is used as fuel and oxygen gas is used as an oxidizer. The reaction is : CH_(3)OH_((l))+(3)/(2)O_(2(g))rarr CO_((g))+2H_(2)O_((l)) At 298K standard Gibb's energies of formation for CH_(3)OH(l), H_(2)O(l) and CO_(2)(g) are -166.2,-237.2 and -394.4kJ mol^(-1) respectively. If standard enthalpy of combustion of methanol is -726kJ mol^(-1) , efficiency of the fuel cell will be :
View SolutionFor the reaction 2H2(g)+O2(g)→2H2O(l). The standard entropies of H2(g),O2(g) and H2O(l) at 27∘C are 30cal K−1mol−1,50calK−1and15calK−1mol−1respectivley.
Assuming H2 and O2 to behave as ideal gas (without vibrational degree of freedom ) and C(PmH2O(l))=15.5calK−1mol−1 . Determine magnitude of standard entropy (in calK−1) change for the reaction at 177∘C. ltbrlt [Given : R=2calK−1mol−1,In 1.5=0.4]
View SolutionIn a fuel cell methanol is used as fuel and oxygen gas is used as an oxidizer. The reaction is CH3OH(l)+32O2(g)→CO2(g)+2H2O(l) at 298K standard Gibb's energies of formation for CH3OH(l),H2O(l) and CO2(g) are −166.2,−237.2 and −394.4 kJ mol−1 respectively. If standard enthalpy of combustion of methanol is −726 kJ mol−1, efficiency of the fuel cell will be -
View SolutionIn a fuel cell methanol is used as fuel and oxygen gas is used as an oxidizer. The reaction is :
CH3OH(l)+32O2(g)→CO2((g))+2H2O(l)
At 298K standard Gibb's energies of formation for CH3OH(l),H2O(l) and CO2(g) are −166.2,−237.2 and −394.4kJmol−1 respectively. If standard enthalpy of combustion of methanol is −726kJmol−1, efficiency of the fuel cell will be :
View SolutionStandard free energies of formation in kJ/mol) at 298 K are -237.2, -394.4 and -8.2 for H_(2)O(l),CO_(2)(g) and pentane (g) respectively. The value of E_(cell)^(@) for the pentane-oxygen fuel cell is
View Solution298 K पर H2O(l),CO2(g) तथा पेन्टेन (g) के निर्माण की मानक मुक्त ऊजाएं (kJ/mol में) क्रमशः −237.2−394.4 तथा −8.2 है पेन्टेन ऑक्सीजन ईंधन सेल के लिये E∘cell का मान है
View SolutionStandard free energies of formation (in kJ/mol) at 298 K are -237.2, -394.4 and -8.2 for H_(2)O(l), CO_(2)(g) and pentance (g) respectively. The value E_(cell)^(@) for the pentance-oxygen fuel cell is:
View SolutionIn a fuel cell methanol is used as fuel and oxygen is used as an oxidiser. The reaction is : CH_(3)OH(l)+(3)/(2)O_(2)(g)rarr CO_(2)(g)+2H_(2)O(l) At 298 K, standard Gibb's energies of formation for CH_(3)OH(l), H_(2)O(l) and CO_(2)(g) are -166.2, - 237.2 and -394.4 kJ/mol respectively. If standard enthalpy of combustion of methanol is - 726 kJ/mol, effeciency of the fuel cell will be :
View SolutionFor hydrogen - oxygen fuel cell at one atm and 298 K H_(2(g)) + (1)/(2)O_(2(g)) to H_2O_((l)), DeltaG^(@) = -240 kJ E^(@) for the cell approximately , (Given F = 96,500 C )
View SolutionDetermine the entropy change for the reaction given below:
2H2(g)+O2(g)→2H2O(l)
at 300K. If standard entropies of H2(g),O2(g), and H2O(l) are 126.6,201.20, and 68.0JK−1mol−1, respectively.
View SolutionFor hydrogen oxygen fuel cell with reaction 2H_(2)(g)+O_(2)(g) rarr 2 H_(2)O(l) DeltaG_(f)^(c-)(H_(2)O)=-237.2kJ mol^(-1) . Hence, EMF of the fuel cell is 1) +2.46 V 2) -2.46V 3) +1.23 V 4) -1.23V
View Solution
