A projectile has

minimum velocity at the point of projection and maximum at the maximum height

maximum at the point of projection and minimum at the maximum height

same velocity at any point in its path

zero velocity at the maximum height irrespective of the velocity of projection

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:B

To solve the problem regarding the velocities of a projectile at different points in its trajectory, we will analyze the situation using the principles of conservation of mechanical energy.
1. Understanding the Problem:
We need to find the minimum and maximum velocities of a projectile at two specific points: at the point of projection (ground level) and at the maximum height.
2. Initial Conditions:
Let the initial velocity of the projectile at the point of projection be $u$ . At this point, the height $h = 0$ .
3. Applying Conservation of Mechanical Energy:
The total mechanical energy at the point of projection (ground level) is entirely kinetic:
$E_{i} = K_{i} = \frac{1}{2} m u^{2}$
At the maximum height $h = H$ , the potential energy is maximum and kinetic energy is minimum:
$E_{f} = U_{f} + K_{f} = m g h + \frac{1}{2} m v^{2}$
4. Setting Up the Energy Conservation Equation:
According to the conservation of mechanical energy:
$E_{i} = E_{f}$
Therefore, we have:
$\frac{1}{2} m u^{2} = m g h + \frac{1}{2} m v^{2}$
5. Simplifying the Equation:
We can cancel $m$ from both sides (assuming $m \neq 0$ ):
$\frac{1}{2} u^{2} = g h + \frac{1}{2} v^{2}$
Rearranging gives:
$\frac{1}{2} u^{2} - g h = \frac{1}{2} v^{2}$
6. Solving for Velocity $v$ :
Multiply the entire equation by 2:
$u^{2} - 2 g h = v^{2}$
Taking the square root gives us the velocity at height $h$ :
$v = \sqrt{u^{2} - 2 g h}$
7. Finding Maximum and Minimum Velocities:
- Maximum Velocity: Occurs at the point of projection where $h = 0$ :
$v_{max} = \sqrt{u^{2} - 2 g (0)} = \sqrt{u^{2}} = u$
- Minimum Velocity: Occurs at maximum height $h = H$ :
$v_{min} = \sqrt{u^{2} - 2 g H}$
Here, $H$ is the maximum height reached by the projectile.
8. Conclusion:
- The maximum velocity $v_{max}$ is at the point of projection (ground level) and equals $u$ .
- The minimum velocity $v_{min}$ is at the maximum height $H$ .
Final Answer:
- Maximum velocity at the point of projection and minimum velocity at maximum height.

Updated on:18/9/2024

Class 11 PHYSICS PROJECTILE MOTION

Topper's Solved these Questions

Knowledge Check

When we analyse the projectile motion from any accelerated frame $O$ as ${\to r}_{o}, {\to u}_{o}$ and ${\to a}_{o}$ respectively, express the following terms, ${\to r}_{p O} + {\to a}_{p} - {\to r}_{O}, {\to u}_{p O} = {\to u}_{p} - {\to u}_{O}$ and ${\to a}_{p O} = {\to a}_{p} - {\to a}_{O}$
where $P$ stands for projectile. Then using the following kinematical equations of the projectile (For constant acceleration) relative to the accelerating frame ,we have ${\to S}_{p O} = {\to u}_{p O} t + \frac{1}{2} {\to a}_{p O} t^{2}, {\to v}_{p O}$
$= {\to u}_{p O} + {\to a}_{p O} t$ and $v_{p O}^{2} = u_{p O}^{2} + 2 \to a . {\to s}_{p} O$
Using the above expressions, anwer the following
question:A projectile has initial velocity $v_{0}$ realtive to the large plate which is moving with a constant upward acceleration a.
Refering to Q.1, velocity of the projectile relative to $B$ ofter some time

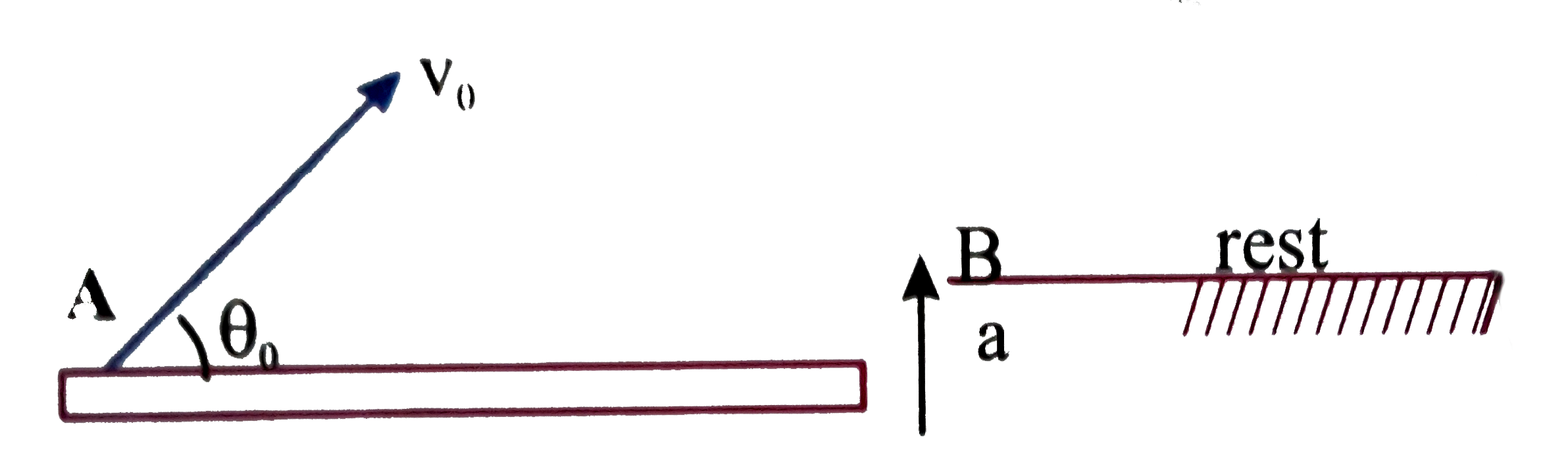A $< v_{0} at an angle θ < θ_{0}$
B $> v_{0} at an angle θ > θ_{0}$
C $> v_{0} at an angle θ = θ_{0}$
D $v_{0} at an angle θ = θ_{0}$
The projectile has only vertical component of velocity at the highest point of its trajectory.
At the highest point, only one component of velocity is present.
AStatement (I) is true, Statement (II) is true , statement (II) is the correct explanation for Statement (I).
BStatement (I) is true, Statement (II) is true , statement (II) is not the correct explanation for Statement (I).
CStatement (I) is true, Statement (II) is false.
DStatement (i) is false, Statement (II) is true.
Neglecting air resistance, a 1.0-kg projectile has an escape velocity of about 11km/s at the surface of Earth. The corresponding escape velocity for a 2.0 kg projectile is:
A3.5 km/s
B5.5 km/s
C7.1 km/s
D11 km/s