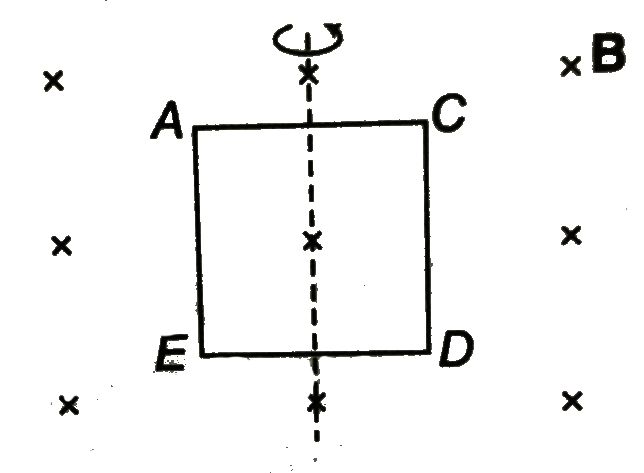
A square loop ACDE of area $20 c m^{2}$ and resistance $5 Ω$ is rotated in a magnetic field B = 2T through $180^{\circ}$
(a) in 0.01 s and (b) in 0.02 s .
Find the magnetic fo e.i and $Δ q$ in both the cases.

Text Solution

Verified by Experts

Let us take the area vector S perpendicular to plane of loop inwards. So initailly dS parallel to B and when it is rotatedy by $180^{\circ}$ , S is anti parallel to B.
Hence initial flux passing through the loop.
$ϕ_{i} = B S {cos 0}^{\circ} = (2) (20 \times 10^{- 4}) (1)$
$= 4 \times 10^{- 3} w b$
Flux passing through the loop when it is rotated by
$180^{\circ}, ϕ_{f} = B S {cos 180}^{\circ}$
$= (2) (20 \times 10^{- 4}) (- 1) = - 4.0 \times 10^{- 3}$ Wb
Therefore , change in flux
$Δ ϕ_{B} = ϕ_{f} - ϕ_{i}, = - 8 \times 10^{- 3}$ Wb
(a) Given $Δ t = 0.01 s, R = 5 Ω, ∴ | e | = ∣ ∣ ∣ \frac{Δ ϕ_{B}}{Δ t} ∣ ∣ ∣$
$= \frac{8 \times 10^{- 3}}{0.01} = 0.8 V, or i = \frac{| e |}{R} = \frac{0.8}{5} = 0.16 A$
and $Δ q = i Δ t = 0.16 \times 0.01, = 1.6 \times 10^{- 3} C$
(b) $Δ t = 0.02 s, ∴ | e | = ∣ ∣ ∣ - \frac{Δ ϕ_{B}}{Δ t} ∣ ∣ ∣$
$= \frac{8 \times 10^{- 3}}{0.02}, 0.4 V, i = \frac{| e |}{R} = \frac{0.4}{5} = 0.08 A$
and $Δ q = i Δ t = (0.08) (0.02),$
$= 16 \times 10^{- 4} C$

Updated on:21/07/2023

A circular conducting loop of area $100 c m^{2}$ and resistance $3 Ω$ is placed in a magnetic field with its plane perpendicular to the field. If the field is spatially uniform but varies with time t (in second) as $B (t) = 1.5 cos ω t$ tesla, the peak value of the current in the loop is
A3 mA
B $5 ω m A$
C $300 ω m A$
D500 mA