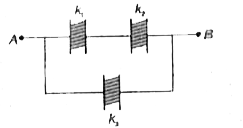
If CAB (equivalent ) = C2 and capacitance of all the capacitors are C in vacuum without dielectric . Find the relation between k1.k2.k3
∵CAB=(Ck1×Ck2Ck1+Ck2)=Ck3
⇒C2=C2C(k1k2k1+k2)+Ck3
⇒12=k1k2k1+k2+k3
⇒12=k1k2+k1k3+k2k3k1+k2
⇒k1+k22=(k1k2+k1k3+k2k3)
Similar Questions
Knowledge Check
A parallel -plate capacitor of area A, plate separation d and capacitance C is filled with four dielectric materials having dielectric constant k1,k2,k3 and k4 as shown in the figure below. If a single dielectric materical is to be used to have the same capacitance C in this capacitor, then its dielectric constant k is given by
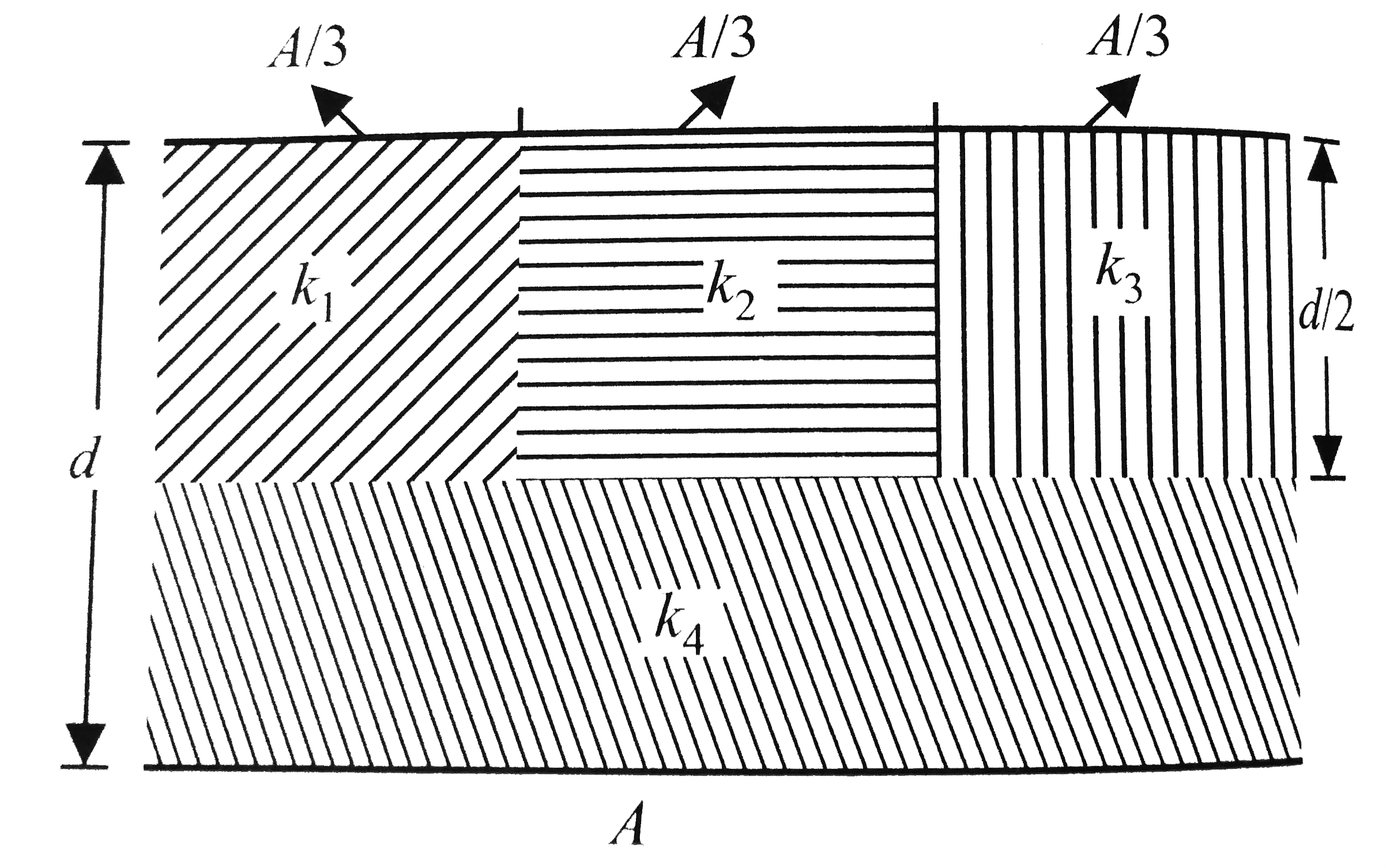 A2k=3k1+k2+k3+1k4B1k=1k1+1k2+1k3+32k4Ck=k1+k2+k3+3k4Dk=23(k1+k2+k3)+2k4
A2k=3k1+k2+k3+1k4B1k=1k1+1k2+1k3+32k4Ck=k1+k2+k3+3k4Dk=23(k1+k2+k3)+2k4A parallel -plate capacitor of area A, plate separation d and capacitance C is filled with four dielectric materials having dielectric constant k1,k2,k3 and k4 as shown in the figure below. If a single dielectric materical is to be used to have the same capacitance C in this capacitor, then its dielectric constant k is given by
 A2k=3k1+k2+k3+1k4B1k=1k1+1k2+1k1=32k4Ck=k1+k2+k+3k4Dk=23(k1+k2+k3)+2k4
A2k=3k1+k2+k3+1k4B1k=1k1+1k2+1k1=32k4Ck=k1+k2+k+3k4Dk=23(k1+k2+k3)+2k4The equivalent capacitance of three capacitors of capacitance C1:C2 and C3 are connected in parallel is 12 units and product C1.C2.C3=48. When the capacitors C1 and C2 are connected in parallel, the equivalent capacitance is 6 units. then the capacitance are
A2,3,7B1.5,2.5,8C1,5,6D4,2,6
In the figure a capacitor is filled with dielectrics K1,K2 and K3. The resultant capacitance is
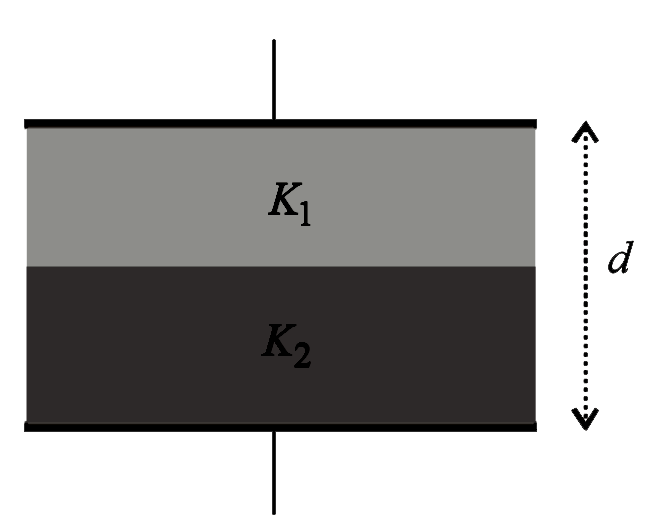
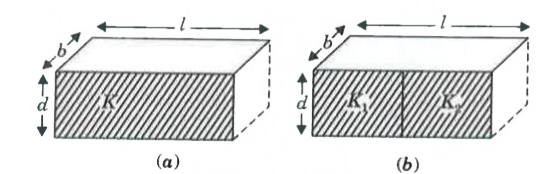
View SolutionTwo identical capacitors of plate dimensions: l×b and plate separation d have dielectric slabs filled in between the space of the plates as shown in the figures. if capacitance of both capacitor is same, Obtain the relation between the dielectric constant K,K1andK2.
View SolutionA parallel plate capacitor of area A, plate separation d and capacitance C is filled with three different dielectric materials having dielectric constant K1,K2 and K3 as shown in fig. If a single dielectric material is to be used to have the same effective capacitance as the above combination then its dielectric constant K is given by :
View SolutionA parallel plate capacitor of area A, plate separation d and capacitance C is filled with three different dielectric materials having dielectric constants k1,k2 and k3 as shown. If a single dielectric naterial is to be used have the same capacitance C in this capacitor, then its dielectric constant k is given by
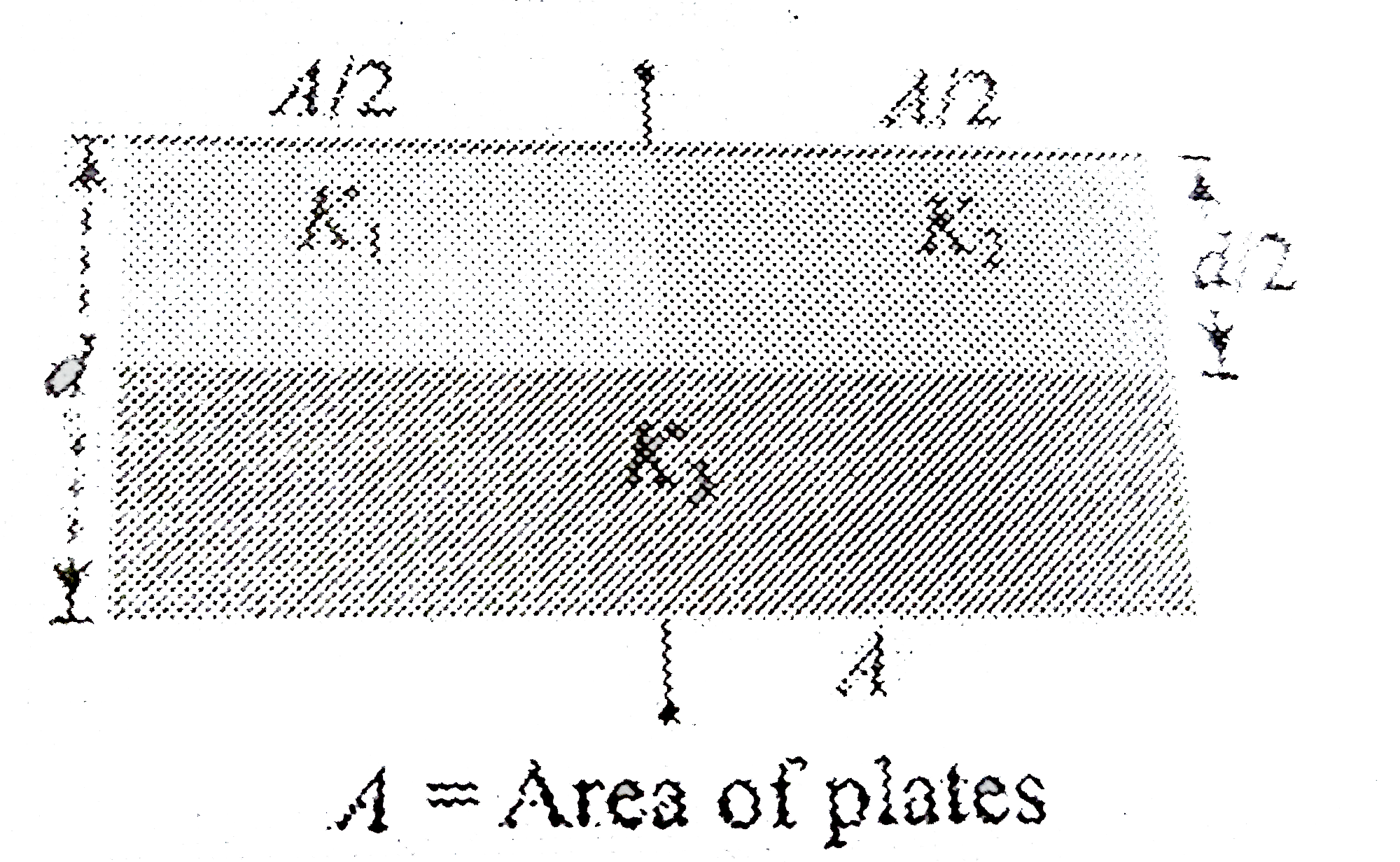
View SolutionA parallel plate capacitor of area A, plate separation d and capacitance C is filled with four dielectric materials hving dielectric constants K1,K2,K3 and K4 as shown in the figure below. If a single dielectric material is to be used to have the same capacitance c in this capacitor, then its dielectric constant K is given by

View Solution