Find the number of distinct solutions of secx+tanx=√3, where 0≤x≤3π.
Text Solution
Here, secx+tanx=√3⇒1+sinx=√3cosx
or √3cosx−sinx=1
√3cosx−sinx=1
dividing both sides by √a2+b2. i.e., √4=2, we get
⇒√32cosx−1xsinx=12
⇒cosπ6cosx−sinπ6sinx=12⇒cos(x+π6)=12
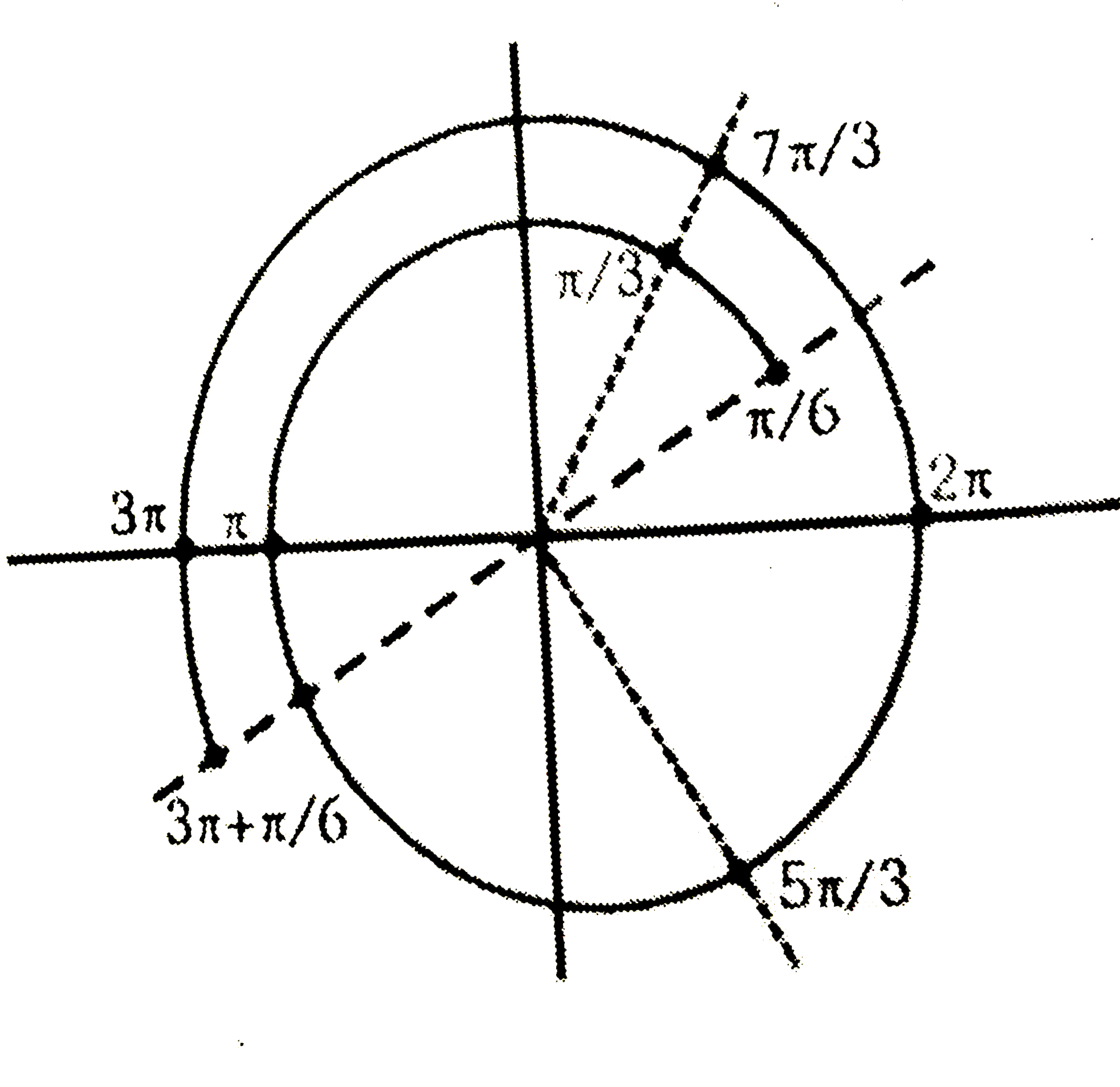
As 0≤x≤3π
π6≤x+π6≤3π+π6
⇒x+π6=π3,5π3,7π3⇒x=π6,3π2,13π6
But at x=3π2,tanx and secx is not defined
∴ Total number of solutions are 2.
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - BASIC MATHS,LOGARITHIM, TRIGNOMETRIC RATIO AND IDENTITIES AND TRIGNOMETRIC EQUATION EXERCISE - DO YOURSELF20videos
BASIC MATHS,LOGARITHIM, TRIGNOMETRIC RATIO AND IDENTITIES AND TRIGNOMETRIC EQUATION
- BOOK - ALLENCHAPTER - BASIC MATHS,LOGARITHIM, TRIGNOMETRIC RATIO AND IDENTITIES AND TRIGNOMETRIC EQUATION EXERCISE - DO YOURSELF 12videos
BASIC MATHS,LOGARITHIM, TRIGNOMETRIC RATIO AND IDENTITIES AND TRIGNOMETRIC EQUATION
- BOOK - ALLENCHAPTER - BASIC MATHS LOGARITHIM TRIGNOMETRIC RATIO AND IDENTITIES AND TRIGNOMETRIC EQUATION EXERCISE - ILLUSTRATIONS39videos
BASIC MATHS LOGARITHIM TRIGNOMETRIC RATIO AND IDENTITIES AND TRIGNOMETRIC EQUATION
- BOOK - ALLENCHAPTER - SOLUTION AND PROPERTIES OF TRIANGLEEXERCISE - All Questions62videos
SOLUTION AND PROPERTIES OF TRIANGLE
Similar Questions
Knowledge Check
- Question 1 - Select One
The number of distinct solutions of secxtanx=√3, where 0≤x≤3π is
A1B2C3D4 - Question 2 - Select One
General solution of √2secx+tanx=1 is
A2nπ+π4,n∈ZB2nπ−π4,n∈ZC2nπ+π8,n∈ZD2nπ−π8,n∈Z
Find the number of distinct solution of secx+tanx=√3
View SolutionFind the number of distinct solutions of secx+tanx=√3 , where 0≤x≤3π .
View SolutionFind the number of solutions of tanx+secx=2cosx in [0,2π]
View SolutionFind the number of solutions of tanx+secx=2cosx in [0,2π]
View SolutionFind the number of solutions of cosx=|1+sinx|,0≤x≤3π
View SolutionFind the number of solutions of cosx=|1+sinx|,0≤x≤3π
View Solution

