Answer the following by appropriately matching the lists based on the information given in the paragraph.
Let $f (x) = sin (π cos x)$ and $g (x) = cos (2 π sin x)$ be two functions defined for $x > 0$ . Define the following sets whose elements are written in the increasing order:
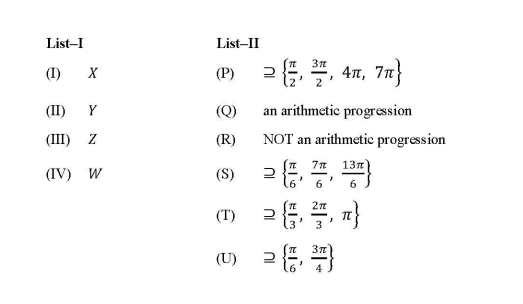
$X = {x : f (x) = 0}, Y = {x : f^{'} (x) = 0}, Z = {x : g (x) = 0}, W = {x : g^{'} (x) = 0}$
List-I contains the sets $X, Y, Z$ and W. List-II contains such some information regarding these sets:
$Answer the following by appropriately matching the lists based on the information given in the paragraph. Let f(x) = sin(picosx) and g(x)=cos(2pi sinx) be two functions defined for x gt0. Define the following sets whose elements are written in the increasing order: X={x:f(x)=0}, Y={x:f^(')(x)=0}, Z={x:g(x)=0}, W={x:g^(')(x)=0} List-I contains the sets X,Y,Z and W. List-II contains such some information regarding these sets: Which of the following is the only CORRECT combinations?$
Which of the following is the only CORRECT combinations?

(II),(R ), (S)

(I), (P), (R )

(II), (Q), (T)

(I), (Q), (U)

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Answer

Step by step video, text & image solution for Answer the following by appropriately matching the lists based on the information given in the paragraph. Let f(x) = sin(picosx) and g(x)=cos(2pi sinx) be two functions defined for x gt0. Define the following sets whose elements are written in the increasing order: X={x:f(x)=0}, Y={x:f^(')(x)=0}, Z={x:g(x)=0}, W={x:g^(')(x)=0} List-I contains the sets X,Y,Z and W. List-II contains such some information regarding these sets: Which of the following is the only CORRECT combinations? by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 MATHS LOGARITHM AND ITS APPLICATIONS

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One or More
Let f(x)= $sin (\frac{π}{x})$ and $D = {x : f (x) > 0)$ , then D contains
A $(\frac{1}{3}, \frac{1}{2})$
B $(\frac{1}{5}, \frac{1}{4})$
C $(- 1, - \frac{1}{2})$
D $(- π, - \frac{1}{2})$
Question 2 - Select One
A function f is defined by $f (x) = x^{x} sin x$ in $[0, π]$ . Which of the following is not correct?
Af is continuous in $[0, π]$
Bf is defferebtiable in $(0, π)$
C $f (0) = f (π)$
DRolle's theorme is not applicable to f x on $[0, π]$
Question 3 - Select One
Let f(x)= $sin (π cos x)$ and g(x)= $cos (1 π sin x)$ e two function defined for $x > 0$ define the following sets whose elements are written in increasing order.
$X = {x : f (x) = 0}, Y = {x : f' (x) = 0}$
$Z = {x : g (x) = 0}, W = {x : g' (x) = 0}$
$\begin{matrix} List I & List II (I) X & (P) \supseteq {\frac{π}{2}, \frac{3 π}{2}, 4 π, 7 π} (I I) Y & (Q) an arithmetic progression (I I I) Z & (R) not an arithmetic progression (I V) W & (S) \supseteq {\frac{π}{6}, \frac{7 π}{6}, \frac{13 π}{6}} (T) \supseteq {\frac{π}{3}, \frac{2 π}{3}, π} (U) \supseteq {\frac{π}{6}, \frac{3 π}{4}} \end{matrix}$
Q. Which of th following is the only correct combination
AIV-(P),(R),(S)
BIII-(R),(U)
CIII-(P),(Q),(U)
DIV-(Q),(T)

ALLEN-BASIC MATHS,LOGARITHIM, TRIGNOMETRIC RATIO AND IDENTITIES AND TRIGNOMETRIC EQUATION -EXERCISE (JA)

Home
Ask Doubt
Profile

The correct Answer is:C

Topper's Solved these Questions

Similar Questions

Knowledge Check

Let f(x)=sin(πx) and D={x:f(x)>0), then D contains

A function f is defined by f(x)=xxsinx in [0,π]. Which of the following is not correct?

ALLEN-BASIC MATHS,LOGARITHIM, TRIGNOMETRIC RATIO AND IDENTITIES AND TRIGNOMETRIC EQUATION -EXERCISE (JA)

Let f(x)= $sin (\frac{π}{x})$ and $D = {x : f (x) > 0)$ , then D contains

A function f is defined by $f (x) = x^{x} sin x$ in $[0, π]$ . Which of the following is not correct?