Solve for x: (i) 10x2−27x+5=0 (ii) pqx2−(p2+q2)x+pq=0
Video Solution
Text Solution
The correct Answer is:(i) 52,15
(ii) pq,qp
(i) 10x2−27x+5=0 ltBrgt x=−b±√b2−4ac2a=27±√(−27)2−4(10)(5)20
=27±2320⇒x=52,15
(ii) (pq)x2−(p2+q2)x+(pq)=0
x=(p2+q2)±√(p2+q2)2−4(pq)(pq)2pq
=(p2+q2)±(p2−q2)2pq⇒x=pq,qp
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - BEGINNER S BOX 72videos
BASIC MATHEMATICS USED IN PHYSICS &VECTORS
- BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - BEGINNER S BOX 82videos
BASIC MATHEMATICS USED IN PHYSICS &VECTORS
- BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - BEGINNER S BOX 51videos
BASIC MATHEMATICS USED IN PHYSICS &VECTORS
- BOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V B19videos
CENTRE OF MASS
Similar Questions
Knowledge Check
- Question 1 - Select One
(i) x2+6x+5=0
(ii) y2−10y+21=0Ax = y or no relation can be established between x and y.Bx≤yCx≥yDx<y - Question 2 - Select One
(i)x2−10x+21=0
(ii) y2−11y+18=0Ax<yBx =y or no relation can be established between x and y.Cx≥yDx>y
Solve: (i) 27x2−10x+1=0.
(ii) 21x2−28x+10=0.View SolutionSolve: (i) 3x2−4x+203=0.
(ii) x2−2x+23=0.View SolutionSolve using formula :
(i) x2−4x−5=0 (ii) x2−7x−3=0
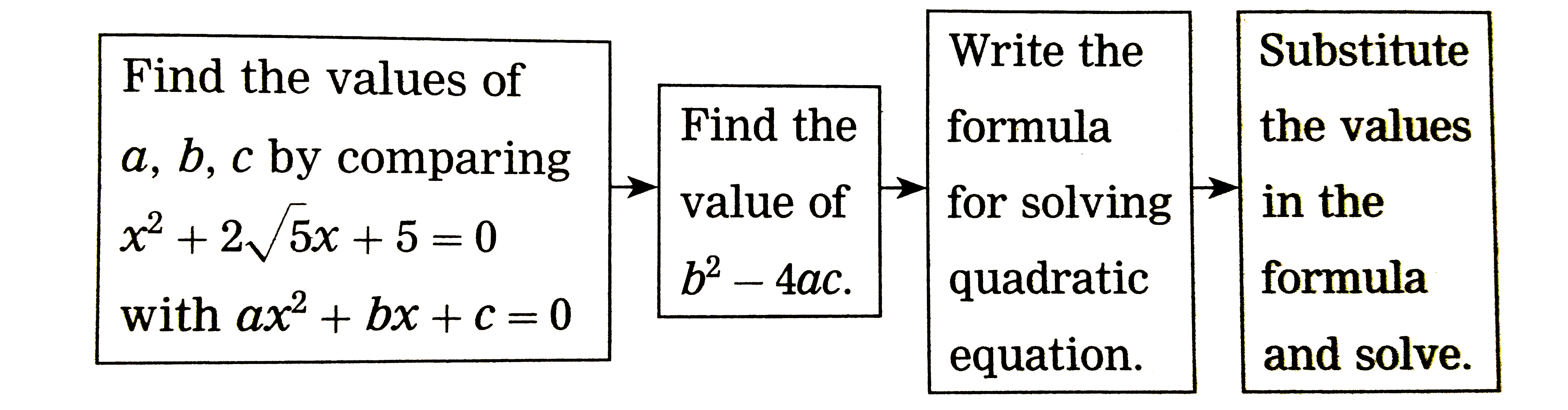
(iii) With the help of flow chart given below solve the equation x2+2√5x+5=0 using the formula :
 View Solution
View Solutiona2p2x2−q2=0,x=
View SolutionSolve log10(x2−2x−2)≤0.
View SolutionIf α,β are the roots of the equation px2−qx+r=0, then the equation whose roots are α2+rpandβ2+rp is (i) p3x2+pq2x+r=0 (ii) px2−qx+r=0 (iii) p3x2−pq2x+q2r=0 (iv) px2+qx−r=0
View Solution

