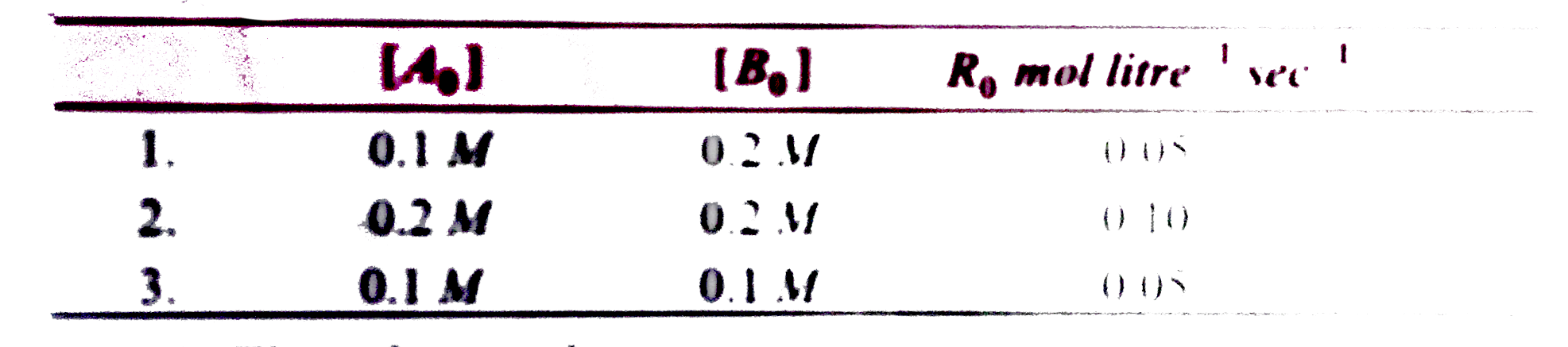For a reaction A+2B→ products, when B is taken in excess, then the rate law expression and order is
To solve the problem, we need to determine the rate law expression and the order of the reaction for the given reaction A+2B→products when reactant B is in excess.
1. Identify the Reaction and Conditions:
The reaction is A+2B→products. We are told that B is taken in excess.
2. Understand the Concept of Excess Reactant:
When one reactant (in this case, B) is in excess, its concentration does not change significantly during the reaction. Therefore, it can be treated as a constant.
3. Write the General Rate Law Expression:
The general rate law for a reaction can be expressed as:
Rate=k(A)m(B)n
where k is the rate constant, m is the order with respect to A, and n is the order with respect to B.
4. Substitute the Excess Condition:
Since B is in excess, we can consider its concentration as constant. We can denote this constant concentration of B as (B)0. Thus, we can rewrite the rate law as:
Rate=k(A)m(B)n0
Since (B)0 is constant, we can combine it with the rate constant k to form a new constant k′:
Rate=k′(A)m
5. Determine the Order of the Reaction:
In this case, the rate of the reaction depends only on the concentration of A, and the order of the reaction with respect to A is 1 (since we typically consider m=1 for a first-order reaction). Therefore, the overall order of the reaction is also 1.
6. Final Rate Law Expression:
The final rate law expression, considering B is in excess, can be simplified to:
Rate=k′(A)1
or simply:
Rate=k′(A)
Conclusion:
- Rate Law Expression: Rate=k′(A)
- Order of Reaction: 1 (pseudo first-order reaction)
The reaction is A+2B→products. We are told that B is taken in excess.
When one reactant (in this case, B) is in excess, its concentration does not change significantly during the reaction. Therefore, it can be treated as a constant.
The general rate law for a reaction can be expressed as:
Rate=k(A)m(B)n
where k is the rate constant, m is the order with respect to A, and n is the order with respect to B.
Since B is in excess, we can consider its concentration as constant. We can denote this constant concentration of B as (B)0. Thus, we can rewrite the rate law as:
Rate=k(A)m(B)n0
Since (B)0 is constant, we can combine it with the rate constant k to form a new constant k′:
Rate=k′(A)m
In this case, the rate of the reaction depends only on the concentration of A, and the order of the reaction with respect to A is 1 (since we typically consider m=1 for a first-order reaction). Therefore, the overall order of the reaction is also 1.
The final rate law expression, considering B is in excess, can be simplified to:
Rate=k′(A)1
or simply:
Rate=k′(A)
- Rate Law Expression: Rate=k′(A)
- Order of Reaction: 1 (pseudo first-order reaction)
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - CHEMICAL KINETICS EXERCISE - EVALUATE YOURSELF - 12videos
CHEMICAL KINETICS
- BOOK - NARAYNACHAPTER - CHEMICAL KINETICS EXERCISE - EVALUATE YOURSELF - 23videos
CHEMICAL KINETICS
- BOOK - NARAYNACHAPTER - CARBOXYLIC ACIDEXERCISE - All Questions288videos
CARBOXYLIC ACID
- BOOK - NARAYNACHAPTER - CHEMISTRY IN EVERYDAY LIFEEXERCISE - ASSERTION & REASON TYPE QUESTIONS10videos
CHEMISTRY IN EVERYDAY LIFE
Similar Questions
Knowledge Check
- Question 1 - Select One
For a reaction A+B→ products, when B is taken in excess, then the rate law expression can be written as
ARate =K[A]1[B]0BRate =K[A]1[B]2CRate =K[A][B]DRate =K[A]2[B]1 - Question 2 - Select One
In the reaction A+B⇒Products, if B is taken in excess, then it is an example of
ASecond order reactionBZero order reactionCFractional order reactionDFirst order reaction - Question 3 - Select One
The rate law of reaction A+2B→product given by
r=K(A)2(B)1. If A is taken in excess the order of the reaction will be :-AzeroB1C2D3
For a reaction A+B→ Products, the rate law is -Rate =k[A][B]3/2
Can the reaction be an elementray reaction ? Explain.View SolutionFor the reaction , A+B→ Products, it is found that the order of A is 2 and of B is 3 in the rate expression. When concentration of both is doubled the rate will increased by
View Solutionfor a reaction 2A+3B→Products, the rate law expression is given by rate = K(A)1(B)2 . The order of the reaction with respect to A,B and over all order of reaction ar
View SolutionFor the reaction A+B→ products, it is found that order of A is 1 and order of B is 1/2. When concentrations of both A & B are increased four times the rate will increase by a factor
View SolutionFor the second order reaction,
A+B→products
when a moles of A react with b moles of B, the rate equation is given by
k2t=1a−bInb(a−x)a(b−x)
when a>>b, the rate expression becomes that ofView Solution