The sum of last 3 digits of 3100 is
A
1
B
2
C
3
D
4
Video Solution
Text Solution
The correct Answer is:A
(a) We have
3100=(34)25
=(81)25
=(80+1)25
=25C0⋅(80)25+25C1⋅(80)24+....+25C22(80)3+25C23(80)2+25C24(80)+25C25
=103['25C0825×1022+25C1×824×1021+...+25C2×83]+25×242×(80)2+25×80+1
=103m+1920000+2000+1, where m∈N
Thus, last three digits of 3100 are 001,
|
Topper's Solved these Questions
- BINOMIAL THEOREMBOOK - CENGAGECHAPTER - BINOMIAL THEOREMEXERCISE - Multiple Correct Answer
4 Videos
- BINOMIAL THEOREMBOOK - CENGAGECHAPTER - BINOMIAL THEOREMEXERCISE - Comprehension
11 Videos
- BINOMIAL THEOREMBOOK - CENGAGECHAPTER - BINOMIAL THEOREMEXERCISE - JEE Previous Year
16 Videos
- AREA UNDER CURVESBOOK - CENGAGECHAPTER - AREA UNDER CURVESEXERCISE - Question Bank
20 Videos
- BINOMIAL THEORMBOOK - CENGAGECHAPTER - BINOMIAL THEORMEXERCISE - Question Bank
31 Videos
Similar Questions
Knowledge Check
The last digit of 340 is
A1B3C7D9If a certain number of two digits is divided by the sum of its digits, the quotient is 6 and the remainder is 3. If the digits are reversed and the resulting number is divided by the sum of the digits, the quotient is 4 and the remainder is 9. The sum of the digits of the number is
A6B9C12D4The last digit of 2137753 is
A9B7C3D1

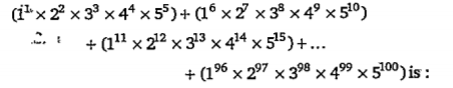 is:
is: