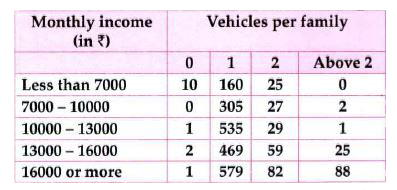
An organization selected 2400 families at random and surveyed them to determine a relationship between income level and the number of vehicles in a family. The information gathered is listed in the table below:
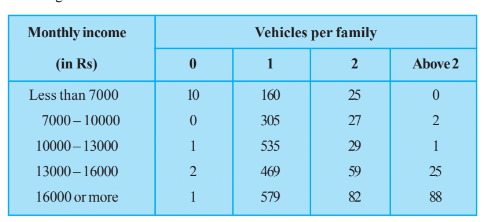
Suppose a family is chosen. Find the probability that the family chosen is
(i) earning Rs 10000 – 13000 per month and owning exactly 2 vehicles.
(ii)
earning Rs 16000 or more per month and owning exactly 1 vehicle.
(iii)
earning less than Rs 7000 per month and does not own any vehicle.
(iv)
earning Rs 13000 – 16000 per month and owning more than 2 vehicles.
(v) owning not more than 1 vehicle
Given
According to the given data
Total number of families = 2400
(i) earning ₹10000 – 13000 per month and owning exactly 2 vehicles.
The probability that the family chosen is earning ₹10000 – 13000 per month and owning exactly 2 vehicles =
(Numbers of families earning ₹10000 –13000 per month and owning exactly 2 vehicles) / (Total number of families)
= 29/2400
(ii) earning ₹16000 or more per month and owning exactly 1 vehicle.
Number of families earning ₹16000 or more per month and owning exactly 1 vehicle = 579
The probability that the family chosen is earning ₹16000 or more per month and owning exactly 1 vehicle =
(Number of families earning ₹16000 or more per month and owning exactly 1 vehicle) / (Total number of families)
=579/2400
(iii) earning less than ₹7000 per month and does not own any vehicle.
Number of families earning less than ₹7000 per month and does not own any vehicle = 10
The probability that the family chosen is earning less than ₹7000 per month and does not own any vehicle =
(Number of families earning less than ₹7000 per month and does not own any vehicle)/(Total number of families)
= 10/2400 = 1/240
(iv) earning ₹13000 – 16000 per month and owning more than 2 vehicles.
Number of families earning ₹13000-16000 per month and owning more than 2 vehicles = 25
The probability that the family chosen is earning ₹13000 – 16000 per month and owning more than 2 vehicles =
(Number of families earning ₹13000-16000 per month and owning more than 2 vehicles ) / (Total number of families)
= 25/2400 = 1/96
(v) owning not more than 1 vehicle.
Number of families owning not more than 1 vehicle = 10+160+0+305+1+535+2+469+1+579 = 2062
The probability that the family chosen owns not more than 1 vehicle = 2062/2400 = 1031/1200
Similar Questions
An organisation selected 2400 families at random and surveyed them to determine relationship between income level and the number of vechicles in a family. The information gathered is listed in the table below:

Suppose a family is chosen. Find the probability that the family chosen is :
(i) earning Rs. 10000-13000 per month and owning exactly 2 vehicles.
(ii) earning Rs. 16000 or more per month and owning exactly 1 vehicle.
(iii) earning less than Rs. 7000 per month and does not own any vehicle.
(iv) earning Rs. 13000-16000 per month and owning more than 2 vehicles.
(v) owning not more than 1 vehicle.
View SolutionAn organisation selected 2400 families at random and surveyed them to determine a relationship between income level and the number of vehicles in a family. The information gathered is listed in the table below.
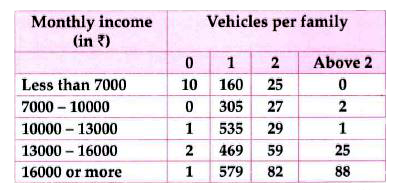
Suppose a family is chosen. Find the probability that the family chosen is
earning Rs 10000 13000 per month and owningexactly 2 vehicles.
View SolutionAn organisation selected 2400 families at random and surveyed them to determine a relationship between income level and the number of vehicles in a family . The information gathered is listed in the table below
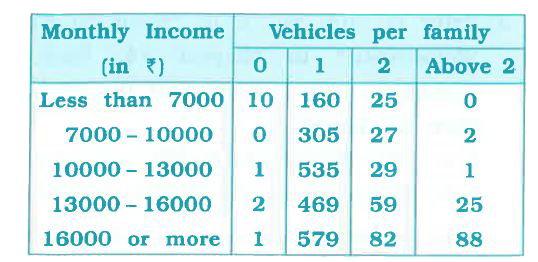
Suppose a family is chosen, Find probability that the family chosen is
earning Rs 10000 - Rs 13000 per month and owning exactly 2 vehicles.
View SolutionAn organisation selected 2400 families at random and surveyed them to determine a relationship between income level and the number of vehicles in a family. The information gathered is listed in the table below.
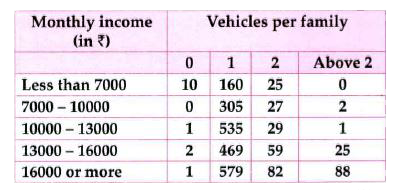
Suppose a family is chosen. Find the probability that the family chosen is
earning Rs 16000 or more per month and owning exactly 1 vehicle.
View SolutionAn organisation selected 2400 families at random and surveyed them to determine a relationship between income level and the number of vehicles in a family. The information gathered is listed in the table below.
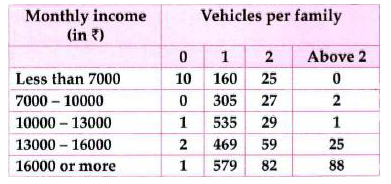
Suppose a family is chosen. Find the probability that the family chosen is
earning Rs 13000-16000 per month and owning more than 2 vehicles.
View SolutionAn organisation selected 2400 families at random and surveyed them to determine a relationship between income level and the number of vehicles in a family . The information gathered is listed in the table below
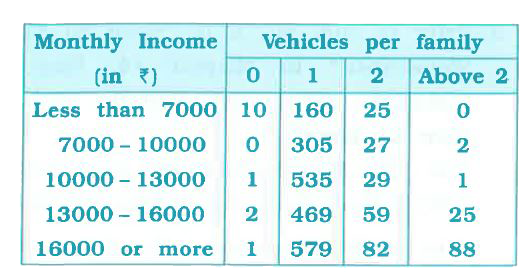
Suppose a family is chosen, Find probability that the family chosen is
earning Rs 13000 - Rs 16000 per month and owning more than 2 vehicles.
View SolutionAn organisation selected 2400 families at random and surveyed them to determine a relationship between income level and the number of vehicles in a family . The information gathered is listed in the table below
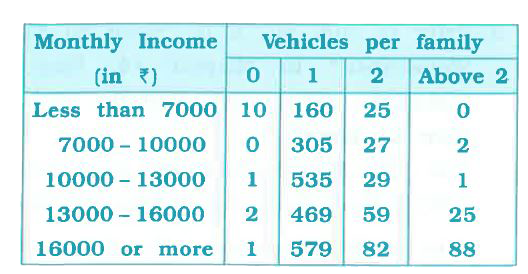
Suppose a family is chosen, Find probability that the family chosen is
earning Rs 16000 or more per month and owning exactly 1 vehicle.
View SolutionAn organisation selected 2400 families at random and surveyed them to determine a relationship between income level and the number of vehicles in a family. The information gathered is listed in the table below.
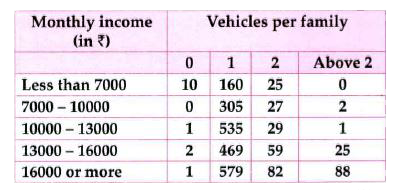
Suppose a family is chosen. Find the probability that the family chosen is
owning not more than 1 vehicle
View SolutionAn organisation selected 2400 families at random and surveyed them to determine a relationship between income level and the number of vehicles in a family. The information gathered is listed in the table below.
Suppose a family is chosen. Find the probability that the family chosen is
earning less than RS 7000 per month and does not own any vehicle
View SolutionAn organisation selected 2400 families at random and surveyed them to determine a relationship between income level and the number of vehicles in a family . The information gathered is listed in the table below
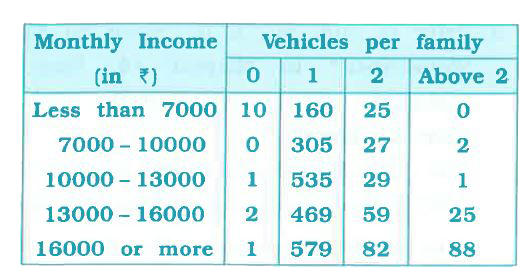
Suppose a family is chosen, Find probability that the family chosen is
owning not more than 1 vehicle.
View Solution
