Objective : To find the mid - point of a line segment using paper folding Procedure : make a line segment on a paper by folding it and name it PQ. Fold the line segment PQ in such a way that P falls on Q and mark the point of intersection of the line segment and crease formed by folding the paper as M. M is the midpoint of PQ. Solution in Tamil
Step by step video & image solution for Objective : To find the mid - point of a line segment using paper folding Procedure : make a line segment on a paper by folding it and name it PQ. Fold the line segment PQ in such a way that P falls on Q and mark the point of intersection of the line segment and crease formed by folding the paper as M. M is the midpoint of PQ. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- GEOMETRYBOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - Activity - 9
1 Videos
- GEOMETRYBOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - Activity - 10
1 Videos
- GEOMETRYBOOK - FULL MARKSCHAPTER - GEOMETRYEXERCISE - Activity - 7
1 Videos
- COORDINATE GEOMETRYBOOK - FULL MARKSCHAPTER - COORDINATE GEOMETRYEXERCISE - TEXTBOOK ACTIVITY
2 Videos
- MENSURATION BOOK - FULL MARKSCHAPTER - MENSURATION EXERCISE - Assignment (II. Answer the following questions)
8 Videos
Similar Questions
Objective : To construct a perpendicular to a line segment from as external point using paper folding. Procedure : Draw a line segment AB and mark an external point P. Move B along BA till the fold passes through P and crease it along that line. The crease thus formed is the perpendicular to AB through the external point P.
View SolutionObjective : To locate the Orthocentre of a triangle using paper folding.
Procedure : Using the above Activity with any two vertices of the triangle as external points, construct perpendiculars to opposite sides. The point of intersection of the perpendiculars is the Orthocentre of the given triangle.
View SolutionProcedure :
(i) Draw a circle of many radius with centre O.
(ii) Mark any four points A,B,C and D on the boundary. Make a cyclic quadrilateral ABCD and name the angles as in Fig. 1
(iii) Make a replica of the cyclic quadrilateral ABCD with the help of tracing paper
(iv) Make the cutout of the angles A,B,C and D as in Fig. 2
(v) Paste the angle cutout ∠1,∠2,∠3 and ∠4 adjacent to the angles opposite to A,B,C and D as in Fig . 3
Measure the angles ∠1+∠3, and ∠2+∠4.
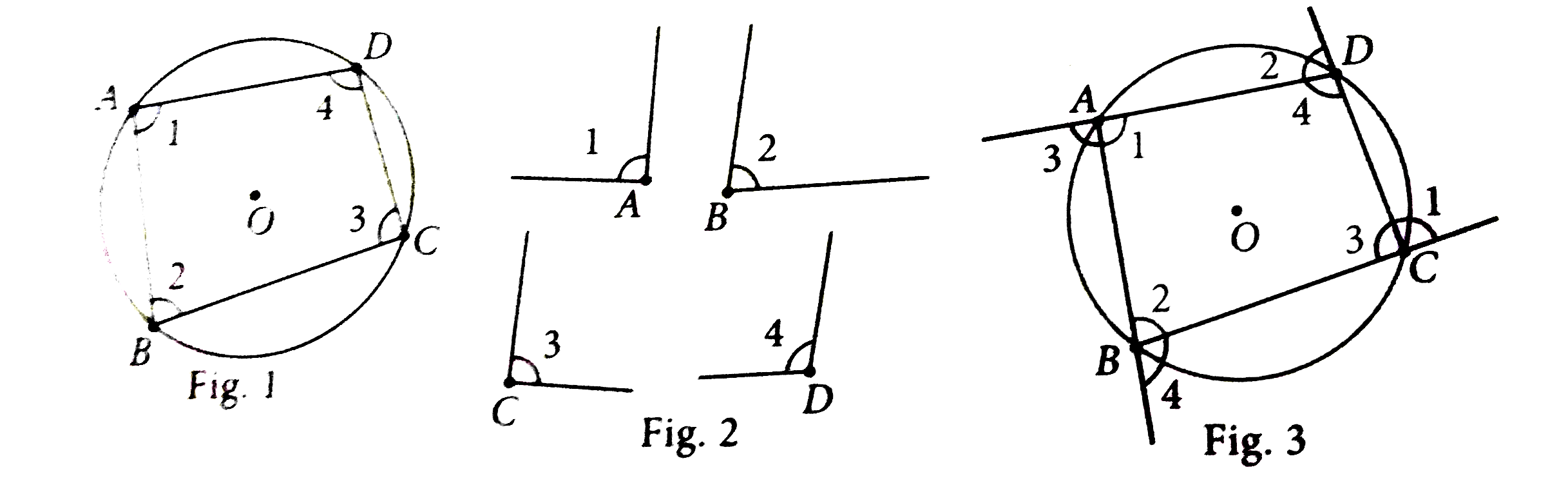
Observe the Fig. 3 and complete the following :
(i)∠A+∠C=.......(ii)∠B+∠D=.......(iii)∠C+∠A=.......(iv)∠D+∠B=.......
2. Sum of opposite angles of a cyclic quadrilateral is . . . . . . . .
3. The opposite angles of a cyclic quadrilateral is . . . . . . .
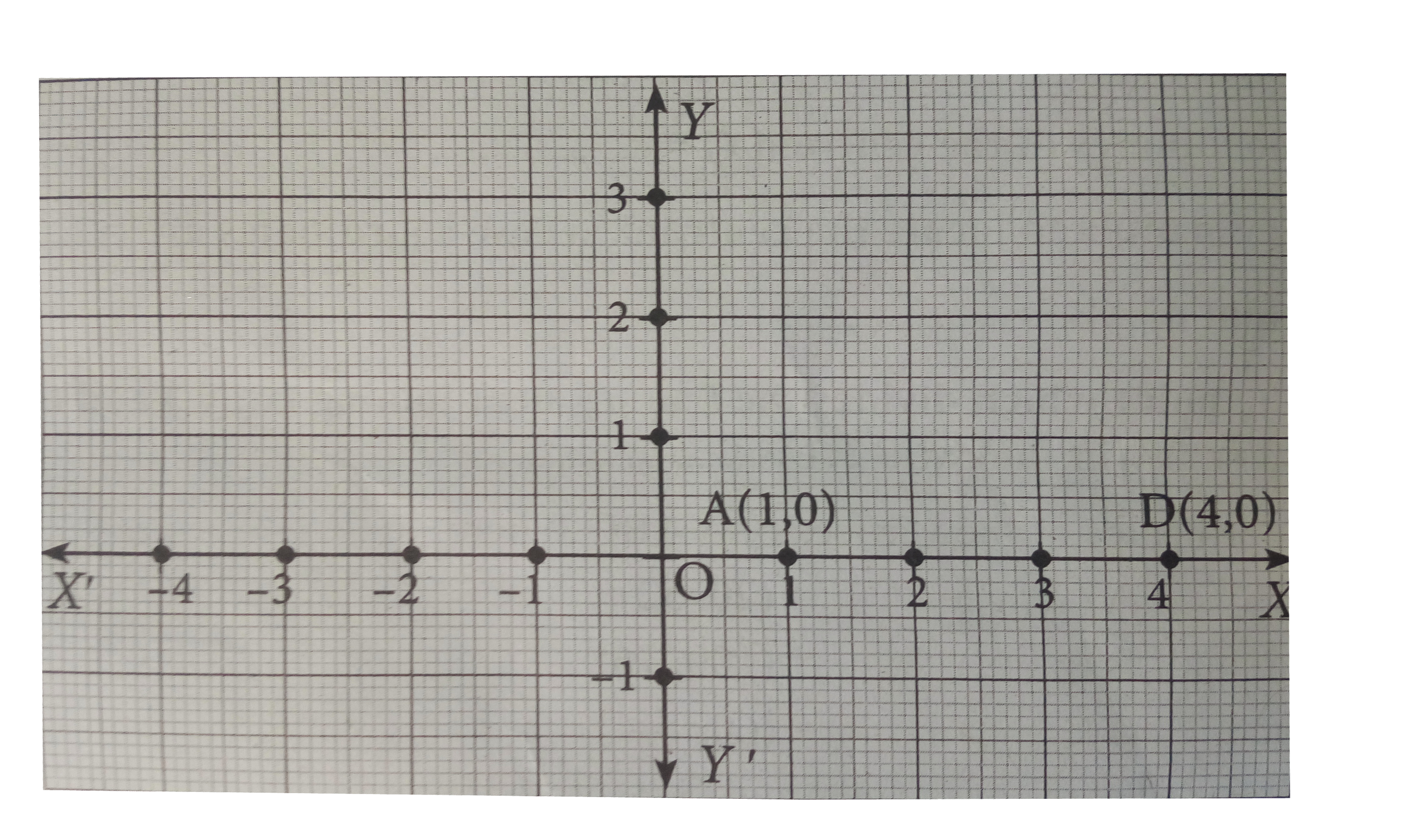
View SolutionPlot the following points on a graph sheet by taking the scale as 1cm=1 unit.
Find how far the points are from each other?
A(1,0) and D(4,0). Find AD and also DA.
Is AD=DA?
You plot another set of points and verify your Result.
View SolutionFind the area of a quadrilateral ABCD where AB = 7 cm, DA = 15 cm, AC = 9 cm, BC = 6 cm and CD = 12 cm.
View Solutionमान लीजिये रेखाखंड PQ रेखाखंड XY पर लंब है | मान लीजिए ये परस्पर बिंदु A पर प्रतिच्छेद करते हैं | ∠PAY की माप क्या है ?
View SolutionIf P is (3, 1) and Q is a point on the curve y2=8x , then the locus of the mid-point of the line segment PQ is
View SolutionIf AB is line segment and if arc at A and B intersect at P and Q resp. then PQ is
View SolutionIf line segment PQ is perpendicular bisector of a given line segment AB and, PQ intersect AB at M if length of AB is 10 cm then AM=?
View SolutionObjective : To construct a perpendicular to a line segment from as external point using paper folding. Procedure : Draw a line segment AB and mark an external point P. Move B along BA till the fold passes through P and crease it along that line. The crease thus formed is the perpendicular to AB through the external point P.
View SolutionObjective : To construct a perpendicular bisector of a line segment using paper folding
Procedure : Make a line segment on a paper by folding it and name it as PQ. Fold PQ in such a way that P falls on Q and thereby creating a creas RS. This line RS is the perpendicular bisector of PQ.
View SolutionEquation of the plane through the mid - point of the line segment joining the points P(4,5,-10) and Q (-1,2,1) and perpendicular to PQ is
View SolutionAB एक रेखाखण्ड है तथा बिन्दु P और Q इस रेखाखण्ड AB के विपरीत और इस प्रकार स्थित है कि इनमे से प्रत्येक A और B से समदूरस्थ है ( देखिए आक्रति ) दर्शाइए कि रेखा PQ रेखाखण्ड AB का लम्ब समद्धिभाजक है |
View SolutionGiven a triangle with side PQ=8 cm. To get a line segment PQ'=34 of PQ, we divide the line segment PQ in the ratio
View Solutionएक रेखा y-अक्ष और x-अक्ष को क्रमशः बिंदुओं P और Q पर प्रतिच्छेद करती है। यदि, (2,-5) रेखाखंड PQ का मध्य-बिंदु है, तो Pऔर Q के निर्देशांक क्रमशः हैं
View Solution
