Is f(x)×g(x)×r(x)=LCM[f(x),g(x),r(x)]×GCD[f(x),g(x),r(x)]?Solution in Tamil
Step by step video & image solution for Is f(x)xx g(x)xx r(x)=LCM[f(x),g(x),r(x)]xx GCD [f(x),g(x),r(x)]? by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
For system of linear equations with three variables the minimum number of equations required to get unique solution is ……
View SolutionSolve the following system of linear equations in three variables
x+y+z=5,2x−y+z=9,x−2y+3z=16
View SolutionIf the points (3,-4) (1,6) and (-2,3) are the vertices of a triangle ,find its area.
View SolutionLet f and g be two functions from R into R defined by f(x) =|x|+x and g(x)=x for all x∈R. Find f o g and g o f
View Solutionमान लीजिए कि f,g:R→R क्रमश: f(x)=x+1,g(x)=2x−3 द्वारा परिभाषित है।
(f+g)(x),(f−g)(x) और (fg)(x) ज्ञात कीजिए।
View SolutionLet f:R→R be given by f(x)=3+4x and g(x)=(f of of−−−−n times) (x) then find the range and domain of g(x)
View SolutionIf f:R→R and g:R→R are given by f(x)=|X| and g(x)=[x] for each x∈R then {x∈R:g(f(x))≤f(g(x))} is equal to
View SolutionComplete the factor tree for the given polynomials f(x) and g(x). Hence find their GCD and LCM.
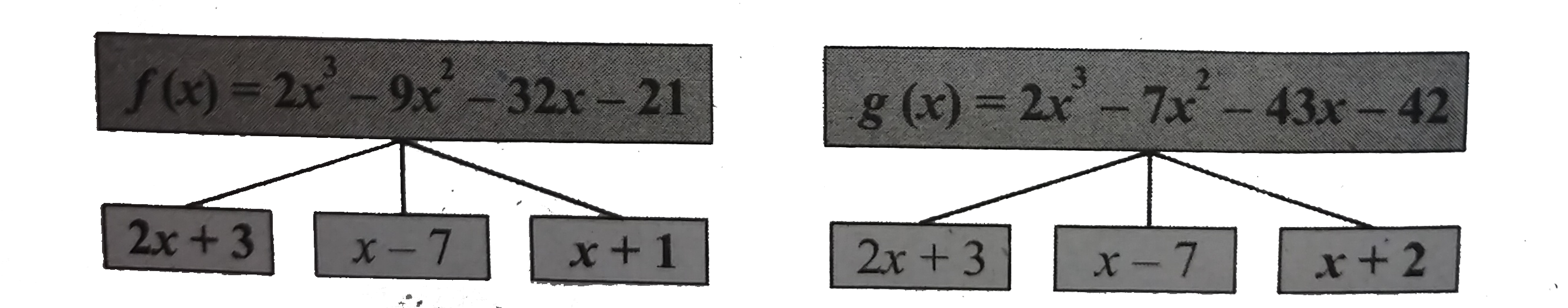
1. GCD [f(x) and g(x)] = ……………
2. LCM [f(x) and g(x)] = …………..
View SolutionIs f(x)×g(x)×r(x)=LCM[f(x),g(x),r(x)]×GCD[f(x),g(x),r(x)]?
View SolutionIf f:R→R and g:R→R defined f(x)=|x| and g(x)=[x−3] for x∈R [.] denotes the greatest integer function then {g(f(x)):−85<x<85}=
View SolutionIf f:R→R and g:R→R are defined by f(x)=x−[x] and g(x)=[x]∀x∈R,f(g(x))=
View SolutionIf f:R→R and g:R→R are given by f(x)=|x| and g(x)=[x] for each x∈R, then {x∈R:g(f(x))≤f(g(x))}=
View SolutionIf f:R→R,f(x)=x−2,g:R→R,g(x)=x+2 then (f+g)(x)= ……..
View SolutionIf f:R→R and g:R→R are given by f(x)=|x| and g(x)={x} for each x∈R, then ∣x∈R:g(f(x))≤f(g(x))}=
View SolutionLet f:R→R be defined by f(x)=3x2−5 and g:R→R by g(x)=xx2+1× Then gof is
View SolutionIf f:R→R are defined by f(x)=x−[x] and g(x)=[x] for x∈R, where [x] is the greatest integer not ex-ceeding x, then for everyx∈R,f(g(x))=
View Solution
