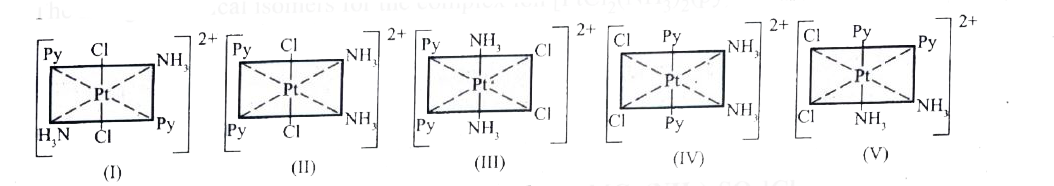
Given an example for complex of the type [Ma2b2c2] where a,b,c are monodentate ligands and give the possible isomers.
The octahedral complexes of [Ma2b2c2] type can exist in five geometrical isomers.
The five geometrical isomers for the complex ion [PtCl2(NH3)2(py)2]2+ are shown below.
Topper's Solved these Questions
- COORDINATION CHEMISTRYBOOK - FULL MARKSCHAPTER - COORDINATION CHEMISTRYEXERCISE - Additional Question(Choose the correct answer)
76 Videos
- COORDINATION CHEMISTRYBOOK - FULL MARKSCHAPTER - COORDINATION CHEMISTRYEXERCISE - Additional Question(Fill in the blanks)
64 Videos
- COORDINATION CHEMISTRYBOOK - FULL MARKSCHAPTER - COORDINATION CHEMISTRYEXERCISE - Additional Question(5 Marks Question)
26 Videos
- CHEMISTRY IN EVERYDAY LIFE BOOK - FULL MARKSCHAPTER - CHEMISTRY IN EVERYDAY LIFE EXERCISE - ADDITIONAL QUESTION ( 5 Mark Questions )
2 Videos
- ELECTRO CHEMISTRYBOOK - FULL MARKSCHAPTER - ELECTRO CHEMISTRYEXERCISE - ADDITIONAL QUESTIONS -5- MARK QUESTIONS
15 Videos
Similar Questions
Why does a tetrahedral complex of the type [MA2B2] not show geometrical isomerism?
View SolutionThe total count of geometrical isomers for a square planar complex [Mabcd]n± is 'x'. The number of geometrical isomers possible for octahedral complex of the type [Ma4b2]n± - is 'y'. Calculate x+y: _______ (a, b, c, d are monodentate ligands).
View Solution
Knowledge Check
A complex MA3X3. Where A and X are unidentate ligands may give
Atwo geometrical isomersBthree geometrical isomersCtwo geometrical isomers which can be resolved into a pair of enantiomersDtwo geometrical isomers one of which can be resolved into a pair of enantiomers .For the square planar complex [M(a) (b) (c ) (d)] (where M = central meatal and a, b, c and d are monodentate ligands), the number of possible geometrical isomers are
A1B2C3D4For the square planar complex [M(a) (b) (c ) (d)] (where M = central metal and a, b, c and d are monodentate ligands), the number of possible geometrical isomers are
AoneBtwoCthreeDfour
Find the total number of diastereomers of the complex Ma2b2c2 where M is the central metal atom/ion and a, b, c are monodentate achiral ligands.
View SolutionFind the total number of diastereomers of the complex Ma2b2c2 where M is the central metal atom/ion and a, b, c are monodentate achiral ligands.
View SolutionThe possible number of stereoismers for the formula [Ma2b2cd]±n.
View SolutionWhat will be the number of stereoisomers for complex [Ma2b2c2]nt, if its two different monodentate ligends are replaced with one symmetrical bidentate ligand
View SolutionHow many isomers are possible for an octahedral complex of the type MA2B2C2 ?
View Solution
