Decay constants of two radio-active samples A and B are 15 x and 3x respectively . They have equal number of initial nuclei . The ratio of the number of nuclei left in A and B after a time 16x is Solution in Kannada
e
e2
e−1
e−2
The correct Answer is:D
According to radioactive decay , N=N0e−λt
where
N0= Initial number of nuclei at t = 0
N = Number of nuclei left undecayed after time t
λ = Decay constant
For A , NA=N0Ae−(15x)(1/6x)
= N0Ae−5/2
For B , NB=N0Be−(3x)(1/6x)
=N0Be−1/2
As per question ,
N0A=N0B
∴NANB=e−5/2e−1/2=e−2
Topper's Solved these Questions
Similar Questions
All capacitors used in the diagram are identical and each is capacitance C .Then the effective capacitance between the points A and B is
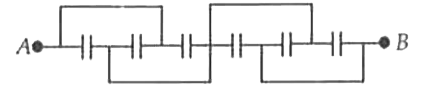 02:51View Solution
02:51View SolutionWhen an additional charge 2C is given to a capacitor, energy stored in it is increased by 21%. The original charge of the capacitor is
01:46View SolutionHalf-lives of two radioactive elements A and B are 20 minutes and 40 minutes respectively. Initially, the samples have equal number of nuclei. After 80 minutes, the ratio of decayed numbers of A and B nuclei will be
01:27View SolutionTwo radioactive materials A and B have decay constants 5lambda and lambda respectively. Initially both A and B have the same number of nuclei. The ratio of the number of nuclei of A to that of B will be 1/e , after the time x/(8lambda) then x is :
02:46View SolutionTwo radioactive nuclides A and B have decay constant 10lambda and lambda respectively. If initially they have same number of nuclei, calculate the ratio of nuclei of A and B after a time 1//9lambda
03:26View SolutionRadioactive material 'A' has decay constant '8 lamda' and material 'B' has decay constant 'lamda'. Initial they have same number of nuclei. After what time, the ratio of number of nuclei of material 'B' to that 'A' will be (1)/( e) ?
02:56View SolutionHalf lives of two radioactive nuclei A and B are 10 minutes and 20 minutes, respectively. If, initially a sample has equal number of nuclei, then after 60 minutes, the ratio of decayed numbers of nuclei A and B will be:
03:31View SolutionHalf-lives of two radioactive elements A and B are 20 minutes and 40 minutes respectively. Initially, the samples have equal number of nuclei. After 80 minutes, the ratio of decayed numbers of A and B nuclei will be :
03:31View SolutionHalf lives of two radioactive elements A and B are 20 minutes and 40 minutes respectively. Initially, the samples have equal number of nuclei. Calculate the ratio of decayed numbers of A and B nuclei after 80 minutes.
View SolutionHalf lives of two radioactive elements A and B are 20 minutes and 40 minutes respectively. Initially, the samples have equal number of nuclei. Calculate the ratio of decayed numbers of A and B nuclei after 80 minutes.
04:40View SolutionDecay constants of two radio-active samples A and B are 15x and 3x zospectively. They have equal number of initial nuclci. The ratio of the number of nuclei left in A and B after a time 16x is :
04:16View Solutionदो रेडियो सक्रिय पदार्थों X1 और X2 के क्षय स्थिरांक क्रमशः 10λ और λ हैं। यदि आरम्भ में उनके नाभिकों की संख्या समान हो , तो X1 के नाभिकों की संख्या और X2 के नाभिकों की संख्या का अनुपात 1/e, कितने समय के बाद होगा
01:59View SolutionThe decay constants of two radioactive samples are 15x and 3x. If initially they have the same number of nuclei. Then, (1)/(6x) The ratio of the number of nuclei after time will be
04:15View SolutionTwo radioactive materials A and B have decay constants 10lambda and lambda , respectively. If initially they have the same number of nuclei, then the ratio of the number of nuclei of A of that of B will be 1//e after a time :
View SolutionHalf lives of two radioactive nuclei A and B are 10 minutes and 20 minutes respectively, If initially a sample has equal number of nuclei, then after 60 minutes, the ratio of decayed numbers of nuclei A and B will be :
03:57View Solution

