Find the de-Broglie wavelength of an electron with kinetic energy of 120 eV.Solution in Kannada
102 pm
124 pm
95 pm
112 pm
The correct Answer is:D
The de - Brogile wavelength of an electron is
λ=h√2mK
where h is the Planck's constant , m is mass of the electron and K is its Kinetcic energy .
Here ,
h=6.63×10−34Js
m=9.1×10−31kg
K=120eV=120×1.6×1019J
(∵2eV=1.6×10−19J)
∴λ=6.63×10−34Js√2(9.1×10−31kg)(120×1.6×10−19J)
=1.12×10−10m=112×10−12m
112 pm (∵1pm=10−12m)
Topper's Solved these Questions
Similar Questions
A solenoid length 0.4cm, radius 1 cm and 400 turns of wire. If a current of 5 A is passed through this solenoid, what is the magnetic field inside the solenoid ?
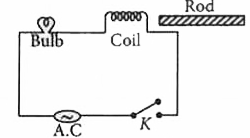
View SolutionIn the A. C. circuit shown, keeping 'K' pressed, if an iron rod is inserted into the coil, the bulb in the circuit
View Solution100 eV गतिज ऊर्जा के इलेक्ट्रॉन की दी-ब्रोगली तरंगदैर्घ्य ज्ञात कीजिए ।
View Solution400 eV गतिज ऊर्जा वाले इलेक्ट्रान की डी ब्रोग्ली तरंगदैर्ध्य ज्ञात कीजिये
View SolutionWhat is the Be Broglie wavelength of an electron with kinetic energy of 120 eV.
View SolutionAn electron is having a kinetic energy of 50 eV. Its de broglie wavelength is
View SolutionFind the de-Broglie wavelength of an electron with kinetic energy of 120 eV.
View SolutionFind the de-Broglie wavelength of an electron with kinetic energy of 120 eV.
View SolutionCalculate the de Broglie wavelength of an electron of kinetic energy 500 eV.
View Solutionde-Broglie wavelength associated with an electron having kinetic energy 500 eV is :
View SolutionFind the de-Broglie wavelength of an electron with kinetic energy of 120 eV.
View SolutionThe de broglie wavelength of electron moving with kinetic energy of 144 eV is nearly
View SolutionDe Broglie proposed the wave nature of electrons suggesting matter waves.
Find the momentum, speed and De-Broglie wavelength of an electron with Kinetic energy of 120 eV.
View Solutionएक इलेक्ट्रॉन की जिसकी गतिज ऊर्जा 120 eV है, डी-ब्रोग्ली तरंग दैर्ध्य होगी
View SolutionWhat is the
(a)Momentum
(b)speed ,and
(c )de-Broglie wavelength of an electron with kinetic energy of 120 eV.
View Solution
