For any three sets A,B C, prove that :
n(A∪B∪C)=[n(A)+n(B)+n(C)+n(A∩B∩C)]−[n(A∩B)+n(B∩C)+n(A∩C)].
Step by step video & image solution for For any three sets A,B C, prove that : n(AuuBuuC)=[n(A)+n(B)+n(C)+n(AcapBcapC)]-[n(AcapB)+n(BcapC)+n(AcapC)]. by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - SETS EXERCISE - OBJECTIVE TYPE QUESTIONS (A )(MULTIPLE CHOICE QUESTIONS)30videos
SETS
- BOOK - MODERN PUBLICATIONCHAPTER - SETS EXERCISE - OBJECTIVE TYPE QUESTIONS (B) (FILL IN THE BLANKS )10videos
SETS
- BOOK - MODERN PUBLICATIONCHAPTER - SETS EXERCISE - Exercise8videos
SETS
- BOOK - MODERN PUBLICATIONCHAPTER - SEQUENCES AND SERIESEXERCISE - CHAPTER TEST12videos
SEQUENCES AND SERIES
- BOOK - MODERN PUBLICATIONCHAPTER - STATISTICSEXERCISE - Chapter Test7videos
STATISTICS
Similar Questions
Given that n(A)=25,n(B)=15,n(A∪B)=10. Determine n(A∩B)
View SolutionIf A , B and C are three non - empty finite sets such that n (A) =19 , n (B) = 15 , n (C ) =17, n(∩B)=11,n(B∩C)=6,n(C∩A)=7andn(A∩B∩C)=5. Also n (U) =50.
(i) n(A∩Bc∩Cc)
(ii) n(B∩Cc∩Ac)
(iii) n(C∩Ac∩Bc)
(iv) n(C∩B∩Cc)
(v) n(B∩C∩Ac)
(vi) n(C∩A∩Bc)
(vii) n(A∪B∪C)
(viii) n((A∪B∪C)c).View Solution
Knowledge Check
- Question 1 - Select One
If A,B,C are subsets of the universal set U such that n(U)=692,n(B)=230,n(C)=370,n(B∩C)=20,n(A'∩B'∩C')=10 then: n(A∩B'∩C')=
A876B784C172Dnone of these. - Question 2 - Select One
If A and B are subsets of the universal set E such that n(E)=50,n(A)=35,n(B)=22andn(A'∩B')=3, then: n(A∩B)andn(A∪B) are respectively
A(47,10)B(45,25)C(12,47)Dnone of these. - Question 3 - Select One
If n(A)=3,n(B)=5andn(A∩B)=2, then: n(A×B)=
A6B36C15Dnone of these.
If A and B are two non empty sets the prove the following1.n(A∪B)=n(A)+n(B)−n(A∩B). 2.nA∪B∪C)=n(A)+n(B)+n(C)−n(A∩B)−n(B∩C)−n(C∩a)+n(A∩B∩C)
View SolutionIf A and B are two sets such that n(A)=12, n(B)=19 and (A∪B)=26 then find.
(a) n(A∩B)
(b) n(A−B)
(c) n(B−A)View SolutionLet A and B be two sets such that :
(i) n(A)=20,n(A∪B)=42andn(A∩B)=4
(ii) n(A)=17,n(A∪B)=38andn(A∩B)=2. Find n (B) , n (A-B) and n (B-A).View SolutionIf n (A)=n(B) , find n (A∩B)andn(A∪B) use the fig
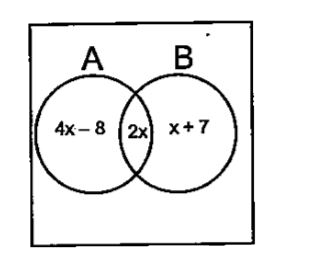 View Solution
View SolutionIf n(A∩B)=51,n(A)=20,n(B)=44,find n(A∪B).
View Solution
