If the highway overpass is represented by x2−2x−8.Then its zeroes are (i) (2,-4) (ii) (4,-2) (iii) (-2,-2) (iv) (-4,-4)
Step by step video & image solution for If the highway overpass is represented by x^2-2x-8.Then its zeroes are (i) (2,-4) (ii) (4,-2) (iii) (-2,-2) (iv) (-4,-4) by Physics experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SAMPLE PAPER (MATHEMATICS STANDARD)BOOK - CBSE MODEL PAPERCHAPTER - SAMPLE PAPER (MATHEMATICS STANDARD)EXERCISE - PART - B
16 Videos
- QUESTION BANK 2021BOOK - CBSE MODEL PAPERCHAPTER - QUESTION BANK 2021EXERCISE - QUESTIONS
60 Videos
- SAMPLE PAPER 2022BOOK - CBSE MODEL PAPERCHAPTER - SAMPLE PAPER 2022EXERCISE - ALTERNATIVE QUESTIONS(SECTION C)
4 Videos
Similar Questions
(i) x4 (ii) x−3 (iii) 3x2
View SolutionCase Study Based- 3
Applications of Parabolas-Highway Overpasses/Underpasses A highway underpass is parabolic in shape.

Parabola
A parabola is the graph that results from p(x)=ax2+bx+c
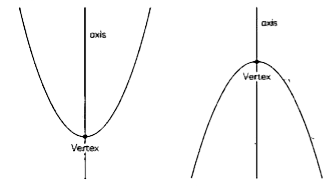
Parabolas are symmetric about a vertical line known as the Axis of Symmetry. The Axis of Symmetry runs through the maximum or minimum point of the parabola which is called the Vertex.
If the highway overpass is represented by x2−2x−8. Then its zeroes are
View SolutionIn which quadrant does the given points lie
(i) (4,−2)
(ii) (−2,−2)
(iii) (4,3)
(iv) (−3,4)
View SolutionThe vertex of the parabola x2+8x+12y+4=0 is (i) (−4,1) (ii)(4,−1) (iii)(−4,−1)
View SolutionLocate the following points
(i) (1,-1,3) (ii) (-1,2,4)
(iii) (-2,-4,-7) (iv) (-4,2,-5)
View SolutionThe representation of Highway Underpass whose one zero is 6 and sum of the zeroes is 0, is
(i) x2-6x+2
(ii)x2-36
(iii)x2−6
(iv) x2−3
View SolutionFind the position of the following points with respect to the parabola y2=16x
(i) (4,-8) , (ii) (2,4)
(iii) (0,1) , (iv) (-2,8)
View SolutionFactorize
(i)(x4+4)
(ii)(x2+4x2)
(iii)(x4+1x4+1)
View SolutionCheck which of the following are solutions of the equations x−2y=4and which are not:
(i) (0,2) (ii) (2,0) (iii) (4,0)
(iv) (√2,4√2) (v) (1, 1)
View SolutionFactorize (i)(x4+3x2+4)
(ii)(x4+5x2+9)
View Solution
