A circle centred at O has radius 1 and contains the point A. Segment AB is tangent to the circle at A and angle AOB=θ.If point C lies on OA and BC bisects the angle ABO then OC equals
Step by step video & image solution for A circle centred at O has radius 1 and contains the point A. Segment AB is tangent to the circle at A and angle AOB=theta.If point C lies on OA and BC bisects the angle ABO then OC equals by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
A circle centred at O has radius 1 and contains the point A. Segment AB is tangent to the circle at A and angle AOB=θ.If point C lies on OA and BC bisects the angle ABO then OC equals
View SolutionA circle centred at O has radius 1 and contains the point A. Segment AB is tangent to the circle at A and angle AOB=θ.If point C lies on OA and BC bisects the angle ABO then OC equals
View Solution
Knowledge Check
On the circle with centre O, points A, B are such that OA =AB. A point C is located on the tangent at B to the circle such that A and C are on the opposite sides of the line OB and Ab =BC. The line segment AC intersects the circle again at F. Then the ratio ∠BOF:∠BOC is equal to-
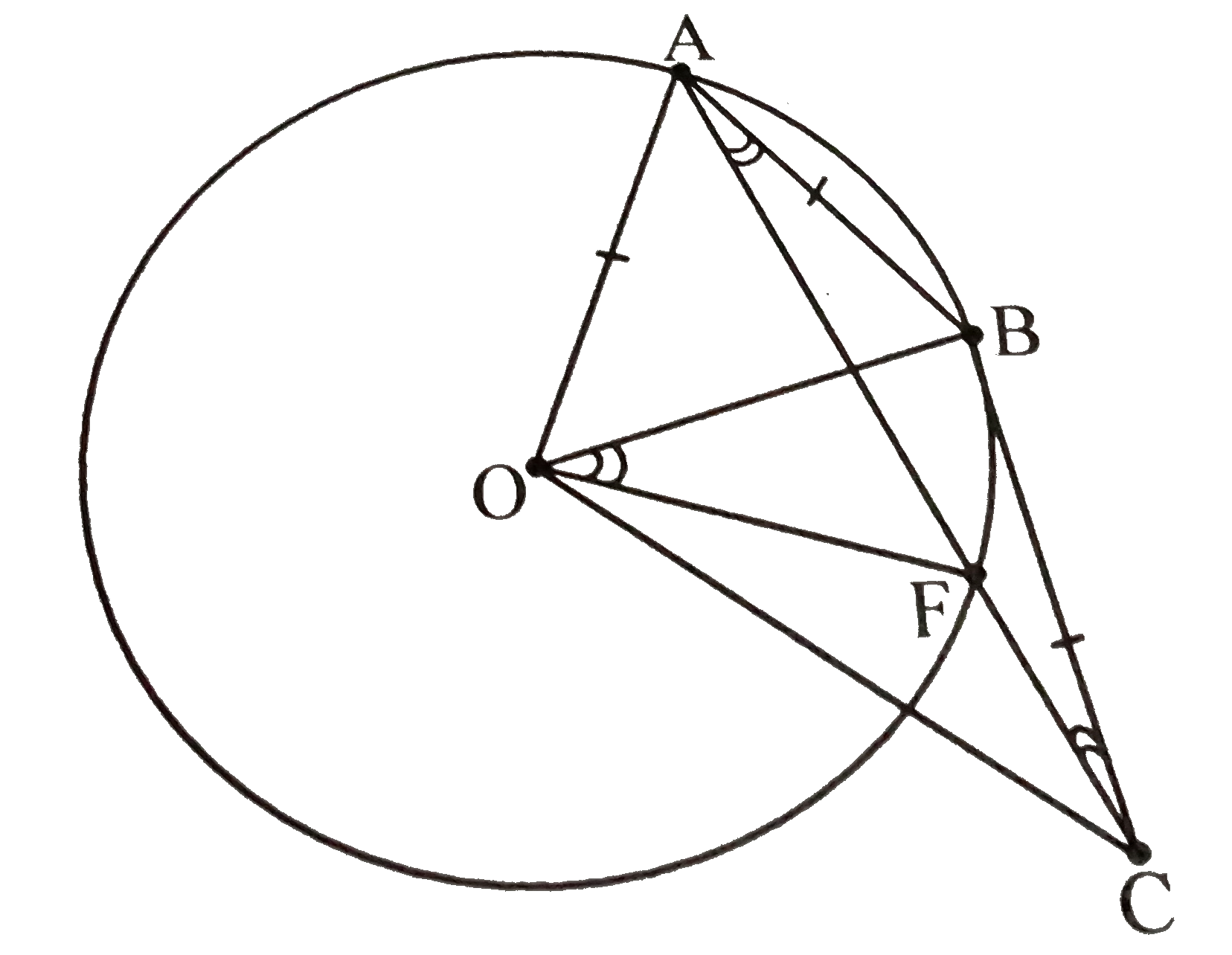 A1:2B2:3C3:4D4:5
A1:2B2:3C3:4D4:5In a circle with the centre is O and AB is the diameter of the circle and CD is the chord of the circle. If angle ABC is 54∘ and the length of CD and BC is equal, then find the ∠DBC.
A1. 36∘B2. 24∘C3. 48∘D4. 12∘
A circle centred at 'O' has radius 1 and contains the point A. Segment AB is tangent to the circle at A and ∠AOB=θ. If point C lies on OA and BC bisects the angle ABO then OC equals
View SolutionA circle centred at 'O' has radius 1 and contains the point A. Segment AB is tangent to the circle at A and ∠AOB=θ. If point C lies on OA and BC bisects the angle ABO then OC equals
View SolutionIn figure PQ is tangent to the circle with centre at O at the point B , if ∠AOB=100∘, then ∠ABP is equal to

View SolutionIn figure PQ is tangent to the circle with centre at O at the point B , if ∠AOB=100∘, then ∠ABP is equal to

View SolutionIf Fig , AOB is diameter of a circle with centre O and AC is a tangent to the circle at A . If ∠BOC=130∘ , then find ∠ACO .

View SolutionIn the given, figure , O is the centre of the circle and AD is a tangent to the circle at.A if ∠CAD=55∘ and ∠ADC=25∘, then find ∠ABO.
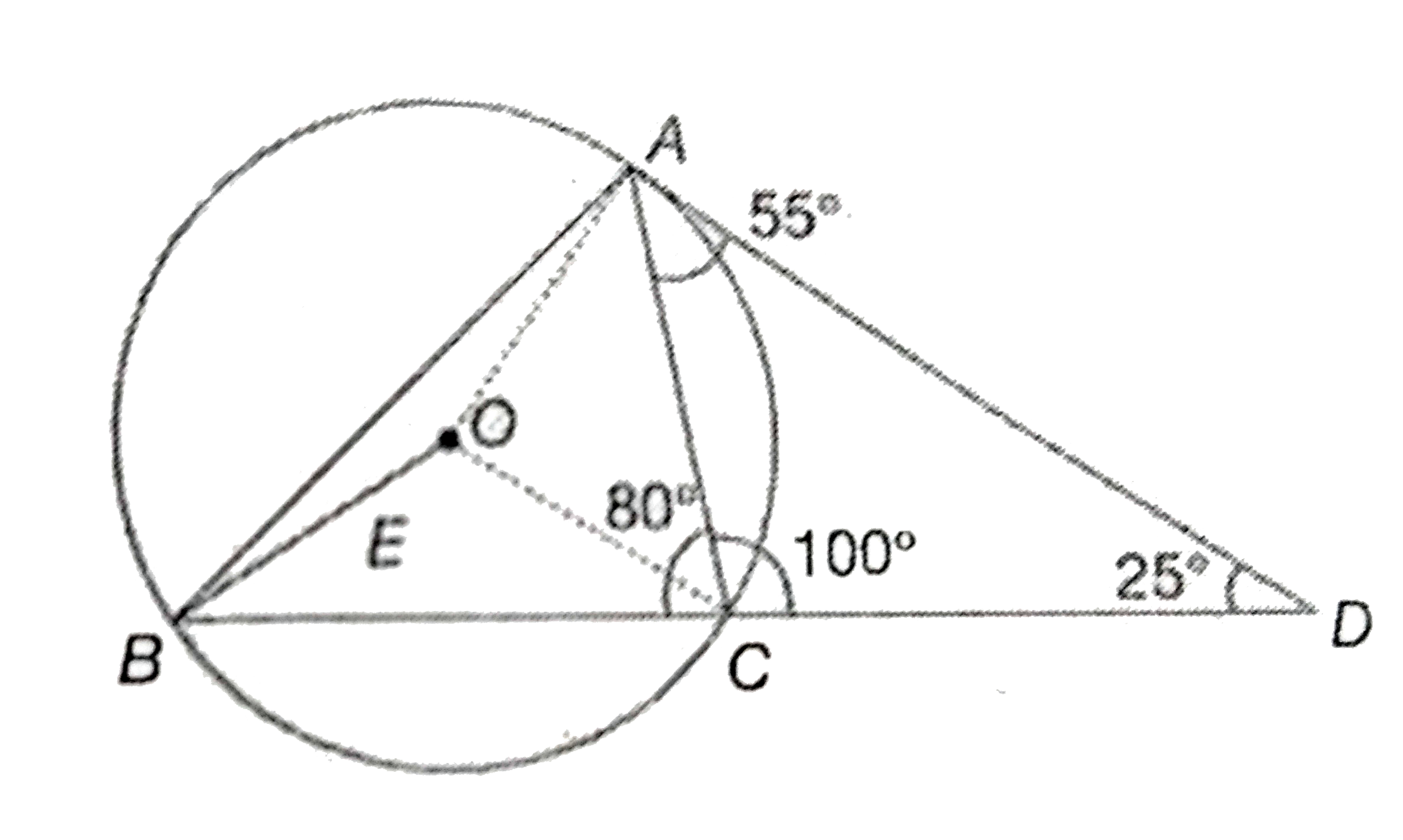
View Solution
