Assertion: A sphere can perform pure rolling on a amooth surface.
Reason: When a sphere performs pure rolling on sufficiently rough surface then acceleration of point of contact is not equal to zero.
If both assertion and reason are correct and reason is the correct explanation of the assertion.
If both assertion and reason are correct but reason is not the correct explanation of assertion
If assertion is correct but reason is incorrect.
If assertion is incorrect but reason is correct
The correct Answer is:B
Here both the statements are correct but do not form assertion reason pair. If we initially set the condition (v=rω) of pure rolling and put the sphere on smooth surface then it will maintain the condition of pure rolling.
When sphere performs pure rolling on surface then tangential acceleration of point of contact becomes zero but there is radial acceleration (rω2) towards the centre.
Topper's Solved these Questions
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - COMPETITION FILE ( OBJECTIVE TYPE QUESTIONS (MATCHING TYPE QUESTIONS) )
3 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - COMPETITION FILE (OBJECTIVE TYPE QUESTIONS (MATRIX MATCH TYPE QUESTIONS) )
1 Video
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - COMPETITION FILE ( OBJECTIVE TYPE QUESTIONS MULTIPLE CHOICE QUESTIONS (with more than one correct answer) )
35 Videos
- PHYSICAL WORLD BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)
6 Videos
- THERMAL PROPERTIES OF MATTERBOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - CHAPTER PRACTICE TEST
15 Videos
Similar Questions
Knowledge Check
Sphere in pure accelerated rolling
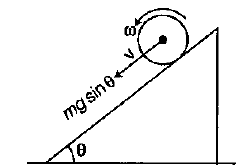 AThe direction of fs is upwardsBThe direction of fs is downwardsCThe direction of gravitational force Is upwardsDThe direction of normal reaction Is downwards
AThe direction of fs is upwardsBThe direction of fs is downwardsCThe direction of gravitational force Is upwardsDThe direction of normal reaction Is downwardsA ring performs pure rolling on a horizontal surface and its state at some instant of time is shown in figure.
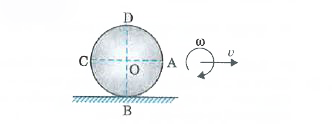 AK.E. stored in the portion AB is same as K.E. stored in the portion CDBK.E. stored in the portion AB is same as KE. stored in the portion BCCK.E. stored in the portion ABC is same as K.E. stored in the portion CDADK.E. stored in the portion ABC is less than K.E. stored in the portion CDA
AK.E. stored in the portion AB is same as K.E. stored in the portion CDBK.E. stored in the portion AB is same as KE. stored in the portion BCCK.E. stored in the portion ABC is same as K.E. stored in the portion CDADK.E. stored in the portion ABC is less than K.E. stored in the portion CDAA ring performs pure rolling on a horizontal fixed surface with its centre moving with speed v.
AMaximum possible speed that any particle of ring may have is v/√2BMinimum possible speed that any particle of ring may have is v/√2Cparticles on the ring may have speed anywhere between 0 and 2u.DNone of these
Assertion : A solid sphere cannot roll without slipping on smooth horizontal surface
Reason : If the sphere is left free on smooth inclined surface. It cannot roll without slipping.
View SolutionA solid sphere is in pure rolling motion on an inclined surface having inclination θ
View SolutionA solid sphere is in pure rolling motion on an inclined surface having inclination θ

View SolutionAssertion : A solid sphere cannot roll without slipping on smooth horizontal surface
Reason : If the sphere is left free on smooth inclined surface. It cannot roll without slipping.
View SolutionA sphere is performing pure rolling on a rough horizontal surface with constant angular velocity. Frictional force acting on the sphere is zero.
Velocity of contact point is zero.
View Solution
