A tank with a square base of area is divided by a vertical parition in the middle. The bottom of the partition has a small hinged door of area . The tank is filled with water and an acid (of relative density 1.7) in the other, both to a height of . Compute to force necessary the force nec cessary to keep the door closed.
Force on the door
For acid
For water,
Pressure exerted by the acid,
Difference of pressure
Area of door,
Force applied on the door
=54.88 N
To keep the door closed a force equal to 54.88 N should be applied from water compartment to acid compartment
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDSBook:MODERN PUBLICATIONChapter:MECHANICAL PROPERTIES OF FLUIDSExercise:NCERT File (Exemplar Problems (Objective Questions))
Explore 5 Videos
- MECHANICAL PROPERTIES OF FLUIDSBook:MODERN PUBLICATIONChapter:MECHANICAL PROPERTIES OF FLUIDSExercise:NCERT File (MCQ)
Explore 5 Videos
- MECHANICAL PROPERTIES OF FLUIDSBook:MODERN PUBLICATIONChapter:MECHANICAL PROPERTIES OF FLUIDSExercise:NCERT (Textbook Exercises)
Explore 20 Videos
- MATHEMATICAL TOOLSBook:MODERN PUBLICATIONChapter:MATHEMATICAL TOOLSExercise:PRACTICE PROBLEMS (10)
Explore 12 Videos
- MOTION IN A PLANE Book:MODERN PUBLICATIONChapter:MOTION IN A PLANE Exercise:Chapter Practice Test
Explore 15 Videos
Similar Questions
A wooden plank of length 1m and uniform cross-section is hinged at one end of the bottom of tank as shown in figure. The tank is filled with water upto a height of 0.5m. Find the angle θ that the plank makes with the vertical in the equilibrium position. [Exclude the case thea=0]
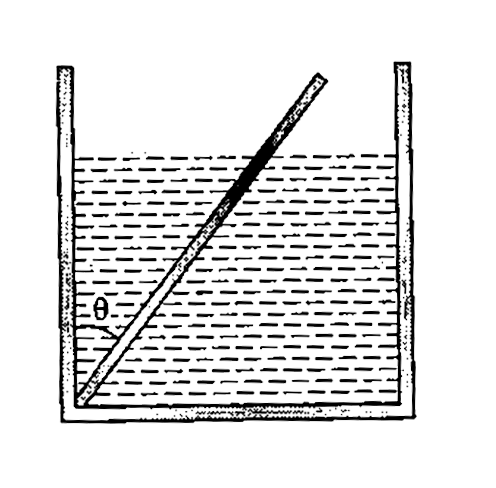
View SolutionWater flows into a large tank with flat bottom at the rate of 10−4m3s−1. Water is also leaking out of a hole of area 1 cm2 at its bottom. If the height of the water in the tank remains steady, then this height is :
View Solution
Knowledge Check
A tank with a square base of area 2m2 is divided into two compartments by a vertical partition in the middle. There is a small hinged door of face area 20cm2 at the bottom of the partition. Water is filled in one compartment and an acid of relative density 1.5 in the other, both to a height of 4m. The force necessary to keep the door closed is (Takeg=10ms−2)
A10 NB20 NC40 ND80 NThe force does water exert on the base of a house tank of base area 1.5m2 when it is filled with water up to a height of 1 m is (g=10ms−2)
A1200 kgwtB1500 kgwtC1700 kgwtD2000 kgwtA tank with vertical walls is monted so that its base is at height of 1.2 m above the horizotnal ground. The tank is filled with water to depth 2.8 m. A holw is punched in the side wall of the tank at a depth x m below the surface of water to have maximum range of the emerging stream. then the value of x in metre is
A4B1.6C2D2.3
A rectangular tank of base area 100 m2 has a height of 2 m. Calculate the thrust at the bottom of the tank, if it is filled upto the brim with water of density 103 kg m−3.
View SolutionThe wooden plank of length 1 m and uniform cross section is hinged at one end to the bottom of a tank as shown in figure. The tank is filled with water up to a height of 0.5 m. The specific gravity of the plank is 0.5. Find the angle θ that the plank makes with the vertical in the equilibrium position. (Exclude the case θ=0)
 ,
,
View SolutionThe level of water in a tank is 5m high.A hole of area 1cm^2 is made in the bottom of the tank. The rate of leakage of water from the hole is (g=10ms^(-2)
View Solution_S01_053_Q01.png)
A large cylinderical tank of cross-sectional area 1m2 is filled with water . It has a small hole at a height of 1 m from the bottom. A movable piston of mass 5 kg is fitted on the top of the tank such that it can slide in the tank freely. A load of 45 kg is applied on the top of water by piston, as shown in figure. The value of v when piston is 7 m above the bottom is (g=10m/s2)
(a). √120m/s
(b). 10m/s
(c). 1m/s
(d). 11m/s
View SolutionWater is filled in a rectangular tank of size 3m×2m×1m. a. Find the total force exerted by the water on the bottom surface of the tank. b. cosider a vertical side of area 2m×1m. Take a horizontal strip of wide δx metre in this side, situated at a depth of x metre from the surface of water. Find the torque of the force calculate in part b. about the bottom edge of this side. e. Find the total torque by the water on the side about the bottom edge. neglect the atmospheric pressure and take =10ms−2.
View Solution