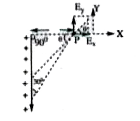
Calculate electric field intensity at point P due to a thin positive uniformly charged rod . Point P is at perpendicular distance d from the rod . The lower and upper ends of the rod make the angles α and β as shown below .

Refer the given figure . Let us select one segment of length dy at a distance y from O. Electric field due to this segment is d→E as shown. We have λ as linear charge density .So the charge on segment is λdy .
dE=14πε0λdyd2+y2
The direction of d→E is dissimilar for different locations of the segment . Hence d→E cannot be integrated directly. So, we shall integrate components perpendicular and parallel to rod separately .
Ex=∫dEcosθ=λ4πε0∫dyd2+y2cosθ
Ey=∫dEsinθ=λ4πε0∫dyd2+y2sinθ
To solve above integrations, let us assume :
y=dtanθ
dy=dsec2θdθ
d2+y2=d2sec2θ
dyd2+y2=dθd
On substituting ,
Ex=∫dEcosθ−λ4πε0d∫β−αcosθd0
Ey=∫dEsinθ=λ4πε0d∫β−αsinθdθ
In the above integration , the limits are taken from θ=−α corresponding to lower end of rod . And θ=β for the upper end of the rod .
On solving both, we get the following :
Ex=∫dEcosθ=λ4πε0d∫β−αcosθdθ=λ4πε0d[sinθ]β−α
=λ4πε0d[sinβ−sin(−α)]
Ey=∫dEsinθ=λ4πε0d∫β−αsinθdθ=λ4πε0d[−cosθ]β−α
=λ4πε0d[(−cosβ)−(−cos(−α))]
Ex=λ4πε0d(sinα+sinβ)Ex represents the component, perpendicular to rod in the direction away from the rod for λ>0.Ey=λ4πε0d(cosα−cosβ)
Ey represents component that is parallel to the rod . The direction of Ey can be upwards or downwards depending on the values of α and β.
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Practice Problems
88 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Conceptual Questions
51 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - CHAPTER PRACTICE TEST
15 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
14 Videos
Similar Questions
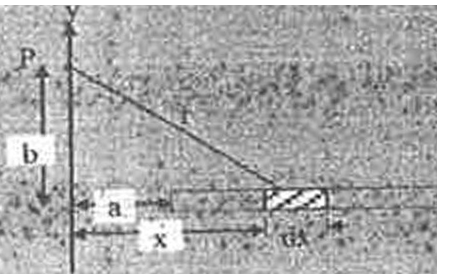
Calculate the potential due to a thin uniformly charged rod of length L at the poiot P shown in figure. The lioear charge density of the rod is λC/m

View SolutionFind the electric field at a point P on the perpendicular bisector of a uniformly charged rod. The length of the rod is L, the charge on it is Q and the distance of P from the centre of the rod is a.
View Solution
Knowledge Check
The expression for electric field intensity at a points outside uniformly charged thin plane sheet is (where, d is the distance of point from plane sheet)
Aindependent of dBdirectly proportional to √dCdirectly proportional to dDdirectly proportional to 1√dThe direction (θ) of →E at point P due to uniformly charged finite rod will be
 AAt an angle 30∘ from the x-axisB45∘ from the x-axisC60∘ from the x-axisDnone of these
AAt an angle 30∘ from the x-axisB45∘ from the x-axisC60∘ from the x-axisDnone of these
Find the electric field at a point P on the perpendicular bisector of a uniformly charged rod. The lengthof the rod is L, the charge on it is Q and the distance of P from the centre of the rod is a.
View SolutionThe direction (q) of →E at point p due to uniformly charged rod will be
View SolutionThe direction (θ) of →E at point P due to uniformly charged finite rod will be

View SolutionFind the electric field at a point P on the perpendicular bisector of a uniformly charged rod. The length of the rod is L, the charge on it is Q and the distance of P from the centre of the rod is a.
View SolutionFind : (i)Electric field intensity due to an infinitely long uniformly charged, straight conductor , at a perpendicular distance d from rod.
(ii)Electric field intensity at a point on perpendicular bisector of uniformly charged rod of length l and charge Q at a distance d from the centre of rod.
View SolutionCalculate the potential due to a thin charged rod of length L at the points along and perpendicular to the length. Charge per unit length on the rod is λ.
View Solution
