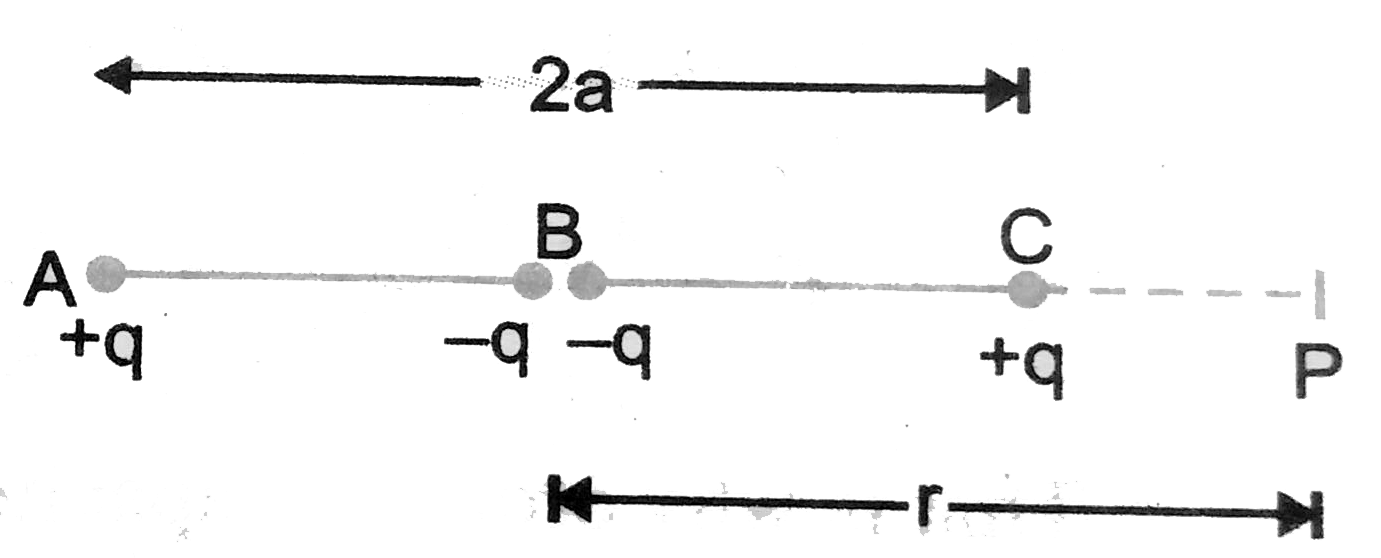
The figure given below shows a charge array known as an 'electric quadrupole'. For a point on the axis of the quadrupole, obtain the dependence of potential on r for ra>>1. Contrast your result with that due to an electric dipole and an electric monopole (i.e. a single charge).

As we know that the electric potential at a point due to a system of n charges is given by:
V=14πε0∑qnrn
Electric potential at point P due to the given charge array can be calculated as:
V=14πε0(qr+a+2×−qr+qr−a)=14πε0(2qa2r(r2−a2))
⇒V=14πε0(2qa2r(r2−a2))=14πε0⎛⎜
⎜⎝2qa2r3(1−a2r2)⎞⎟
⎟⎠
As, ra>>1,⇒V=14πε0(2qa2r3)
Thus, V∝1r3
For a monopole (single charge) electric potential varies as: V∝1r
For large values of r electric potential due to an electric dipole varies as V∝1r2 and that due to quadrapole as V∝1r3
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - NCERT FILE Solved (Additional Exercises) (Answer carefully)
7 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - NCERT FILE Solved (Additional Exercises) (Answer the following)
4 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - NCERT FILE Solved (Textbook Exercise)
11 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
- MAGNETISM AND MATTERBOOK - MODERN PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
16 Videos
Similar Questions
Figure shows a charge array known as an 'electric quadrupole'. For a point on the axis of the quadrupole, obtain the dependence of potential on r for ra>>1, and contrast your results with that due to an electric dipole, and an electric monopole (i.e., a single charge).

View SolutionFig shows a charge array known as an 'electric quadrupole'. For a point on the axis of the quadrupole, obtain the dependence of potential on r for r/a>>1, and contract your results with that due to an electric dipole and an electric monopole (i.e, a single charge).
View Solution
Knowledge Check
The electric potential at a point on the axis of an electric dipole depends on the distance r of the point from the dipole as
A∝1rB∝1r2C∝rD∝1r3The electric potential V at a point on the axis of an electric dipole depends on the distance 'r of the point from the dipole as
AV∝1rBV∝1r2CV∝1r3DV∝rElectric potential (ϕ) of a quadrupole varies with distance 'r' on its axis as :-
Aϕ∞r−1Bϕ∞r−2Cϕ∞r−3Dϕ∞r−3/2
Fig shows a charge array known as an 'electric quadrupole'. For a point on the axis of the quadrupole, obtain the dependence of potential on r for r/a>>1, and contract your results with that due to an electric dipole and an electric monopole (i.e, a single charge).

View SolutionFigure shows a charge array known as an "electric quadrupole". For a point on the axis of the quadruple, the dependence of potential on r(ra>>1) is 1rn. Find n?
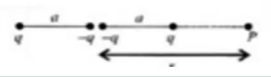
View SolutionFigure shows a charge array known as an "electric quadrupole". For a point on the axis of the quadruple, the dependence of potential on r(ra>>1) is 1rn. Find n?

View SolutionDeduce an expression for the electric potential due to an electric dipole at any point on its axis. Mention one contrasting feature of electric potential of a dipole at a point as compared to that due to a single charge.
View SolutionDeduce an expression for the electric potential due to an electric dipole at any point on its axis. Mention one contrasting feature of electric potential at a point due to dipole as compared to that due to a single charge.
View Solution
