(i) On what factors does the capacitance of a capacitor depend?
(ii) Two capacitors of capacitances 20μF and 60μF are connected in series. If the potential difference between the two eneds of the combination is 40 volt, calculate the terminal potential difference of each capacitor.
Step-by-Step Solution
1. Size and Shape of the Conducting Plates:
- The capacitance of a capacitor is directly proportional to the area of the plates. Larger plate areas allow for more charge to be stored, thus increasing capacitance.
- The distance between the plates also affects capacitance. The closer the plates are to each other, the higher the capacitance, as the electric field strength increases.
2. Dielectric Material:
- The type of dielectric material placed between the plates influences capacitance. Different materials have different dielectric constants (relative permittivity), which affect the ability of the capacitor to store charge. A higher dielectric constant results in higher capacitance.
(ii) Calculation of Terminal Potential Difference of Each Capacitor:
1. Identify the Given Values:
- Capacitance of Capacitor 1 (C1) = 20 μF
- Capacitance of Capacitor 2 (C2) = 60 μF
- Total potential difference (V_total) = 40 V
2. Formula for Capacitors in Series:
- For capacitors in series, the total capacitance (C_total) can be calculated using the formula:
1Ctotal=1C1+1C2
3. Calculate Total Capacitance:
1Ctotal=120μF+160μF
- Finding a common denominator (60):
1Ctotal=360+160=460
- Thus,
Ctotal=604=15μF
4. Calculate Total Charge (Q):
- The total charge stored in the series combination can be calculated using:
Q=Ctotal×Vtotal=15μF×40V=600μC
5. Calculate Individual Voltages (V1 and V2):
- For Capacitor 1 (C1):
V1=QC1=600μC20μF=30V
- For Capacitor 2 (C2):
V2=QC2=600μC60μF=10V
6. Final Results:
- The terminal potential difference across Capacitor 1 (C1) is 30 V.
- The terminal potential difference across Capacitor 2 (C2) is 10 V.
- The capacitance of a capacitor is directly proportional to the area of the plates. Larger plate areas allow for more charge to be stored, thus increasing capacitance.
- The distance between the plates also affects capacitance. The closer the plates are to each other, the higher the capacitance, as the electric field strength increases.
- The type of dielectric material placed between the plates influences capacitance. Different materials have different dielectric constants (relative permittivity), which affect the ability of the capacitor to store charge. A higher dielectric constant results in higher capacitance.
- Capacitance of Capacitor 1 (C1) = 20 μF
- Capacitance of Capacitor 2 (C2) = 60 μF
- Total potential difference (V_total) = 40 V
- For capacitors in series, the total capacitance (C_total) can be calculated using the formula:
1Ctotal=1C1+1C2
1Ctotal=120μF+160μF
- Finding a common denominator (60):
1Ctotal=360+160=460
- Thus,
Ctotal=604=15μF
- The total charge stored in the series combination can be calculated using:
Q=Ctotal×Vtotal=15μF×40V=600μC
- For Capacitor 1 (C1):
V1=QC1=600μC20μF=30V
- For Capacitor 2 (C2):
V2=QC2=600μC60μF=10V
- The terminal potential difference across Capacitor 1 (C1) is 30 V.
- The terminal potential difference across Capacitor 2 (C2) is 10 V.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Revision Exercises (Long Answer Questions)
13 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Revision Exercises (Numerical Problems)
25 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Revision Exercises (Fill in the Blanks)
11 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
- MAGNETISM AND MATTERBOOK - MODERN PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
16 Videos
Similar Questions
Knowledge Check
Three capacitors of capacitance 1μF,2μF and 3μF are connected in series and a potential difference of 11V is applied across the combination. Then, the potential difference across the plates of 1μF capacitor is
A2VB4VC1VD6VTwo capacitors A and B of capacitances 2μF and 5μF are connected to two battery as shown in figure The potential difference in volt between the plate of A is
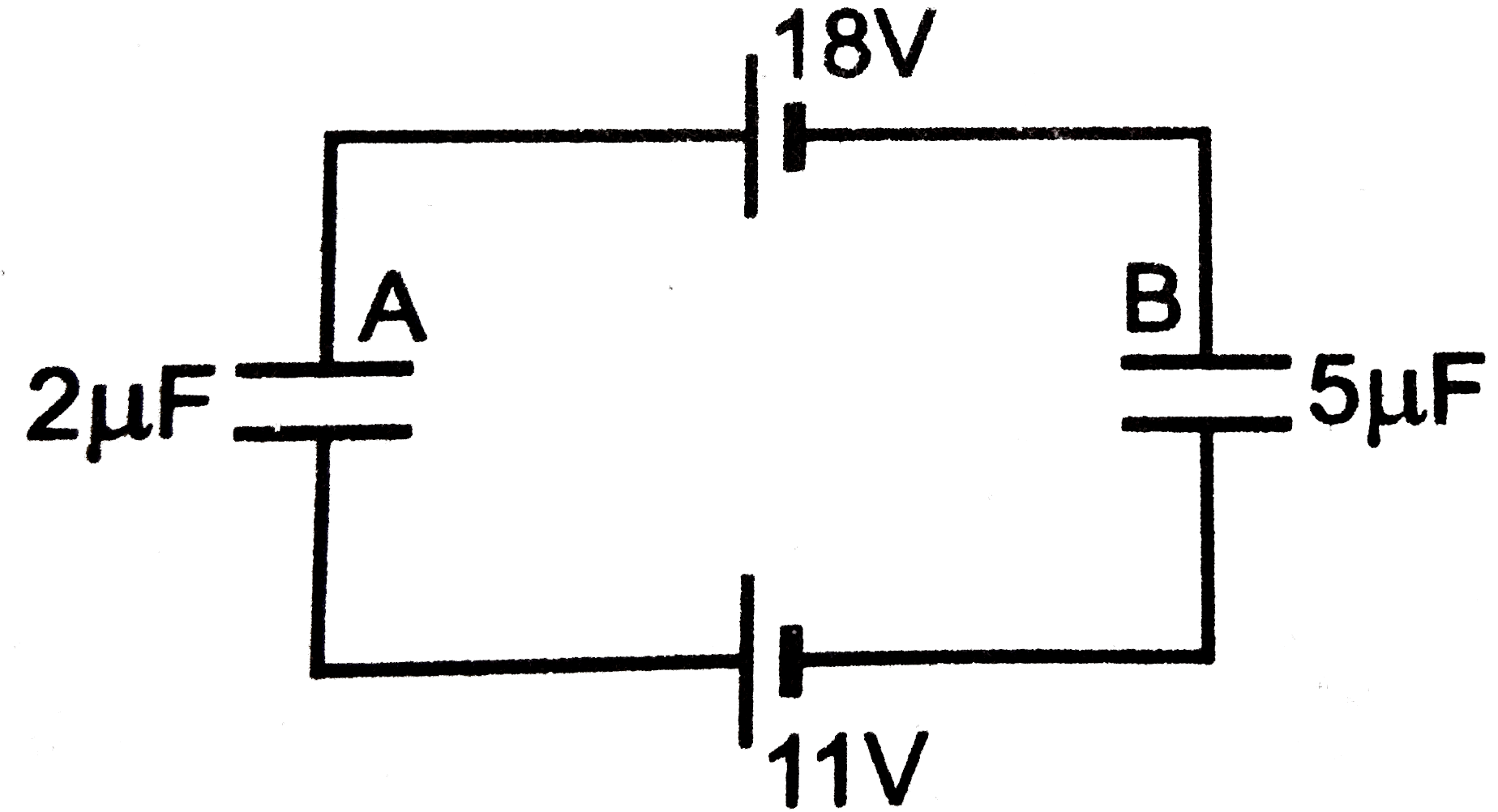 A2B5C7D18
A2B5C7D18Two capacitors of capacitances 10μFand20μF are connected in series across a potential difference of 100V. The potential difference across each capacitor is respectively
A66.67 V, 33.33 VB60 V, 40 VC50 V, 50 VD90 V, 10 V
Two capacitors of capacitance C1=2μF and C2=8μF are connected in series and the resulting combination is connected across 300 volts. Calculate the charge, potential difference and energy stored in the capacitor separately.
View SolutionThree capacitors of capacities 8μF,8μFand4μF are connected in a series and a potential difference of 120 volt is maintained across the combination. Calculate the charge on capacitor of capacity 4μF.
View SolutionThree capacitors of capacities 8μF,8μFand4μF are connected in a series and a potential difference of 120 volt is maintained across the combination. Calculate the charge on capacitor of capacity 4μF.
View SolutionThree capacitors of capacities 12μF,6μFand4μF are connected in series and a potential difference of 20 V is applied to their combination. What is the cahrge on the capacitor of 4μF ?
View SolutionTwo capacitors 3μF and 6μF are connected in series across a potential difference of 120V. Then the potential difference across 3μF capacitor is:
View Solution
