In a region of constant potential
the electric field is uniform.
the electric field is zero.
there can be no charge inside the region.
the electric field shall necessarily change if a charge is placed outside the region.
The correct Answer is:B, C
The relation between the electric field (E ) and electric potential (V) is given by:
E=−dVdr As the electric potential is constant:
⇒E=−d(constant)dr=0.
Therefore, electric field is zero. When the electric field is zero in a region of space, then electric flux linked with any closed surface within this region will be zero. From Gauss.s law, we can deduce that enclosed charge must also be zero.
Visualise a case of some metallic object. We know that potential everywhere on metallic object remains the same in electrostatics. Hence, its volume can be considered as a region of constant potential and zero electric field. If we place some charge near this metal object then also electric field inside metal remains zero. Hence elecrtric field in the region of constant potential may not change if a charge is placed outside the region. Hence, option (d) is not correct.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Chapter Practice Test
9 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Competition file (NCERT Exemplar Problems: Multiple Choice Questions Type-I)
6 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
- MAGNETISM AND MATTERBOOK - MODERN PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
16 Videos
Similar Questions
(Figure 3.41) shows the lines of constant potential in region in which an electric field is present. The values of potentials are written in brackets. At which points A, B or C, the electric field is greatest.
.
View SolutionA proton and α−partic≤ are accelerated with same potential difference and they enter in the region of constant magnetic field B perpendicular to the velocity of particles. Find the ratio of radius of curvature of alpa−partic≤.
View Solution
Knowledge Check
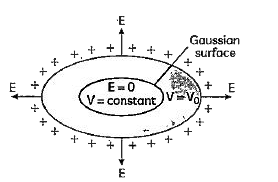
In the region of constant potential:Athe electric field is uniformBthere can be no charge inside the regionCthe electric field is zeroDboth (b) and (c)When a parallel plate capacitor is connected to a source of constant potential difference
Aall the charge drawn from source is stored in the capacitorBthe potential difference across capacitor grows very rapidly initially and this rate decreases to zero eventuallyConly half of the energy drawn from the source is dissipated outside the capacitorDall of theseTwo long coaxial cylindrical metal tubes stand on an insulatiog floor as shown in figure. A dielectric oil is filled in the annular region between the tubes. The tubes are maintained at a constant potential difference V. A small hole is opened at bottom then :
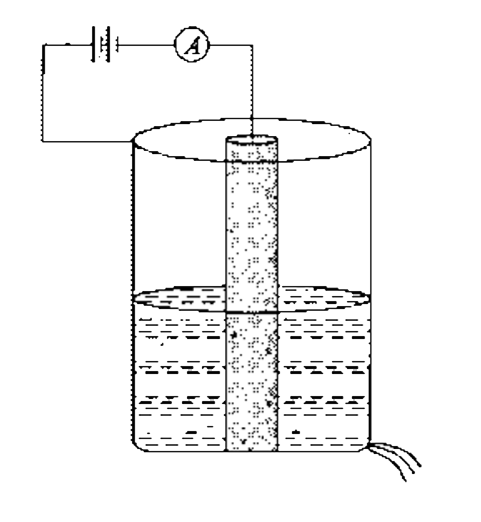AReading of ammeter remain constantBCapacitance of system increasesCCurrent in circuit is dependent on area ofholeDCurrent in circuit is inversely proportional to dielectric constant
Assertion: In parallel combination of electrical appliances, total power consum ption is equal to the sum of the powers of the indivisual appliances.
Reason : Electrons move away from a region of lower potential to a region of higher potential.
View SolutionIf the length of a potentiometer wire is increased by keeping constant potential difference across the wire, then
View SolutionStatement I: If electric potential in certain region is constant, then the electric field must be zero in this region.
Statement II: →E=−dVdrˆv.
View SolutionAn electron traveling in a uniform electric field passes from a region of potential V1 to a region of higher potential V2. Then
View SolutionA particle of mass m is present in a region where the potential energy of the particle depends on the x-coordinate according to the expression U=ax2−bx, where a and b are positive constant. The particle will perform.
View Solution