Two circular coils are kept in vicinity of each other. The current in primary coil changes from 10 A to 6 A in 0.3 sec. Calculate the coefficient of mutual inductance of the pair of emf of 1V induced in secondary coil.
The correct Answer is:0.075 H
To solve the problem, we will use the formula for the induced electromotive force (emf) in the secondary coil due to the changing current in the primary coil. The relationship is given by Faraday's law of electromagnetic induction.
1. Identify the Given Values:
- Initial current in the primary coil, I1=10A
- Final current in the primary coil, I2=6A
- Time interval, Δt=0.3s
- Induced emf in the secondary coil, emf=1V
2. Calculate the Change in Current:
ΔI=I2−I1=6A−10A=−4A
3. Determine the Rate of Change of Current:
dIdt=ΔIΔt=−4A0.3s=−403A/s
4. Use Faraday's Law to Relate Induced emf to Mutual Inductance:
According to Faraday's law, the induced emf (emf) in the secondary coil is given by:
emf=−MdIdt
Here, M is the mutual inductance we need to find.
5. Substituting the Values:
1V=−M(−403A/s)
Simplifying this gives:
1=M⋅403
6. Solve for Mutual Inductance M:
M=340H
Final Answer:
The coefficient of mutual inductance M is 340H.
---
- Initial current in the primary coil, I1=10A
- Final current in the primary coil, I2=6A
- Time interval, Δt=0.3s
- Induced emf in the secondary coil, emf=1V
ΔI=I2−I1=6A−10A=−4A
dIdt=ΔIΔt=−4A0.3s=−403A/s
According to Faraday's law, the induced emf (emf) in the secondary coil is given by:
emf=−MdIdt
Here, M is the mutual inductance we need to find.
1V=−M(−403A/s)
Simplifying this gives:
1=M⋅403
M=340H
The coefficient of mutual inductance M is 340H.
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - CONCEPTUAL QUESTIONS22videos
ELECTROMAGNETIC INDUCTION
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - TOUGH & TRICKY PROBLEMS14videos
ELECTROMAGNETIC INDUCTION
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION14videos
ELECTROMAGNETIC INDUCTION
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Chapter Practice Test15videos
ELECTRIC CHARGES AND FIELDS
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST14videos
ELECTROMAGNETIC WAVES
Similar Questions
Current in primary coil changes from 20 A to 0 A uniformly in 0.005 sec. Calculate the emf induced in the secondary coil if coefficient of mutual inductance is 2 H.
View SolutionThe average emf induced in the secondary coil is 0.1V when the current in the primary coil changes from 1 to 2A in 0.1s.What is the mutual inductance of the coils.
View Solution
Knowledge Check
- Question 1 - Select One
The current in a coil decreases from 1 A to 0.2 A. In 10 sec. Calculate the coefficient of self inductance. If induced emf is 0.4 volt .
A5 HB3HC4 HD2H - Question 2 - Select One
The current in a coil changes from 0 to 2 A in 0.05 sec. If the induced e.m.f is 80 V, the self inductance of the coil is
A2.4 HB2 HC1.5 HD1 H - Question 3 - Select One
When the current in a coil charges from 2A to 4A in 0.05 s, emf of 8 volt is induced in the coil. The coefficient of self induction of the coil is -
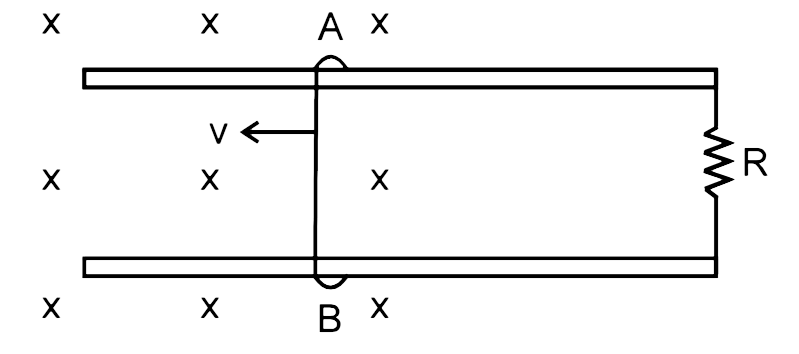 AincreaseBdecreaseCremains the sameDMay increase or decrease depending on whether the semicircle bulges towards the resistance or away from it.
AincreaseBdecreaseCremains the sameDMay increase or decrease depending on whether the semicircle bulges towards the resistance or away from it.
An e.m.f. of 0.5 V is developed in the secondary coil, when current in primary coiol changes form 5.0 A to 2.0 A in 300 millisec. Calculate the mutal inductance of tow coils.
View SolutionAn emf of 40 kV is induced in the secondary of car spark coil when the current in primary changes from 5A to 2A in 20μs. Calculate the mutual inductance between the primary and secondary windings of this coil.
View SolutionA carspark coil developes an induced e.m.f. of 40000 V in the secondary when when current in primary changes form 4 A to zero in 10μs. What is the mutual inductance of the coil ?
View SolutionWhen the current in a coil charges from 2A to 4A in 0.05 s, emf of 8 volt is induced in the coil. The coefficient of self induction of the coil is -
View SolutionA varying current in a coil change from 10A to 0A in 0.5sec. If the average emf induced in the coil is 220V, the self inductance of the coil is
View Solution

