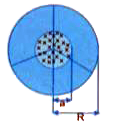
A line charge lsmbda per unit length is lodged uniformly onto the rim of a wheel of mass M and radius R. The wheel has light non-conducting spokes and is free to rotate without friction about its axis (refer to the figure given). A uniform magnetic field extends over a circular region within the rim. It is given by
→B=[−B00ˆk(r≤a,a<R)(otherwise)
What is the angular velocity of the wheel after the field is suddenly switched off?

Area of wheel under the influence of magnetic field is
A=πa2
The magnetic flux linked with area A is
ϕ=πa2B
The induced emf in the loop is
ε=−dϕdt=−ddt(BA)=−AdBdt=−πa2dBdt ...(i)
The change in magnetic field B gives rise to the electric field E.
Work done in moving a charge q once around the loop of radius R is
W=Electric force×Distance=qE×2πR
⇒Wq=2πRE
Also, ε=Wq (By definition of electric potential)
⇒ε=2πRE ...(ii)
From (i) and (ii), we have
−πa2dBdt=2πRE
⇒a2dB=−2REdt ...(iii)
Thus magnitude of average electric field developed can be written as follows:
E=a22RΔBΔt
This electric field is along closed circular lines and the ring is also charged so it will apply tangential force on the ring to apply torque. Impulse of this torque will provide angular speed to ring.
We know that, angular impulse=τΔt=qERΔt (in this case)
Also, if mass = M, then
Angular impulse = Change in angular momentum
qERΔt=MR2ω (ω is the angular velocity)
qa22RΔBΔtRΔt=MR2ω
ω=qa22ΔBMR2
Here, ΔB=B0
λ=q2πR
⇒ω=λπa2B0MR
The distance of ω will be along negative Z-axis.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - NCERT FILE (NCERT EXEMPLAR PROBLEMS SUBJECTIVE QUESTIONS) (Very short answer type questions)
6 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - NCERT FILE (NCERT EXEMPLAR PROBLEMS SUBJECTIVE QUESTIONS) (Short answer type questions)
5 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - NCERT FILE (NCERT TEXTBOOK EXERCISE)
10 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Chapter Practice Test
15 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
Similar Questions
A line charge λ per unit length is lodged uniformly onto the rim of a wheel of mass M and radius R. The wheel has light non-conducting spokes and is free to rotate without friction about its axis (Fig. 6.08). A uniform magnetic field extends over a circular region within the rim. It is given by,
→B=−B0ˆk(r≤a,aItR)
= 0 (otherwise)
What is the angular velocity of the wheel after the field is suddenly switched off ?
View SolutionA line charge λ per unit length is lodged uniformly onto the rim of a wheel of mass M and radius R. The wheel has light non-conducting spokes and is free to rotate without friction about its axis (Fig.). A uniform magnetic field extends over a circular region within the rim. It is given by,
B=−B0k (r≤a,a<R)
=0 (otherwise)
What is the angular velocity of the wheel after the field is suddenly switched off?

View Solution
Knowledge Check
A line charge λ per unit length is pasted uniformly on to the wire of a wheel of mass m and radius R. The wheel has light non-conducting spokes and is free to rotate about a vertical axis as shown in Fig.3.166. A uniform magnetic field extends over a radial rigion of radius r given by B=−B0ˆk(r≤a,a<R)=0 (otherwise). What is the angular velocity of the wheel this field is suddenly switched off?
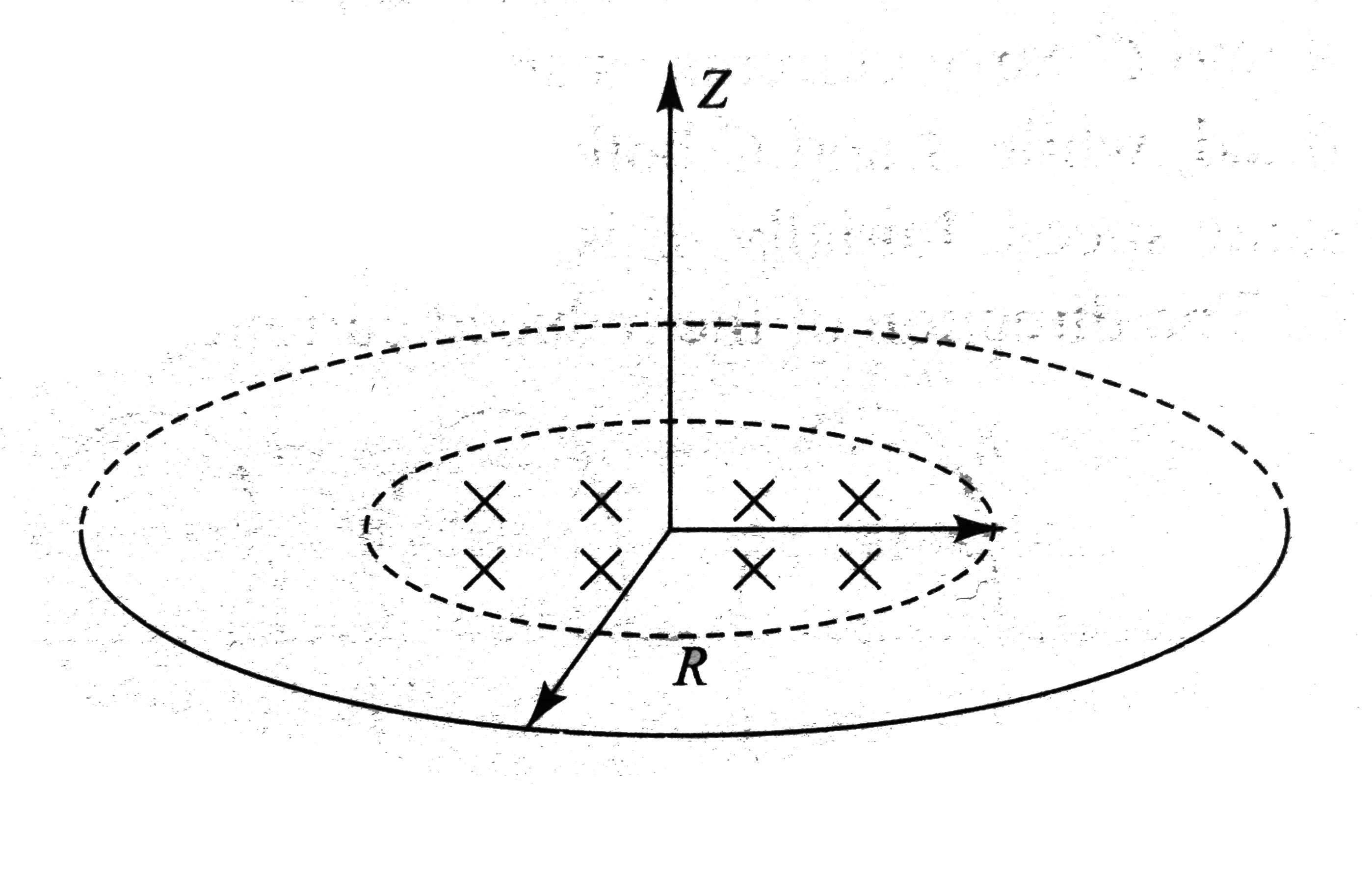 A(a) −2B0πa2rmRˆkB(b) −2B0πa2r3mRˆkC( c) B0πa2λmRˆkD( d) −B0πa2λmRˆk
A(a) −2B0πa2rmRˆkB(b) −2B0πa2r3mRˆkC( c) B0πa2λmRˆkD( d) −B0πa2λmRˆkA wheel comprises a ring of radius R and mass M and three spokes of mass m each. The moment of inertia of the wheel about its axis is
 A(M+m4)R2B(M+m)R2C(M+3m)R2D(M+m2)R2
A(M+m4)R2B(M+m)R2C(M+3m)R2D(M+m2)R2A non-conducting disk of radius R is rotating about its own axis with constant angular velocity ω in a perpendicular uniform magnetic field B as shown in figure. The emf induced between centre and rim of disk is
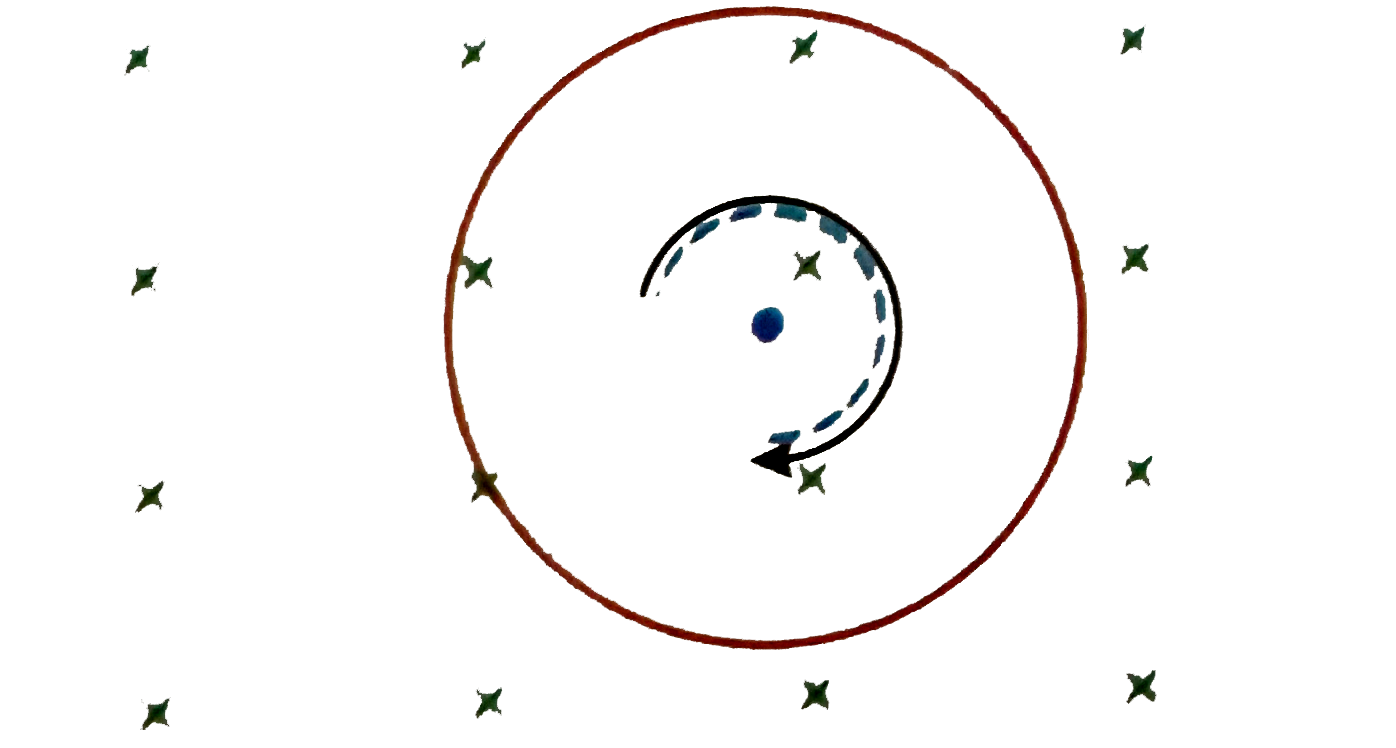 ABωR22BBωR2CZeroDBωR23
ABωR22BBωR2CZeroDBωR23
A line charge λ per unit length is lodged uniformly onto the rim of a wheel of mass M and radius R. The wheel has light non-conducting spokes and is free to rotate without friction about its axis (Fig. 6.22). A uniform magnetic field extends over a circular region within the rim. It is given by,
B=B0k (ra≤a,a<R)
=0 (otherwise)
What is the angular velocity of the wheel after the field is suddenly switched off?

View SolutionA line charge λ per unit length is pasted uniformly on to the wire of a wheel of mass m and radius R. The wheel has light non-conducting spokes and is free to rotate about a vertical axis as shown in Fig.3.166. A uniform magnetic field extends over a radial rigion of radius r given by B=−B0ˆk(r≤a,a<R)=0 (otherwise). What is the angular velocity of the wheel this field is suddenly switched off?
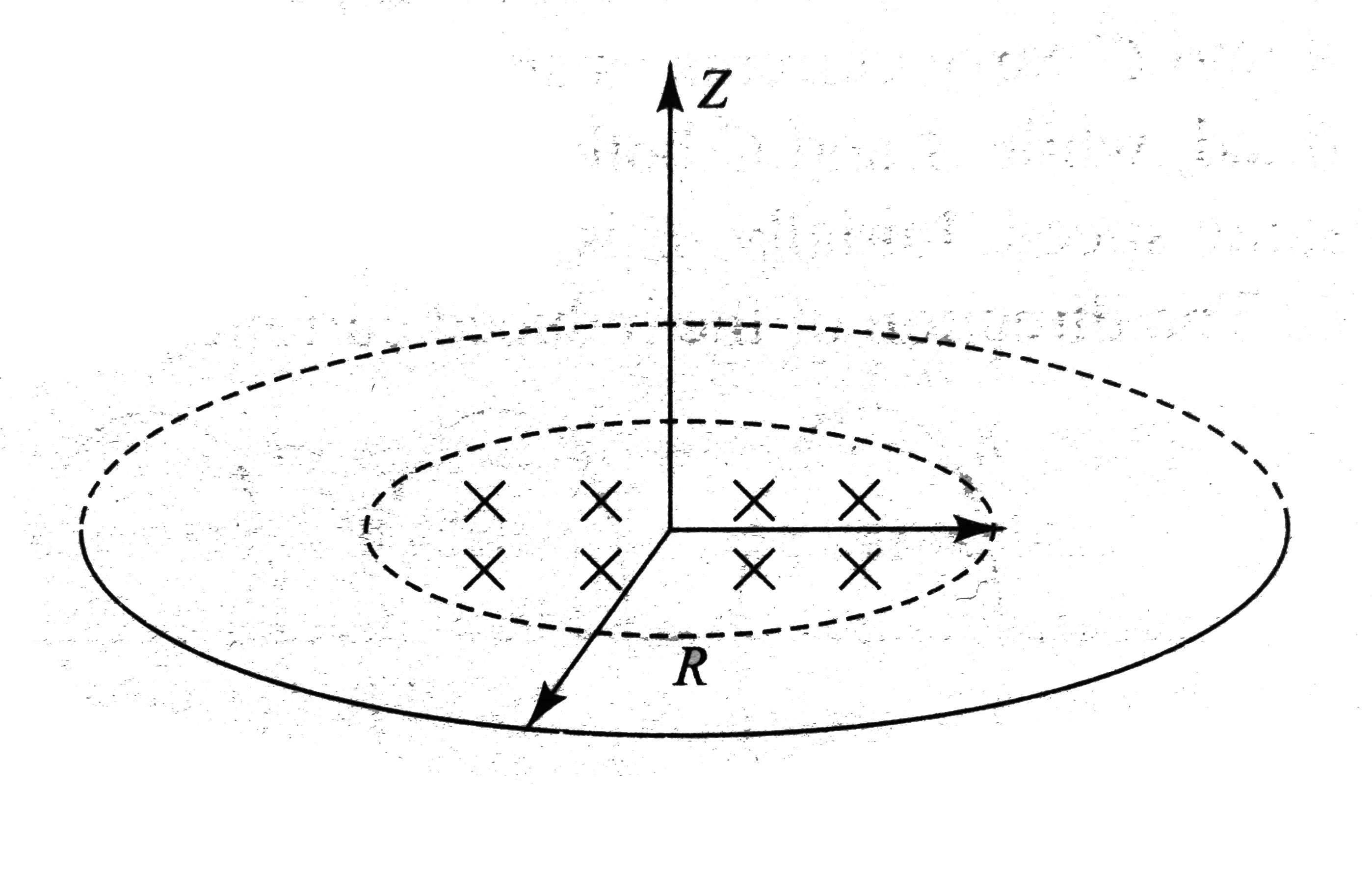
View SolutionA wheel comprises a ring of radius R and mass M and three spokes of mass m each. The moment of inertia of the wheel about its axis is

View SolutionA charge Q is spread uniformly over an insulated ring of radius R.What is the magnetic moment of the ring if it is rotated with an angluar velocity ω about its axis?
View SolutionA circular wheel is rotating in horizontal plane without friction about its axis. If a body is gently attached to the rim of the wheel then following is false.
View Solution
