What is mutual induction? Find the coefficient of mutual induction and also define S.I. unit of mutual induction.
Step-by-Step Solution
1. Definition of Mutual Induction:
Mutual induction occurs when two coils are placed close to each other, such that the magnetic field produced by one coil influences the other coil. This interaction leads to the generation of an electromotive force (EMF) in the second coil due to a change in current in the first coil.
2. Understanding the Coefficient of Mutual Induction:
The coefficient of mutual induction (M) quantifies the extent to which a change in current in one coil induces an EMF in the other coil. It is defined mathematically as:
M=N2Φ12I1
where:
- M is the mutual inductance,
- N2 is the number of turns in the second coil,
- Φ12 is the magnetic flux through the second coil due to the current I1 in the first coil.
3. Relationship with Self-Inductance:
If we denote the self-inductances of the two coils as L1 and L2, the mutual inductance can also be expressed in terms of the coupling coefficient k:
M=k√L1L2
where k is a dimensionless coefficient that represents the coupling between the two coils.
4. S.I. Unit of Mutual Induction:
The SI unit of mutual inductance is the Henry (H). One Henry is defined as the amount of mutual inductance that will induce an EMF of one volt when the current changing at the rate of one ampere per second.
Summary
- Mutual Induction: Interaction between two coils leading to induced EMF in one due to changing current in the other.
- Coefficient of Mutual Induction (M): M=N2Φ12I1 and M=k√L1L2.
- SI Unit: Henry (H), equivalent to V·s/A.
Mutual induction occurs when two coils are placed close to each other, such that the magnetic field produced by one coil influences the other coil. This interaction leads to the generation of an electromotive force (EMF) in the second coil due to a change in current in the first coil.
The coefficient of mutual induction (M) quantifies the extent to which a change in current in one coil induces an EMF in the other coil. It is defined mathematically as:
M=N2Φ12I1
where:
- M is the mutual inductance,
- N2 is the number of turns in the second coil,
- Φ12 is the magnetic flux through the second coil due to the current I1 in the first coil.
If we denote the self-inductances of the two coils as L1 and L2, the mutual inductance can also be expressed in terms of the coupling coefficient k:
M=k√L1L2
where k is a dimensionless coefficient that represents the coupling between the two coils.
The SI unit of mutual inductance is the Henry (H). One Henry is defined as the amount of mutual inductance that will induce an EMF of one volt when the current changing at the rate of one ampere per second.
- Mutual Induction: Interaction between two coils leading to induced EMF in one due to changing current in the other.
- Coefficient of Mutual Induction (M): M=N2Φ12I1 and M=k√L1L2.
- SI Unit: Henry (H), equivalent to V·s/A.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - REVISION EXERCISE (Long Answer Questions)
9 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - REVISION EXERCISE (Numerical Problems)
16 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - REVISION EXERCISE (Fill in the blanks)
7 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Chapter Practice Test
15 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
Similar Questions
Knowledge Check
The coefficient of mutual inductance
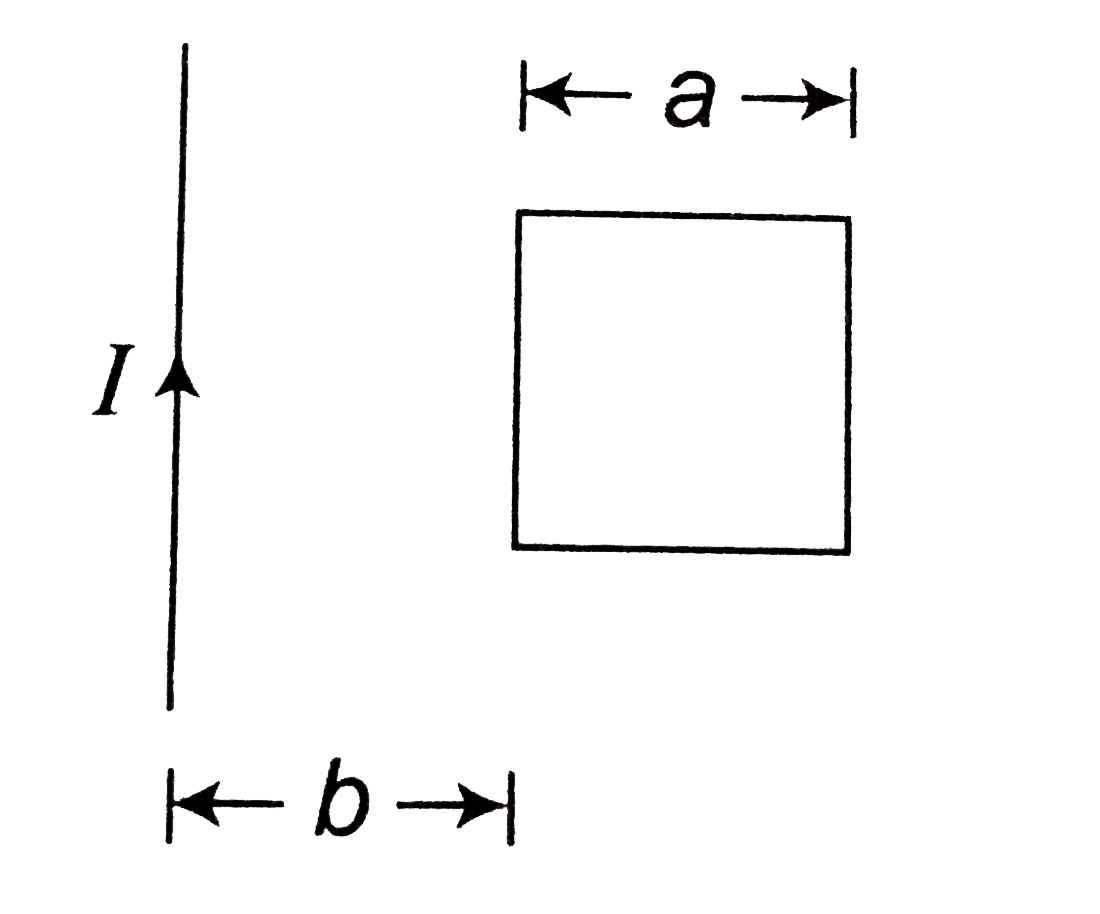 Aμ0a2π1n(1+a2b)Bμ0aπ1n(1+b2a)Cμ0a2π1n(1+ab)Dμ0a2π1n(1+ba)
Aμ0a2π1n(1+a2b)Bμ0aπ1n(1+b2a)Cμ0a2π1n(1+ab)Dμ0a2π1n(1+ba)What is the SI unit of mutual inductance?
AWeberBHenryCFaradDTeslaThe unit of mutual inductance is-
AvoltBweberCteslaDhenry
