There is one composite rod. Half length (l2) is made of wood and other half is made of metal as shown in figure.

Rod is made to rotate about wooden end as shown in figure. Magnetic field B exists perpendicular to the plane of rotation. Potential difference developed between the two ends of the metal rod is found to be 3nBωl2 Find value of n.
The correct Answer is:8
Let us select a segment at a distance x from centre of rotation of width dx as shown in figure,

Speed of the segment is xω. And emf developed across the segment can be written as follows:
dV=Bxωdx
Total potential difference developed across the length of metal rod can be calculated by integrating above relation
V=Bω∫ll/2xdx
V=Bω[x22]ll/2
V=Bω[l22−l28]⇒38Bωl2
Hence value of n is 8.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - COMPETITION FILE (EXEMPLAR PROBLEMS)(OBJECTIVE QEUSTIONS)
10 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
14 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - COMPETITION FILE (ASSERTION REASON TYPE QUESTIONS)
10 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Chapter Practice Test
15 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CHAPTER PRACTICE TEST
14 Videos
Similar Questions
A rod of length l rotates with a uniform angular velocity omega about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the two ends of the lrod is
View SolutionA rod of length l rotates with a small but uniform angular velocity ω about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the centre of the rod and an end is
View Solution
Knowledge Check
A rod of length l rotates with a uniform angular velocity omega about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the two ends of the rod is
ABl2ω2BzeroC(Bl2ω8)D2Bl2ωA rod of length l rotates with a uniform angular velocity omega about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the two ends of the lrod is
ABl2ω2BzeroC(Bl2ω8)D2Bl2ωA rod of length l rotates with a uniform angular velocity omega about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the two ends of the rod is
AzeroB12ωBI2CωBI2D2ωBI2
A rod of length l rotates with a small but uniform angular velocity ω about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The potential difference between the centre of the rod and an end is
View SolutionA straight rod of length L is rotating about an axis passing through O and perpendicular to the plane. In the space a uniform magnetic field B exits normal to the plane of rotation. Potential difference between a & b is equal to

View SolutionRod PQ of length 2l is rotating about one end P in a uniform magnetic field B which is perpendicular to the plane of rotation of the rod . Point M is the mid- point of the rod. Find the induced emf between M and Q if the potential between P and Q is 100V.
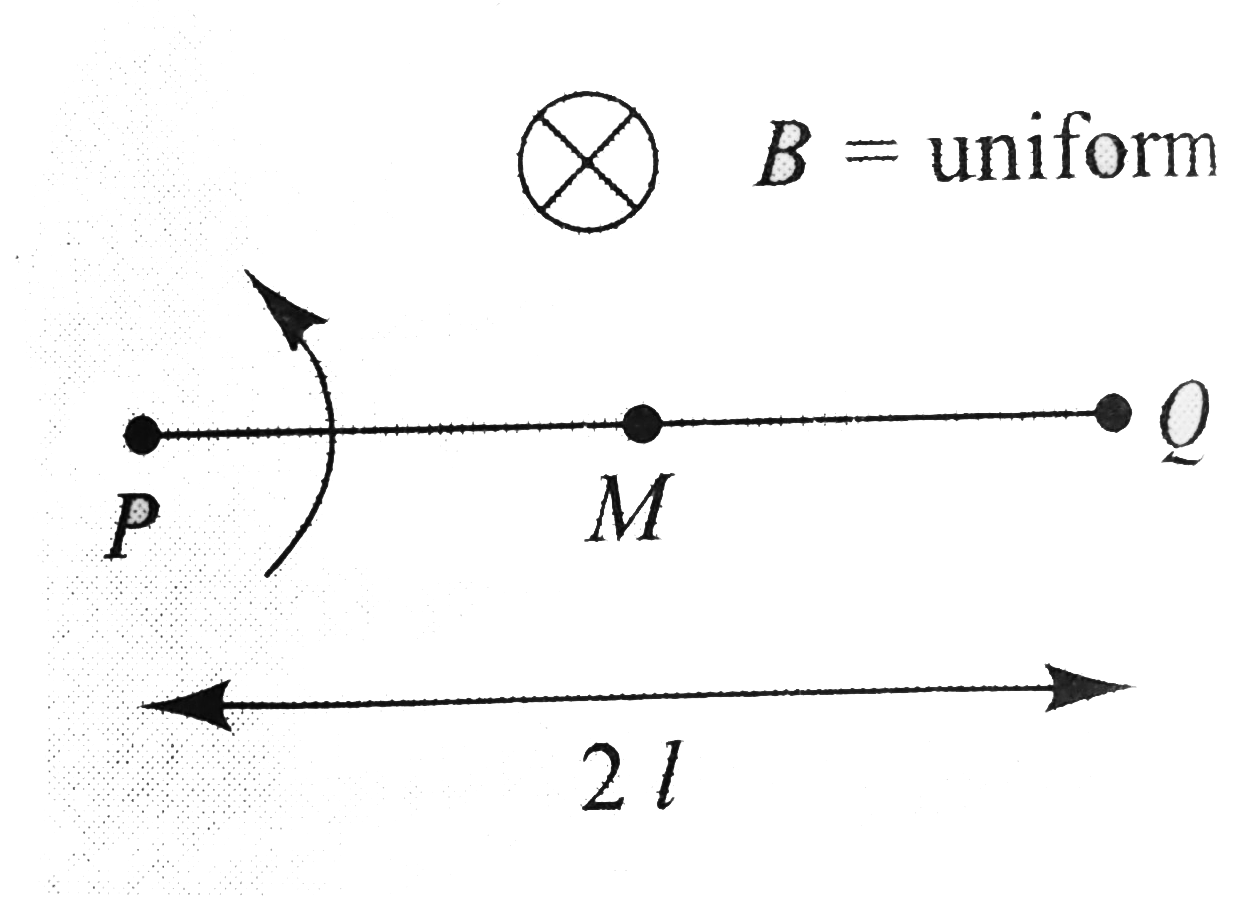
View SolutionRod PQ of length 2l is rotating about one end P in a uniform magnetic field B which is perpendicular to the plane of rotation of the rod . Point M is the mid- point of the rod. Find the induced emf between M and Q if the potential between P and Q is 100V.

View SolutionA rod of length l is rotated with angular speed ω about an axis passing through the centre of rod and perpendicular to its length. A uniform magnetic field B is applied parallel to the axis of rotation, Potential difference developed between the ends of the rod is
View Solution
