Question

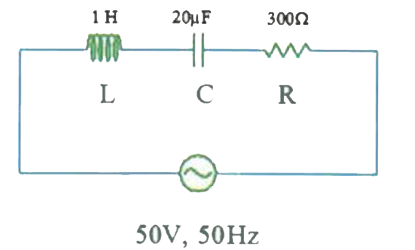
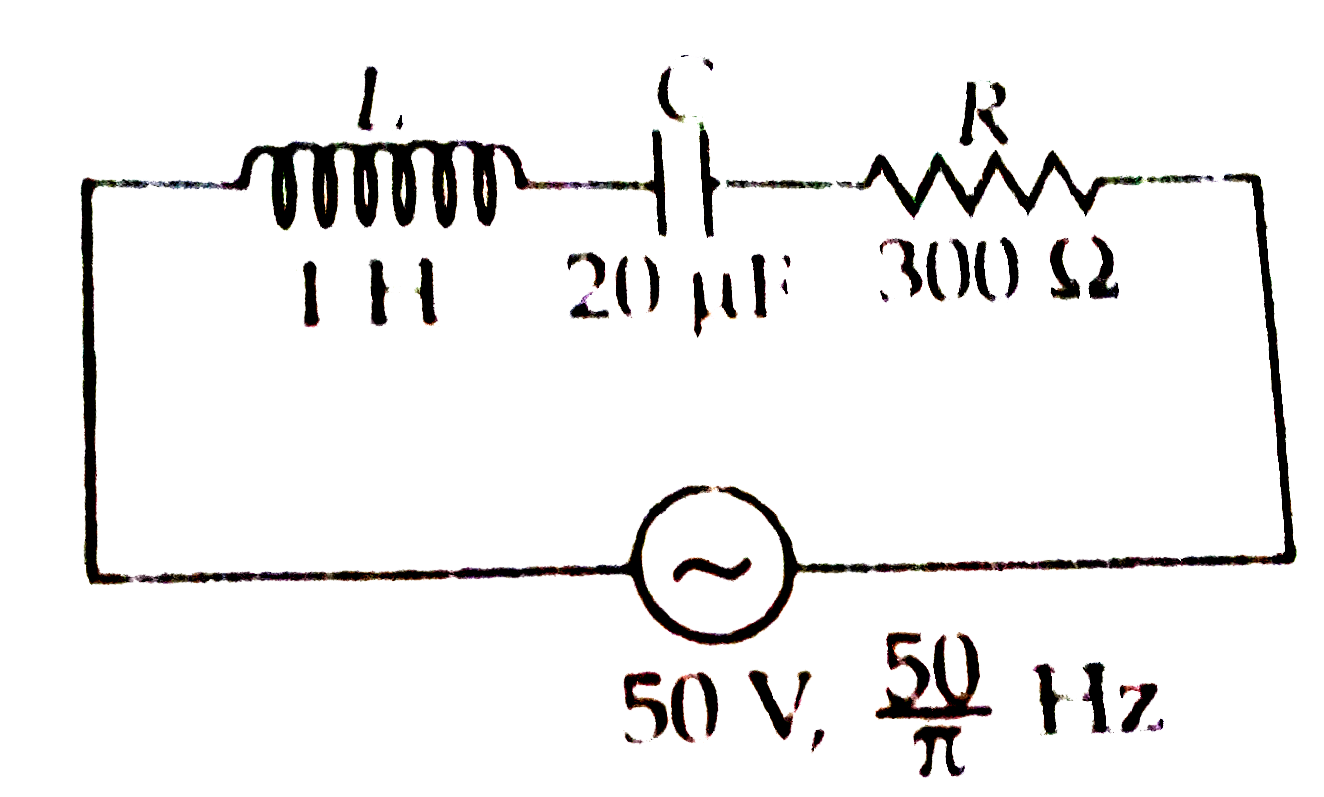In a series LCR circuit, how can we achieve the maximum power by choosing appropriate value of capacitance of the capacitor in the circuit ?

Explanation

To achieve maximum power in a series LCR (Inductor-Capacitor-Resistor) circuit by choosing an appropriate value of capacitance, follow these steps:

1. Understand the Power Formula: The power drawn from the source in an LCR circuit is given by the formula:
\( P = V_{\text{rms}} \cdot I_{\text{rms}} \cdot \cos \phi \)
where \( \phi \) is the phase angle between the voltage and the current.

2. Identify the Role of Power Factor: The term \( \cos \phi \) is known as the power factor. To achieve maximum power, we need to maximize \( \cos \phi \).

3. Relate Power Factor to Impedance: The power factor \( \cos \phi \) can be expressed in terms of resistance \( R \) and impedance \( Z \):
\( \cos \phi = \frac{R}{Z} \)
where \( Z \) is the total impedance of the circuit.

4. Determine Impedance in LCR Circuit: In a series LCR circuit, the total impedance \( Z \) is given by:
\( Z = \sqrt{R^2 + (X_L - X_C)^2} \)
where \( X_L \) is the inductive reactance and \( X_C \) is the capacitive reactance.

5. Condition for Maximum Power: For maximum power, we want \( \cos \phi \) to be equal to 1. This occurs when the phase angle \( \phi \) is 0 degrees, meaning:
\( X_L - X_C = 0 \)
or equivalently,
\( X_L = X_C \)

6. Express Reactance in Terms of Capacitance: The inductive reactance \( X_L \) is given by:
\( X_L = \omega L \)
and the capacitive reactance \( X_C \) is given by:
\( X_C = \frac{1}{\omega C} \)
where \( \omega \) is the angular frequency of the AC source.

7. Set Inductive and Capacitive Reactance Equal: To achieve resonance (maximum power), set \( X_L \) equal to \( X_C \):
\( \omega L = \frac{1}{\omega C} \)

8. Solve for Capacitance: Rearranging the equation gives:
\( C = \frac{1}{\omega^2 L} \)
This equation shows how to choose the capacitance \( C \) to achieve maximum power in the circuit.

Conclusion:
To achieve maximum power in a series LCR circuit, select the capacitance \( C \) such that the capacitive reactance equals the inductive reactance, which can be calculated using the formula \( C = \frac{1}{\omega^2 L} \).