A point charge is moving along a straight line with a constant velocity v. Consider a small area A perpendicular to the direction of motion of the charge . Calculate the displacement current through the area when its distance from the charge is x. The value of x is not large so that the electric field at any instant is essentially given by Coulomb's law.
(##HCV_VOL2_C40_E01_023_Q01##)
Electric field lines of a point charge are radially outwards or inwards depending on whether charge is positive or negative .SO direction of electric field at a point on line of motion of point charge will be along the line of motion and hence perpendicular to the area selected . hence magnitude of the electric flux associated with this area when charge is at a distance x from area can be written as follows :
ϕ−qA4πε0x2
Note that above electric flux is written for every small area A. Displacement current through any surface can be written as :
id=ε0dϕdt
=Id=qAd(x−2)4πdt
=Id=qA4π(dx−2dx)(dxdt)
Hence , magnitude of displacement current can be written as :
⇒id=qA4π|−2|(x3)v
⇒Id=qAv2πx3
We can see that displacement current is inversely proportional to the cube of the distance of point charge from that point .
Topper's Solved these Questions
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - PRACTICE PROBLEMS
14 Videos
- ELECTROMAGNETIC WAVESBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - CONCEPTUAL QUESTIONS
12 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
14 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Chapter Practice Test
9 Videos
Similar Questions
A charged particle of charge 2μC and mass 10 milligram, moving with a velocity of 1000m/s enters a uniform electric field of strength 103N/C directed perpendicular to its direction of motion. Find the velocity and displacement of the particle after 10s.
View SolutionFor a particle moving along a straight line, the relation between the velocity and the displacement at any instant is given by v2=S2+2S2+3
Find the acceleration of the particle when it is at a distance of 4 meters from a fixed point on the line.
View Solution
Knowledge Check
A long wire having linear charge density λ moving with constant velocity v along its length. A point charge moving with same speed in opposite direction and at that instant it is at a distance r from the wire. The net force acting on the charge is given by
 Aλq2πr[1ε0+v2μ0]Bλq2πr[1ε0−μ0v2]Cλq2πr√(1ε0)2+v4μ20Dzero
Aλq2πr[1ε0+v2μ0]Bλq2πr[1ε0−μ0v2]Cλq2πr√(1ε0)2+v4μ20DzeroThe law of motion of a body moving along a straight line is x=12vt, x being its distance from a fixed point on the line at time t and v is its velocity there. Then
Aacceleration f varies directly with xBacceleration f varies inversely with xCacceleration f is constantDacceleration f varies directly with t
The electric foield at a point A on the perpendicular bisector of a uniformly charged wire of length l=3m and total charge q = 5 nC is x V/m. The distance of A from the centre of the wire is b = 2m. Find the value of x.
View SolutionAn infinite line cahrge is perpendicular to the plane of the figure having linear charge density λ, A partcle having charge Q and mass m is projected in the field of the line cahrge fromo point P. the point P is at a distance R from the line cahrge and velocity given tot he particle is perpendicular tot he radial line at P (see figure)
(i). Find the speed of the particle when its distance from the line cahrge grows the ηR(η>1)
(ii). Find the velocity component of the particle along the radial line (joining the line charge to the particle) at the instant its distance becomes ηR.

View SolutionA point charge q moves with a non-relatives velocity v = const. Find the displacement current density .d at a point location at a distance r from the charges on a straight line
(a) coinciding with the charge path,
(b) perpendicualr to the path and passing through the charge.
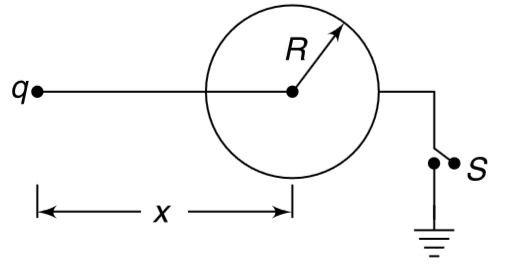
View SolutionA point charge q is placed at a distance x from the centre of a conducting sphere of radius R(lt x). (a) How much charge will flow through the switch S when it is closed to ground the sphere? (b) Find the current through the switch S when charge ‘q’ is moved towards the sphere with velocity V.
View SolutionThe ratio of energy density in electric field (UE) to square of potential (V2) at point 'A' at a distance 'x' from static point charge Q is 'n'.The value of this ratio at a distance '3xfrom the charge is..?
View SolutionThere is an electric field E in x-direction. If the work done on moving a charge of 0.2C through a distance of 2w m along a line making a angle 60∘ with x-axis is 4 J, then what is the value of E?
View Solution
