An electromagnetic wave travelling along z-axis is given as E=E0 cos (kz−ωt). Choose the correct options from the following
The associated magnetic field is given as →B=1cˆk×→E=1ω(ˆk×vevE).
The electromagentic field can be written in terms of the associated magnetic field as →E=c(→B׈k).
ˆk.→E=0,ˆk→B=0
ˆk×→E=0,ˆk×→B=0
The correct Answer is:A, B, C
Let us consider an electromagnetic wave is travelling along Z-direction. The electric field vector is given by
→E=E0cos(kz−ωt) (j )
It acts along the Y- direction. So, the associated magnetic field is along the X axis, i.e. along ˆk×→E
∴→B=ˆk×Ecas→B=→E/c
Also, the associated electric field can be written in terms of magnetic field as
→E=c(→B׈k)
The angle between ˆk and →Eis 90∘. So, ˆk.E=0. Also, the angle between k and B is 90∘. Thus, k-B is also equal to zero.
The correct options are (a), (b) and (c).
Topper's Solved these Questions
- ELECTROMAGNETIC WAVESBook:MODERN PUBLICATIONChapter:ELECTROMAGNETIC WAVESExercise:NCERT FILE ( NCERT EXEMPLAR PROBLEMS SUBJECTIVE QUESTIONS ) ( VERY SHORT ANSWER TYPE QUESTIONS )
Explore 7 Videos
- ELECTROMAGNETIC WAVESBook:MODERN PUBLICATIONChapter:ELECTROMAGNETIC WAVESExercise:NCERT FILE ( NCERT EXEMPLAR PROBLEMS SUBJECTIVE QUESTIONS ) ( SHORT ANSWER TYPE QUESTIONS )
Explore 7 Videos
- ELECTROMAGNETIC WAVESBook:MODERN PUBLICATIONChapter:ELECTROMAGNETIC WAVESExercise:NCERT FILE ( NCERT ADDITIONAL EXERCISES )
Explore 4 Videos
- ELECTROMAGNETIC INDUCTIONBook:MODERN PUBLICATIONChapter:ELECTROMAGNETIC INDUCTIONExercise:CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
Explore 14 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE Book:MODERN PUBLICATIONChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCE Exercise:Chapter Practice Test
Explore 9 Videos
Similar Questions
Knowledge Check
An electromagnetic wave travelling along z-axis is given as E=E0 cos (kz−ωt). Choose the correct options from the following
AThe associated magnetic field is given as →B=1cˆk×→EBThe e.m. wave field can be written in terms of the associated magnetic field as →E=c(→B×׈k)Cˆk.→E=0,ˆk.→B=0Dˆk×→E=0,ˆk×→B=0An e.m. wave trevels in vacuum along z direction:
→E=(E1ˆi+E2ˆj)cos(kz−ωt). Choose the correct option from the following :AThe associated magnetic field is given as →B=1c(E1ˆi+E2ˆj)cos(kz−ωt)BThe associated magnetic field is given as →B=1c(E1ˆi−E2ˆj)cos(kz−ω)CThe given electromagnetic field circular polarisedDThe given e.m. wave is plane polarisedFrom the given, choose the correct option
 .Aacceleration of block A is zeroBacceleration of B is gCacceleration of block A is non zeroDtension in the string connecting A is zero
.Aacceleration of block A is zeroBacceleration of B is gCacceleration of block A is non zeroDtension in the string connecting A is zero
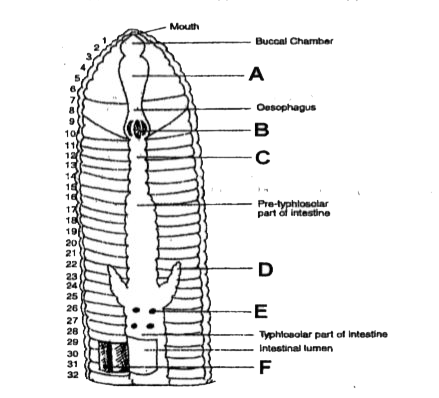
Identify A to E choose the correct option accordingly .
View Solution(a) Derive a relation for the velocity of electromagnetic wave.
(b) An electromagnetic wave is travelling along Z-axis is described by magnetic field
Bx=4×10−10sin(ωt−kx−kz)T
Find the maximum electric and magnetic field on an alpha particle moving along Y-axis with a speed of 5×106 m/s. Charge on the electron is1.6×10−19 C.
View SolutionWhich of the following statements is not correct ? Choose the correct options from the given below.
View SolutionIf the magnetic field B of a polarised electromagnetic wave oscillates parallel to y-axis and is given by : By=Bmsin(kz−ωt). What is the direction of propagation of the electromagnetic wave and parallel to which axis does the associated electric field oscillates ?
View SolutionA electromagnetic wave going through a medium is given by E=E0sin(kx–ωt)andB=B0sin(kx–ωt) then
View Solution