There is one parallel plate capacitor with circular plates of radius R. Capacitor is being charged by connecting to a battery . If I is the instantaneous conduction current flowing in the connecting wires then what will be displacement current flowing in the region between the plates enclosed between radii, to R
The correct Answer is:A
To solve the problem, we need to find the displacement current flowing in the region between the plates of a parallel plate capacitor, specifically between the radii R/2 and R.
1. Understanding the Setup:
We have a parallel plate capacitor with circular plates of radius R. The capacitor is being charged, which means that there is a conduction current I flowing in the connecting wires.
2. Concept of Displacement Current:
The displacement current ID is related to the changing electric field between the plates due to the charging of the capacitor. According to Maxwell's equations, the displacement current can be expressed as:
ID=ϵ0dΦEdt
where ΦE is the electric flux.
3. Electric Field Between the Plates:
The electric field E between the plates of a capacitor is given by:
E=σϵ0
where σ is the surface charge density on the plates. The charge Q on the plates is related to σ by:
Q=σ⋅A
where A is the area of the plates.
4. Area of the Plates:
The area A of the circular plates is:
A=πR2
5. Finding the Charge Density:
The surface charge density σ can be expressed as:
σ=QπR2
6. Calculating the Electric Flux:
The electric flux ΦE through the area between the radii R/2 and R can be calculated as:
ΦE=∫EdA
where dA is the differential area element. For a circular ring of radius r and thickness dr, the area element is:
dA=2πrdr
Thus, the flux becomes:
ΦE=∫RR/2E⋅2πrdr
7. Substituting for Electric Field:
Substituting E=Qϵ0πR2 into the flux equation gives:
ΦE=∫RR/2Qϵ0πR2⋅2πrdr
This simplifies to:
ΦE=Qϵ0R2∫RR/2rdr
8. Calculating the Integral:
The integral ∫RR/2rdr evaluates to:
(r22)RR/2=R22−(R/2)22=R22−R28=4R28−R28=3R28
9. Finding the Displacement Current:
The displacement current ID in the region between R/2 and R can now be expressed as:
ID=ϵ0dΦEdt
Since ΦE depends on Q, and Q is changing with time as the capacitor charges, we can relate ID to the conduction current I.
10. Using Kirchhoff's Current Law:
By Kirchhoff's current law, the conduction current I entering the capacitor must equal the displacement current ID flowing through the region. Since we are considering only the area between R/2 and R, we find that:
ID=34I
Final Answer:
Thus, the displacement current flowing in the region between the plates enclosed between the radii R/2 and R is:
3I4
We have a parallel plate capacitor with circular plates of radius R. The capacitor is being charged, which means that there is a conduction current I flowing in the connecting wires.
The displacement current ID is related to the changing electric field between the plates due to the charging of the capacitor. According to Maxwell's equations, the displacement current can be expressed as:
ID=ϵ0dΦEdt
where ΦE is the electric flux.
The electric field E between the plates of a capacitor is given by:
E=σϵ0
where σ is the surface charge density on the plates. The charge Q on the plates is related to σ by:
Q=σ⋅A
where A is the area of the plates.
The area A of the circular plates is:
A=πR2
The surface charge density σ can be expressed as:
σ=QπR2
The electric flux ΦE through the area between the radii R/2 and R can be calculated as:
ΦE=∫EdA
where dA is the differential area element. For a circular ring of radius r and thickness dr, the area element is:
dA=2πrdr
Thus, the flux becomes:
ΦE=∫RR/2E⋅2πrdr
Substituting E=Qϵ0πR2 into the flux equation gives:
ΦE=∫RR/2Qϵ0πR2⋅2πrdr
This simplifies to:
ΦE=Qϵ0R2∫RR/2rdr
The integral ∫RR/2rdr evaluates to:
(r22)RR/2=R22−(R/2)22=R22−R28=4R28−R28=3R28
The displacement current ID in the region between R/2 and R can now be expressed as:
ID=ϵ0dΦEdt
Since ΦE depends on Q, and Q is changing with time as the capacitor charges, we can relate ID to the conduction current I.
By Kirchhoff's current law, the conduction current I entering the capacitor must equal the displacement current ID flowing through the region. Since we are considering only the area between R/2 and R, we find that:
ID=34I
Thus, the displacement current flowing in the region between the plates enclosed between the radii R/2 and R is:
3I4
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - COMPETITION FILE - OBJECTIVE TYPE QUESTIONS ( MULTIPLE CHOICE QUESTIONS )(B )40videos
ELECTROMAGNETIC WAVES
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - COMPETITION FILE - OBJECTIVE TYPE QUESTIONS ( MULTIPLE CHOICE QUESTIONS )(C )5videos
ELECTROMAGNETIC WAVES
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - REVISION EXERCISES ( NUMERICAL PROBLEMS )8videos
ELECTROMAGNETIC WAVES
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION14videos
ELECTROMAGNETIC INDUCTION
- BOOK - MODERN PUBLICATIONCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - Chapter Practice Test9videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
Similar Questions
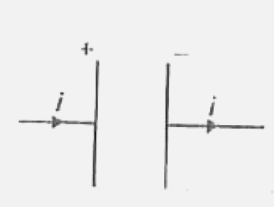
A parallel plate capacitor with circular plates of radius R is being charged as shown. At the instant shown, the displacement current in the region between the plates enclosed between R2 and R is given by
 View Solution
View SolutionEach plate of a parallel plate capacitor has a charge q on it. The capacitor is now connected to a battery. Now,
View Solution
Knowledge Check
- Question 1 - Select One
During charging of capacitor, IC. is the conduction current flowing in the wires connecting capacitor to the battery and Id is the Displacement current flowing between the plates of the capacitor.
AIc>IdBIc<IdCIc=IdDNo relation exists between ic and Id - Question 2 - Select One
A parallel plate capacitor is charged. If the plates are pulled apart
Athe potential difference increasesBthe capacitance increasesCthe total charge increasesDthe charge and the potential difference remain the same - Question 3 - Select One
A parallel plate capacitor having a plate separation of 2mm is charged by connecting it to a 300V supply. The energy density is
A0.01J/m3B0.1J/m3C1.0J/m3D10J/m3
A parallel plate air capacitor is made using two square plates each of side 0.2 m, spaced 1 cm apart. It is connected to a 50V battery.
What is the charge on each plate ?View SolutionWhen a parallel plate capacitor is connected across a d.c. battery. Explain briefly how the capacitor gets charged .
View SolutionA capacitor of capacitance C is connected to a battery of emf V through a resistance R with a switch connected in series .Switch is turned on at t=0 . Find the displacement current flowing between the plates of capacitor as a function of time .
View SolutionA parallel plate capacitor 20 μF is being charged by a voltage source whose potential is changing at the rate of 3 V/s. The conduction current through the connecting wires, and the displacement current through the plates of the capacitor, would be, respectively:
View SolutionA parallel plate capacitor of capacitance 20 μF is being charged by a voltage source whose potential is changing at the rate of 3 V/s. The conduction current through the connecting wires, and the displacement current through the plates of the capacitor, would be, respectively:
View Solution

