Perfectly reflecting mirror of mass M mounted on a spring consitute a spring mass system of angular frequency Ω such that 4πMΩh=1024m−2 where h is plank constant. N photons of wavelength λ=8π×10−6m strikes the mirror simultaneously at normal incidence such that the mirror gets displaced by 1 μm. if the value of N is x×1012, then find value of x.
_PHY_E01_012_Q01.png)
The correct Answer is:[1]
Let momentum of one photon is p. It is given that mirror is perfectly reflecting, hence it gets reflected with the same momentum p along opposite direction. Let v be the velocity gained by mirror in the same direction as that of the incident photon. According to conservation of linear momentum we can write the following equation for N photons.
Np=−Np+mv
⇒mv=2Np ...(i)
Let A(A=1) be the amplitude of oscillation for the mirror and Ω be the angular frequency of the mirror then we should know that v=AΩ And further linear momentum p of the photon can be written as p=hλ .
So equation (i) can be written as follows:
⇒mAΩ=2N(h/λ)
⇒N=mΩh×λA2 ...(ii)
Further it is given that mΩh=10244πandλ=8π×10−6,
Substituting in equation (ii),
N=10244π×8π×10−6×10−62
⇒N=1×112
On comparing we get x = 1.
Topper's Solved these Questions
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - CHAPTER PRACTICE TEST
15 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - COMPETITION FILE (OBJECTIVE TYPE QUESTIONS (MATRIX MATCH TYPE QUESTIONS))
2 Videos
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - Chapter Practice Test
15 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - MODERN PUBLICATIONCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - Chapter Practice Test
15 Videos
Similar Questions
Photoelectric work function for a surface is 2.4 eV. Light of wavelength 6.8×10−7m shines on the surface. Find the frequency of incident light and also the threshold frequency. Will there be photoelectric emission or not ?
View SolutionIn the arrangement shown in the diagram, pulleys are small and springs are ideal. k1=k2=k3=k4=10Nm−1 are force constants of the springs and mass m=10kg. If the time period of small vertical oscillations of the block of mass m is given by 2πx seconds, then find the value of x.
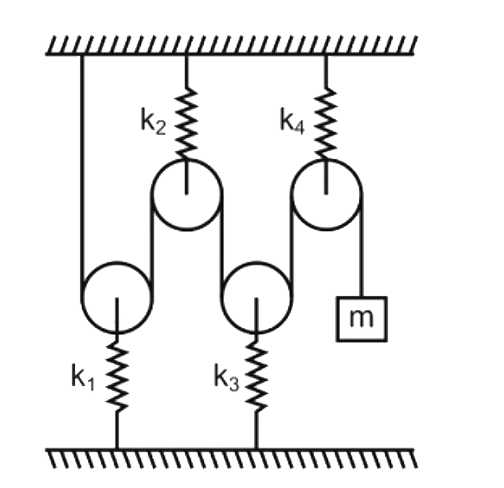
View Solution
Knowledge Check
The frequency f of vibrations of a mass m suspended from a spring of spring constant k is given by f=Cmxky , where C is a dimensionnless constant. The values of xandy are, respectively,
Ax=12,y=12Bx=−12,y=−12Cx=−12,y=12Dx=12,y=12The angular frequency of the damped oscillator is given by ω=√km−r24m2 ,where k is the spring constant, m is the mass of the oscillator and r is the damping constant. If the ratio r2/(mk) is 8% ,the change in the time period compared to the undamped oscillator
Aincreases by 1%Bincreases by 8%Cdecreased by 1%Ddecreasea block of mass 0.1 kg attached to a spring of spring constant400Nm pulled horizontally from x=0 tox1=10mm. Find the work done by the spring force
A2⋅10−2JB2⋅10−6JC3⋅10−6JD4⋅10−2J
Monochromatic light of wavelength 3000A is incident normally on a surface of area 4cm2. If the intensity of the light is 5×10−2W/m2, determine the rate at which photons strike the surface
View SolutionA block of mass m moving at a speed v compresses a spring through a distance x before its speed is halved. The spring constant of the spring is 6mv2nx2. Find value of n.
View SolutionA πσ meson at rest decays into two photons of equal energy. If the wavelength (in m) of the photons is 1.8×10−n then find n/2 ( The mass of the πo135MeV/c)
View Solution" of intensity "1.5kW/m^(2)" is incident normally on a perfectly absorbing surface.The pressure exerted by the light on the surface is "n times10^(-6)Pa" .Value of "n" is "
View Solutionlf the conductivity of mercury at 0°C is 1.07×106Sm−1 and the resistance of a cell containing mercury is 0.243Ω, then the cell constant of the cell is x×104m−1. The value of x is _________ (Nearest integer)
View Solution
