An instantaneous displacement of a simple harmonic oscillator is x=Acos(ωt+π/4). Its speed will be maximum at time : Solution in Kannada
π/4ω
π/ω
π/2ω
2π/ω.
The correct Answer is:A
v=dxdt=−Aωsin(ωt+π/4)
For velocity to be maximum
ωt+π/4=π/2 ⇒ t=π4ω
Correct choice is (a).
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONSEXERCISE - MCQ (LEVEL-II)62videos
OSCILLATIONS
- BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONSEXERCISE - MCQ (LEVEL-III) Questions From AIEEE/JEE Examination10videos
OSCILLATIONS
- BOOK - MODERN PUBLICATIONCHAPTER - MOCK TEST-3EXERCISE - MCQs49videos
MOCK TEST-3
- BOOK - MODERN PUBLICATIONCHAPTER - PROPERTIES OF MATTEREXERCISE - RECENT COMPETITIVE QUESTIONS17videos
PROPERTIES OF MATTER
Similar Questions
A pendulum suspended from ceiling of a train has a time period T, when the train is at rest. When the train is accelerating with uniform acceleration 'a', the period of oscillation will
03:13View SolutionTwo particles are executing simple harmonic motion of the same amplitude A and frequency ω along the x-axis. Their mean position is separated by distance X0(X0>A). If the maximum separation between them is (X0+A), the phase difference between their motion is
02:09View SolutionA man standing in a lift holds a spring balance with a load of 5 kg suspended from it. What would be the reading of the balance when the lift is descending with an acceleration of 4ms−2 ?
01:57View SolutionWhich of the following substances has the highest elasticity ?
04:40View SolutionThe displacement of a particle executing simple harmonic motion is given by y=A0+Asinωt+Bcosωt. Then the amplitude of its oscillation is given by
01:50View SolutionThe instantaneous displacement x of a particle executing simple harmonic motion is given by x=a_1sinomegat+a_2cos(omegat+(pi)/(6)) . The amplitude A of oscillation is given by
02:25View SolutionThe instantaneous displacement of a simple pendulum oscillator is given by x= A cos (omegat+ pi / 4 ) Its speed will be maximum at time
02:26View SolutionThe displacement equation of a simple harmonic oscillator is given by y=A sin omegat-Bcos omegat The amplitude of the oscillator will be
01:57View SolutionThe figure gives the displacement versus time graph of a simple harmonic oscillator. The position with maximum speed directed down wards is at
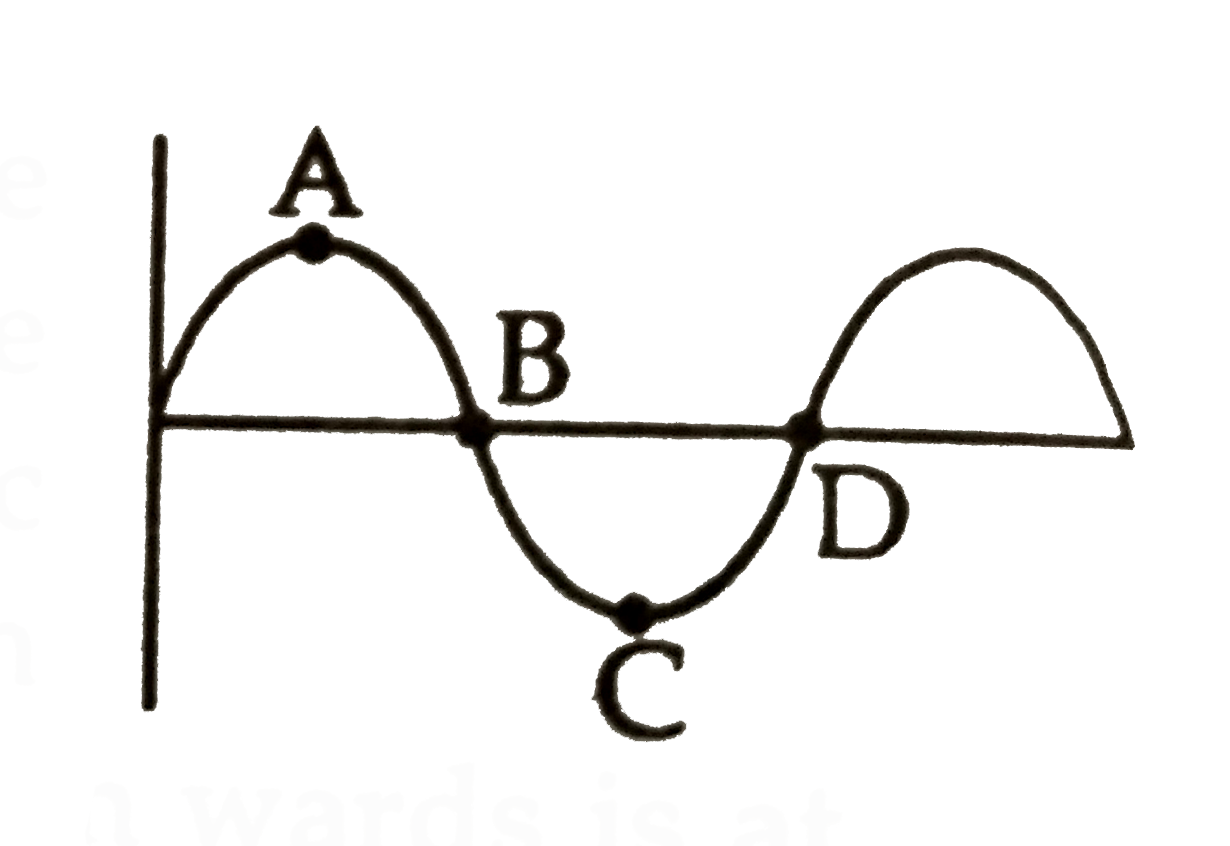 05:16View Solution
05:16View SolutionA simple harmonic oscillator has its displacement varying as x = 15 sin (2pit+pi//4) metre. Then its initial displacement is
04:37View SolutionThe displacement of a simple harmonic oscillator is given by x = 4 cos (2pit+pi//4)m . Then velocity of the oscillator at t = 2 s is
02:47View SolutionThe instantaneous displacement of a simple pendulum oscillator is given by x=Acos(ωt+π4)Its speed will be maximum at time
View Solutionएक सरल लोलक दोलित्र का तात्क्षणिक विस्थापन x=Acos(ωt+π4) द्वारा दिया जाता है। किस समय पर इसकी चाल अधिकतम होगी
View SolutionThe displacement of a particle executing simple harmonic motion is given by y=A0+Asinωt+Bcosωt. Then the amplitude of its oscillation is given by
03:07View SolutionThe instantaneous displacement x of a particle executing simple harmonic motion is given by x=a1sinωt+a2cos(ωt+π6). The amplitude A of oscillation is given by
02:01View Solution

