If the length of the perpendicular from the vertices of a triangle A,B,C on the opposite sides are P1,P2,P3 then 1P1+1P2+1P3=
Step by step video & image solution for If the length of the perpendicular from the vertices of a triangle A,B,C on the opposite sides are P_(1),P_(2),P_(3) then (1)/(P_(1))+(1)/(P_(2))+(1)/(P_(3))= by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Similar Questions
If the lengths of the perpendiculars from the vertices of a triangle ABC on the opposite sides are p1,p2,p3 then prove that 1p1+1p2+1p3=1r=1r1+1r2+1r3.
View SolutionIf p1,p2 and p3 and are respectively the perpendiculars from the vertices of a triangle to the opposite sides, then 1p1+1p2−1p3=.
View Solution
Knowledge Check
- Question 1 - Select One or More
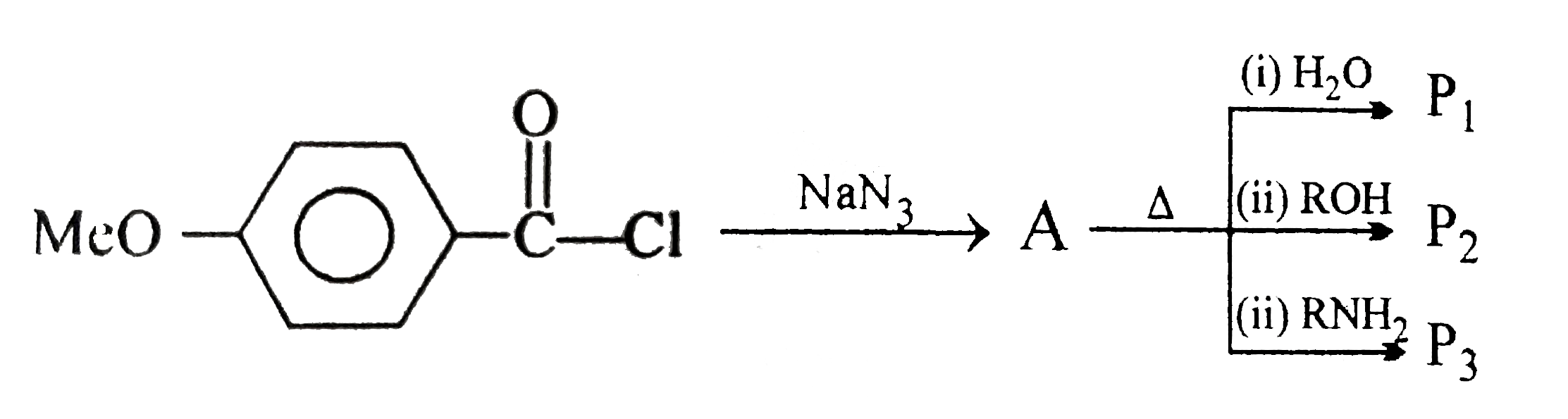
P1,P2,P3,are:A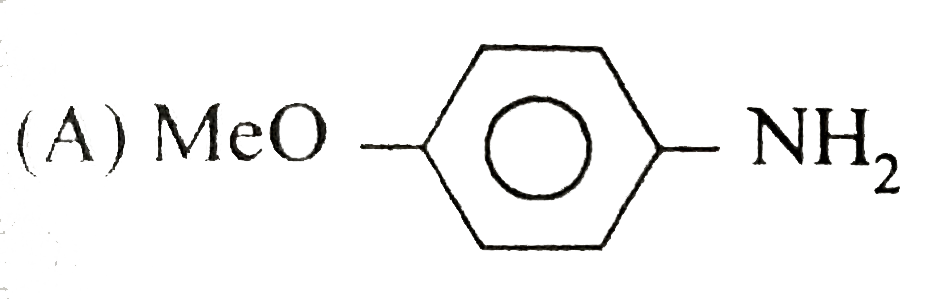 B
B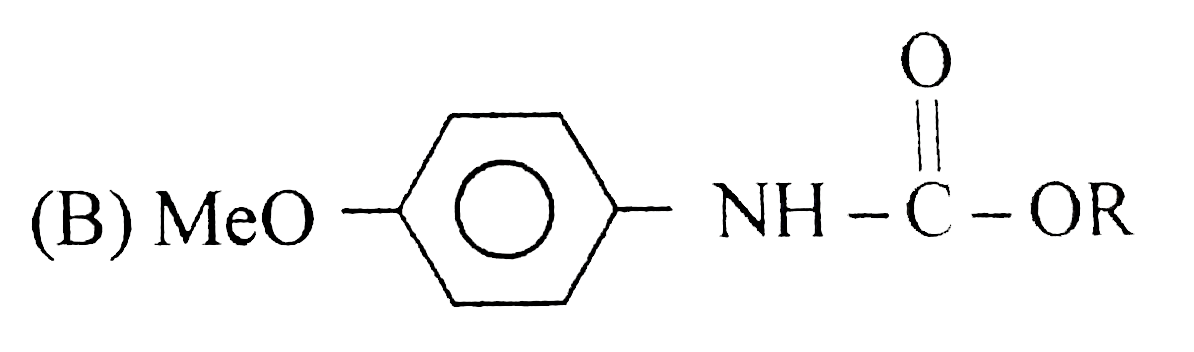 C
C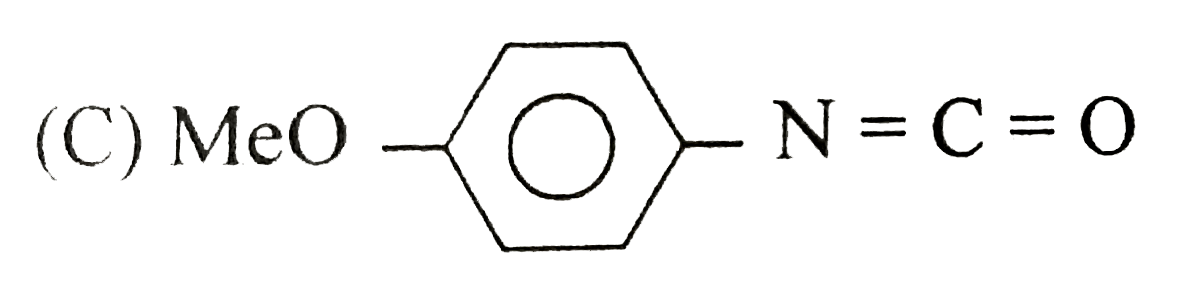 D
D
- Question 2 - Select One
If p1,p2,p3 are respectively the perpendiculars from the vertices of a triangle to the opposite sides , then cosAp1+cosBp2+cosCp3 is equal to
A1/rB1/RC1/!Dnone of these - Question 3 - Select One
The length of the perpendicular drawn from any point in the interior of an equilateral triangle to the respective sides are p1,p2 and p3. The length of each side of the triangle is
A(a) 2√3(p1+p2+p3)B(b) 13(p1+p2+p3)C(c) 1√3(p1+p2+p3)D(d) 4√3(p1+p2+p3)
If p1,p2 and p3 and are respectively the perpendiculars from the vertices of a triangle to the opposite sides, prove that : 1p1+1p2+1p3=1r.
View SolutionIf p2,p2,p3 are the perpendiculars from the vertices of a triangle to the opposite sides, then prove that
p1p2p3=a2b2c28R3View SolutionIf p1,p2,p3 are respectively the perpendicular from the vertices of a triangle to the opposite sides , then find the value of p1p2p3
View SolutionOne diagonal of a square is the portion of the line x/97+y/79=1 intercepted between the axes p1,p2 are the length of the perpendiculars from the vertices of the other diagonal on the axis of y. If p2<p1 then p1/p2 is equal to
View SolutionIf p1,p2,p3 are respectively the perpendicular from the vertices of a triangle to the opposite sides, then find the value of p1p2p3.
View Solution
