Find the values of x for which the following functions are identical.
(i) $f (x) = x and g (x) = \frac{1}{1 / x}$
(ii) $f (x) = \frac{\sqrt{9 - x^{2}}}{\sqrt{x - 2}} and g (x) = \sqrt{\frac{9 - x^{2}}{x - 2}}$

Video Solution

Text Solution

Verified by Experts

(i) $f (x) = x$ is defined for all x.
but $g (x) = \frac{1}{1 / x} = x$ is not defined for $x = 0$ as $1 / x$ is not defined at $x = 0$ .
Hence both the functions are identical for $x \in R - {0} .$
(ii) $f (x) = \frac{\sqrt{9 - x^{2}}}{\sqrt{x - 2}}$ is defined if $9 - x^{2} \geq 0 and x - 2 > 0$
$\Rightarrow x \in [- 3, 3] and x > 2 \Rightarrow x \in (2, 3]$
$g (x) = \sqrt{\frac{9 - x^{2}}{x - 2}}$ is defined if $\frac{9 - x^{2}}{x - 2} \geq 0$
$\Rightarrow \frac{x^{2} - 9}{x - 2} \leq 0$

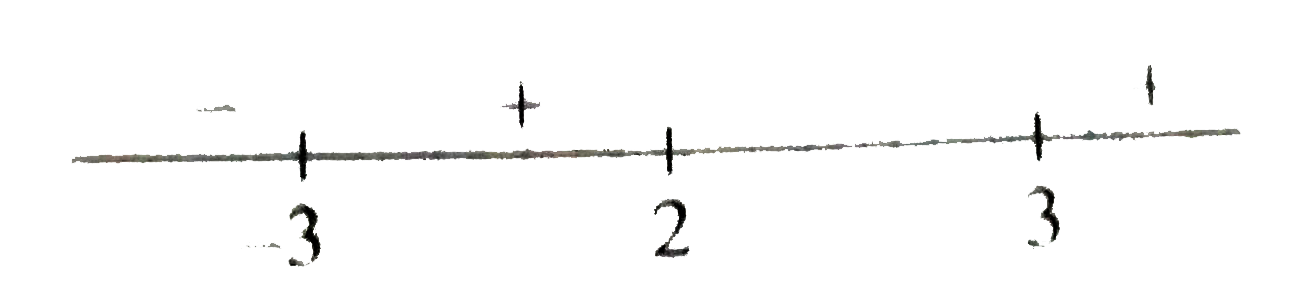
From the sign scheme $x \in (- \infty, - 3] \cup (2, 3]$
Hence, f(x) and g(x) are identical if $x \in (2, 3]$

Updated on:21/07/2023