Solve (a) tanx<2 cosx≤−12
Video Solution
Text Solution
The correct Answer is:(a) ∪n∈Z(nπ−π2,nπ+tan−12)
(b) ∪n∈Z[2nπ+2π3,2nπ+4π3]
(a) We know that tanx is periodic with period π. So, check the solution in the interval (−π2,π2).
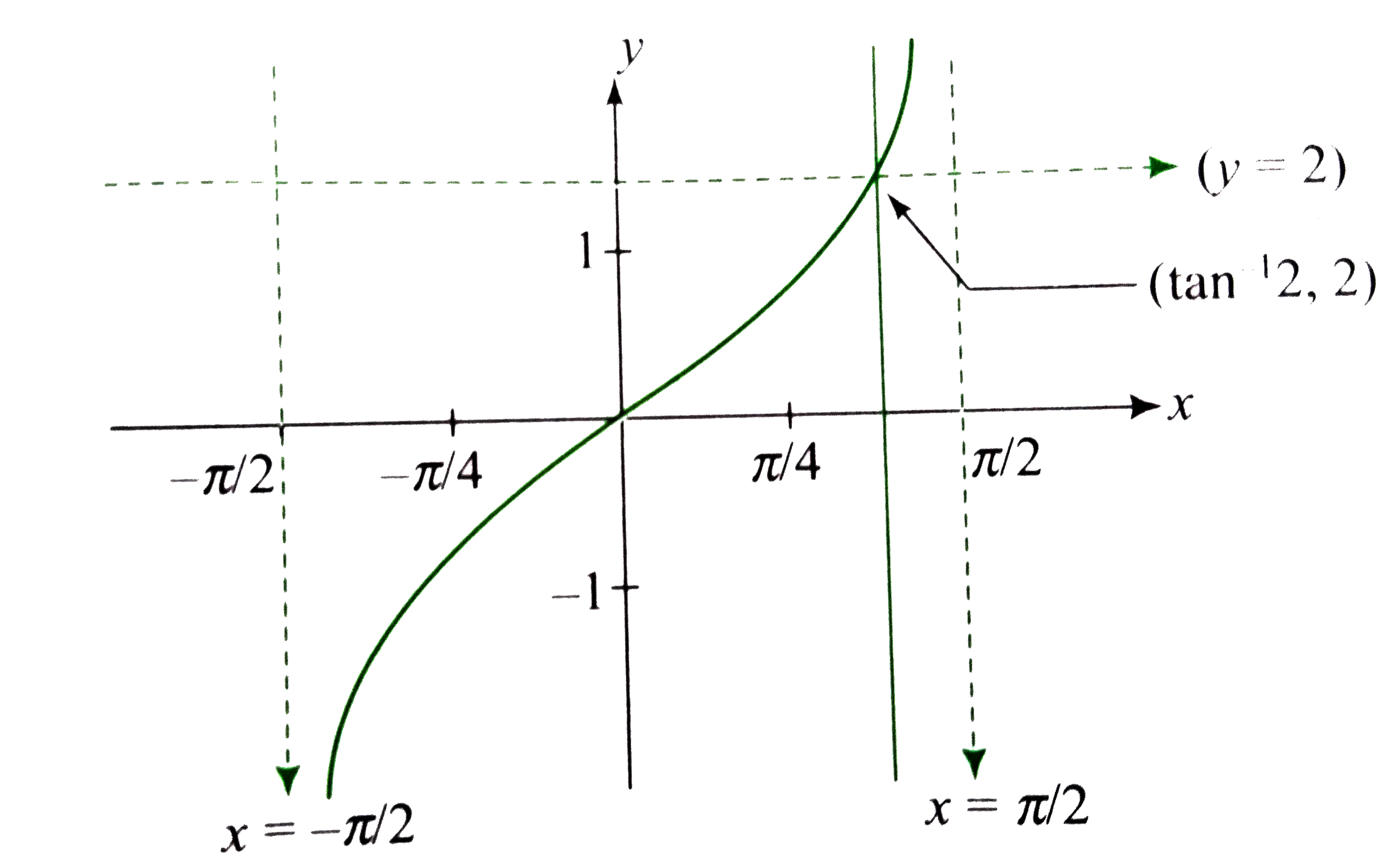
It is clear from the figure that tanx<2 when
−π2<x<tan−12.
Therefore, general solution is
∪n∈Z(nπ−π2,nπ+tan−12)
(b) cosx is periodic with period 2π.
So, check the solution in [0,2π].
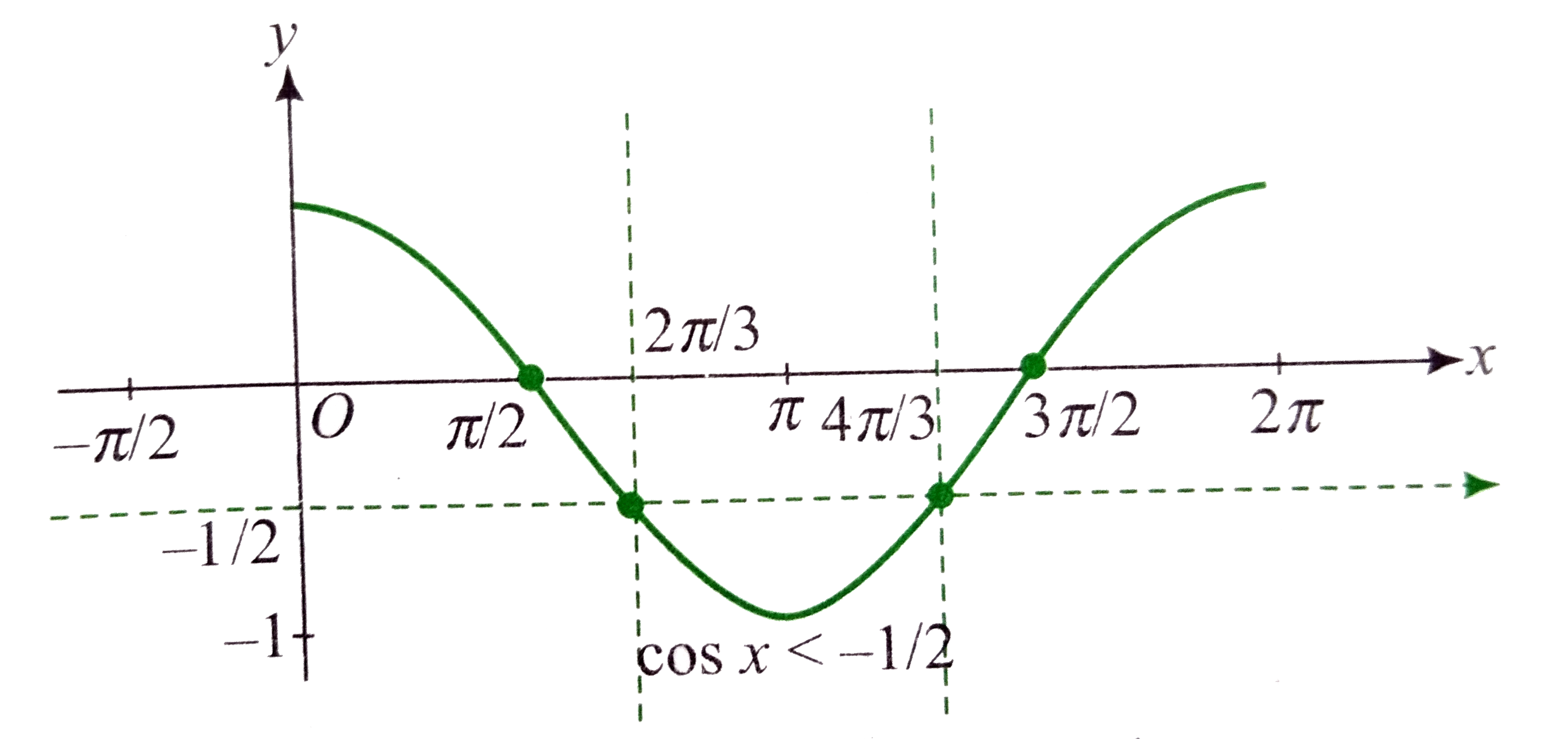
It is clear from the figure that cosx≤−12 when
2π3≤x≤4π3.
On generalizing the above solution, we get
x∈∪n∈Z[2nπ+2π3,2nπ+4π3]
|
Topper's Solved these Questions
- RELATIONS AND FUNCTIONSBOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Exercise 1.7
5 Videos
- RELATIONS AND FUNCTIONSBOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Exercise 1.8
9 Videos
- RELATIONS AND FUNCTIONSBOOK - CENGAGECHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Exercise 1.5
5 Videos
- Quadratic Equations, Inequalities, Modulus and LogarithmsBOOK - CENGAGECHAPTER - Quadratic Equations, Inequalities, Modulus and LogarithmsEXERCISE - Question Bank
31 Videos
- SCALER TRIPLE PRODUCTS BOOK - CENGAGECHAPTER - SCALER TRIPLE PRODUCTS EXERCISE - DPP 2.3
11 Videos
Similar Questions
Solve :(tanx−1√3)(cosx−12)≤0.
View Solutionx4tanx
View SolutionSolve (i) limx→0+[tanxx]
(ii) limx→0−[tanxx]
(where [.] denotes greatest integer function)
View SolutionSolve sec2xtanydx+sec2ytanxdy=0
View SolutionSolve the equation, (1−tanx)(1+sin2x)=1+tanx
View SolutionSolve the equation 1+tanx1−tanx=1+sin2xcos2x.
View SolutionSolve the equation (cosx−sinx)(2tanx+2)=0
View SolutionSolve the equation (sinx+cosx)=√2evaluatetanx+cotx.
View SolutionSolve the equation sin2x(tanx+1)=3sinx(cosx−sinx)+3.
View SolutionEvaluate ∫tanxsecx+tanxdx
View Solution
