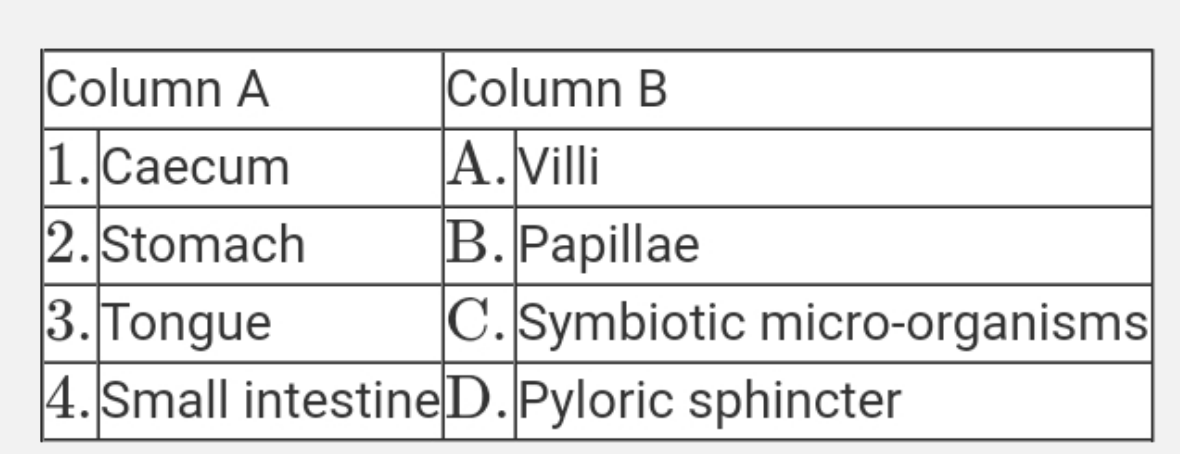
Match the column
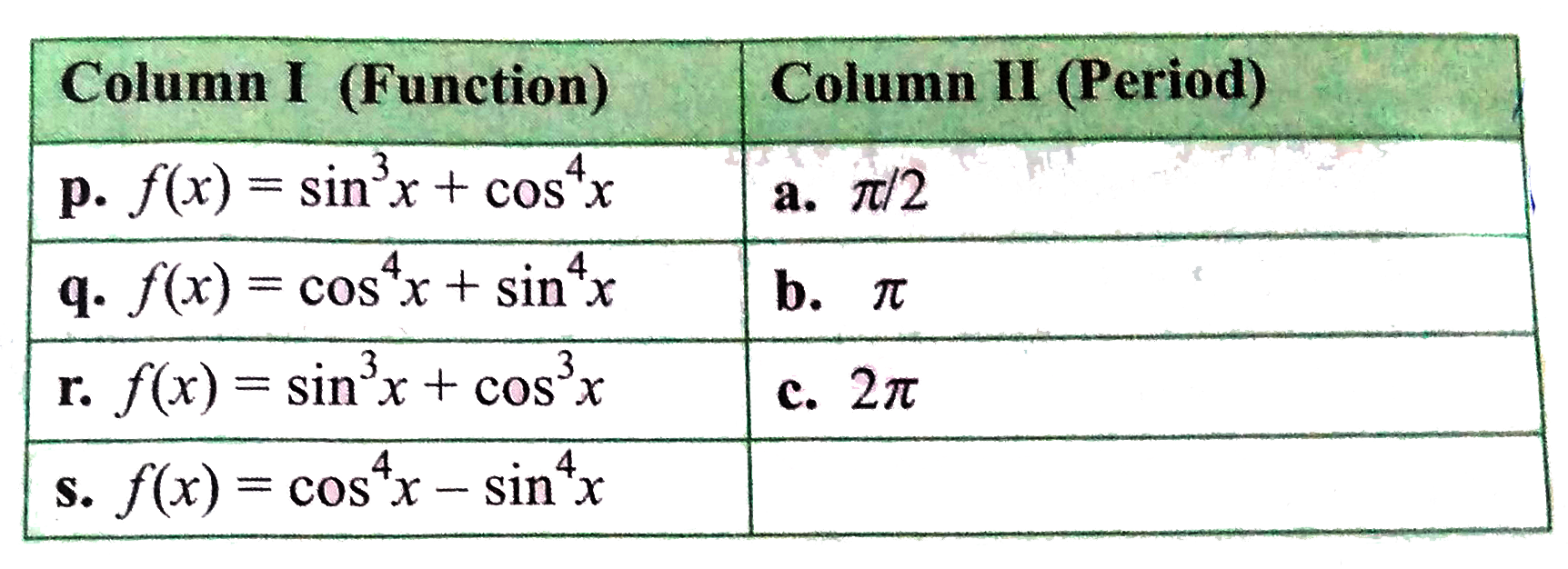
Text Solution
The correct Answer is:p→c;q→a;r→c;s→b
(p) f(x)=sin3x+cos4x,
sin3x has period 2π and cos4x has period π, and L.C.M. of π and 2π is 2π. Hence, period is 2π.
(q) f(x)=sin4x+cos4x,
Both sin4x and cos4x have the same period π, and L.C.M. of π and π is π.
But f(x+π/2)=f(x). Then period is π/2.
(r) Both sin3x and cos3x have the same period 2π, and L.C.M. of 2π and 2π is 2π.
Hence, period is 2π,[(f(x+π)≠f(x)].
(s) f(x)=cos4x−sin4x
Both sin4x and cos4x have the same period π, and L.C.M. of π and π is π.
Hence, period is π,[f(x+π/2)≠f(x)].
|
Topper's Solved these Questions
- RELATIONS AND FUNCTIONSBook:CENGAGEChapter:RELATIONS AND FUNCTIONSExercise:Exercise 1.12
Explore 9 Videos
- RELATIONS AND FUNCTIONSBook:CENGAGEChapter:RELATIONS AND FUNCTIONSExercise:Exercise 1.13
Explore 7 Videos
- RELATIONS AND FUNCTIONSBook:CENGAGEChapter:RELATIONS AND FUNCTIONSExercise:Exercise 1.10
Explore 6 Videos
- Quadratic Equations, Inequalities, Modulus and LogarithmsBook:CENGAGEChapter:Quadratic Equations, Inequalities, Modulus and LogarithmsExercise:Question Bank
Explore 31 Videos
- SCALER TRIPLE PRODUCTS Book:CENGAGEChapter:SCALER TRIPLE PRODUCTS Exercise:DPP 2.3
Explore 11 Videos