The number of triangles which are obtuse and which have the points (8,9),(8,16) and (20,25) as the feet of perpendiculars drawn from the vertices on the opposite sides is
0
1
2
3
The correct Answer is:D
There exist exactly four triangles ABC,APC,APB and BPC satisfying the given conditions of which three triangles will be obtuse angle.
Topper's Solved these Questions
- COORDINATE SYSTEMBOOK - CENGAGECHAPTER - COORDINATE SYSTEMEXERCISE - Comprehension Type
4 Videos
- COORDINATE SYSTEMBOOK - CENGAGECHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - CENGAGECHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Question Bank
22 Videos
- COORDINATE SYSYEM BOOK - CENGAGECHAPTER - COORDINATE SYSYEM EXERCISE - JEE Main Previous Year
6 Videos
Similar Questions
Consider the following statements :
1. The point of intersection of the perpendicular bisectors of the sides of a triangle may lie outside the triangle.
2. The point of intersection of the perpendiculars drawn from the vertices to the opposite sides of a triangle may lie on two sides.
Which of the above statements is/are correct ?
View SolutionThe coordinates of the feet of the perpendiculars from the vertices of a triangle on the opposite sides are (20, 25), (8, 16) and (8, 9). If the orthocentre of the triangle is (h, k) then k/h is equal to.
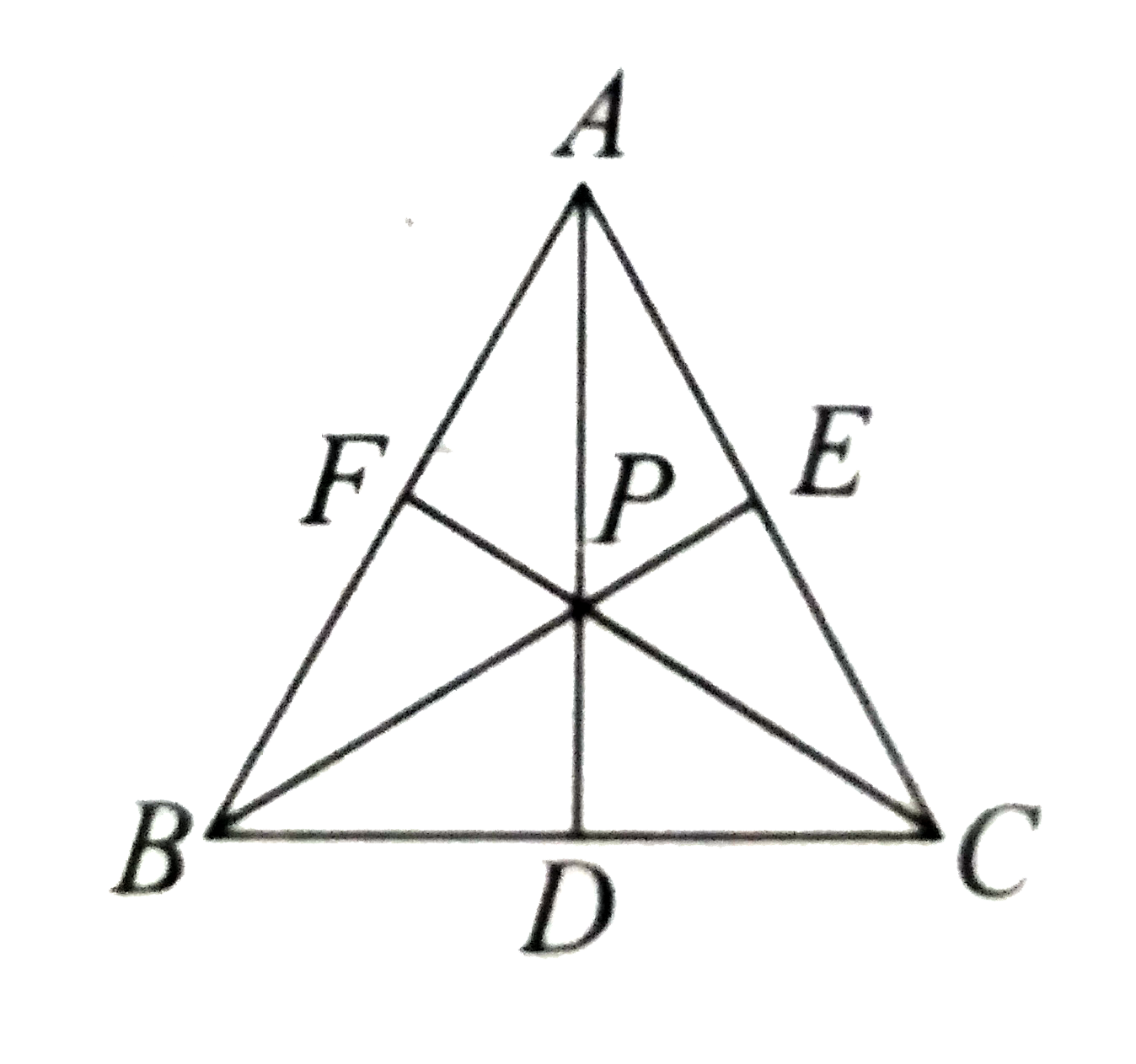
Note : The triangle joining the feel of the perpendiculars from the vertices of a trangle on the opposite sides is called the PEDAL TRIANGLE of the triangle.
View Solution
Knowledge Check
The vertices of a triangle are (2,1),(5,2)and(4,4) The lengths of the perpendicular from these vertices on the opposite sides are
A7√5,7√13,7√6B7√6,7√8,7√10C7√5,7√8,7√15D7√5,7√13,7√10The perpendiculars drawn from the vertices to the opposite sides of a triangle, meet at the point whose name is
AincentreBcircumcentre barCcentroidDorthocentreThe point in the plane of a triangle which is at equal perpendicular distance from the sides of the triangle is :
AcentroidBincentreCcircumcentreDorthocentre
Prove that the perpendicular let fall from the vertices of a triangle to the opposite sides are concurrent.
View SolutionProve that the perpendicular let fall from the vertices of a triangle to the opposite sides are concurrent.
View SolutionNumber of tangent(s) which can be drawn to the ellipse 16x2+25y2=400 such that sum of perpendicular distance from the foci to the tangent is 8.
View SolutionProve that the perpendiculars drawn from the vertices of equal angles of an isosceles triangle to the opposite sides are equal.
View SolutionIf ΔABC is an isosceles triangle with AB = AC. Prove that the perpendiculars from the vertices B and C to their opposite sides are equal.
View Solution
