Two vertices of a triangle are (1, 3) and (4, 7). The orthocentre lies on the line $x + y = 3$ . The locus of the third vertex is

A

$x^{2} - 2 x y + 2 y^{2} - 3 x - 4 y + 36 = 0$

B

$2 x^{2} - 4 x y + 3 y^{2} - 4 x - y + 42 = 0$

C

$3 x^{2} + x y - 4 y^{2} - 2 x + 24 y - 40 = 0$

D

$x^{2} - 4 x y + 3 y^{2} - 2 x - y + 40 = 0$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

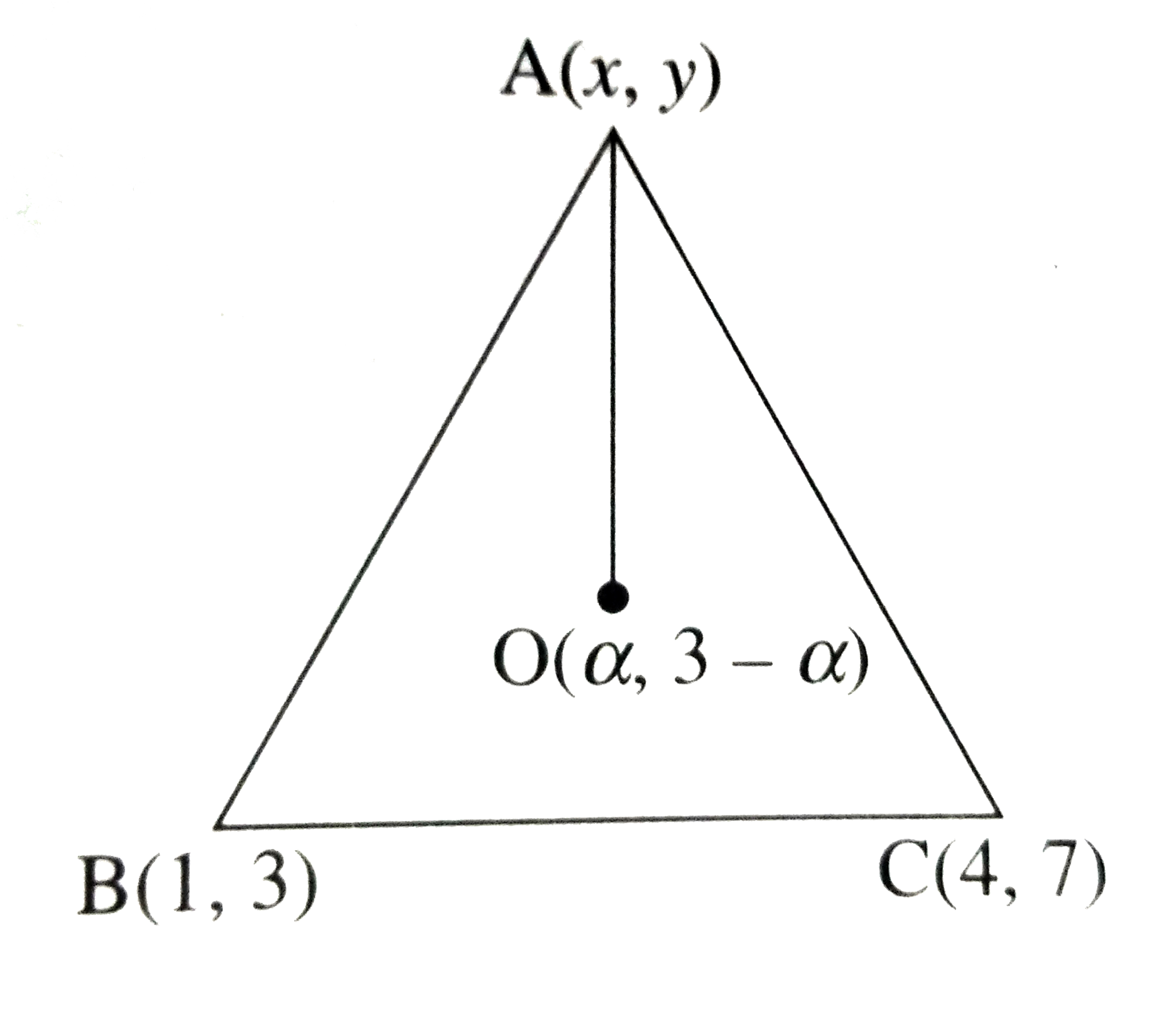
Orthocenter lies on the line $x + y = 2$
Let orthocenter be $O (α, 3 - α)$
$B O ⊥ A C$
$\Rightarrow \frac{y - 7}{x - 4} \frac{α}{1 - α} = - 1$
$\Rightarrow \frac{α - 1}{α} = \frac{y - 7}{x - 4}$
$\Rightarrow 1 - \frac{1}{α} = \frac{y - 7}{x - 4}$
$\Rightarrow \frac{1}{α} = 1 - \frac{y - 7}{x - 4}$
$\Rightarrow α = \frac{x - 4}{x - y + 3}$
Also, $A O ⊥ B C$
$\Rightarrow \frac{y - 3 + α}{x - α} \times \frac{4}{3} = - 1$
$\Rightarrow 4 y = 12 + 4 α = - 3 x + 3 α$
$\Rightarrow α = - 3 x - 4 y + 12$
$∴$ The locus is $\frac{x - 4}{x - y + 3} = - 3 x - 4 y + 12$

|

Updated on:21/07/2023

Class 12 MATHS STRAIGHT LINES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Two vertices of a triangle ABC are B(5,-1) and C(-2,3) .If the orthocentre of the triangle is the origin , find the thrid vertex.
A $(\frac{7}{2} . \frac{13}{2})$
B $(\frac{3}{2}, \frac{11}{2})$
C(-4, -7)
Dnone of these
Question 2 - Select One
If two vertices of a triangle are (5, -1) and (-2, 3) and if its orthocentre lies at the origin, then the coordinates of third vertex are
A(4, 7)
B(-4, -7)
C(2, -3)
D(5, -1)
Question 3 - Select One
Two vertices of a triangle ABC are B(5,-1) and C(-2,3) .If the orthocentre of the triangle is the origin , find the thrid vertex.
Aa. $(\frac{7}{2}, \frac{13}{2})$
B $(\frac{3}{2}, \frac{11}{2})$
C $(- 4, - 7)$
Dnone of these

CENGAGE-COORDINATE SYSTEM-Multiple Correct Answers Type

Home
Ask Doubt
Profile